Skip to main content
Springer Nature Link
Log in

Amplification of squeezing and anti-bunching in the output beams from a degenerate parametric amplifier: linear and nonlinear coherent state approaches

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

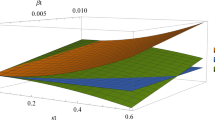
Nonlinear optical phenomena have noticeable importance in quantum computation and quantum information processing. The optical nonlinearity leads to the generation of new frequency components possessing nonclassical properties. In this paper, we have investigated nonclassical properties of output beams from a degenerate parametric amplifier (DPA) based on both linear and nonlinear coherent state approaches. Considering the rotating wave approximation, solutions of the Heisenberg equations of motion associated with the standard coherent and nonlinear coherent radiation fields are compared. Nonclassical properties including the first- and second-order squeezing, anti-bunching and photon statistics are investigated for the signal mode starting with different input field states. The calculations based on both approaches confirm the existence of the first-order squeezing in position quadrature (\(\hat{x}_1(\hat{X}_1)\) for linear(nonlinear) approach) of the field with no squeezing in momentum quadrature, while the second-order squeezing occurs in the momentum quadrature of the field (\(\hat{x}_2(\hat{X}_2)\) for linear(nonlinear) approach) with no squeezing in position quadrature. In addition, photon statistics has been studied wherein anti-bunching has been observed using both approaches. The comparison of the obtained results from the two approaches confirms that in the nonlinear approach the nonclassical properties of the output beams are more amplified.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

No datasets were generated or analyzed during the current study.

References

  1. Albarelli, F., Ferraro, A., Paternostro, M., Paris, M.G.A.: Nonlinearity as a resource for nonclassicality in anharmonic systems. Phys. Rev. A 93(3), 032112 (2016)

    ADS  Google Scholar 

  2. Xue-xiang, Xu., Yuan, Hong-chun: Conditional generation of multiphoton-subtracted squeezed vacuum states: loss consideration and operator description. Quantum Inf. Process. 19, 1–15 (2020)

    Google Scholar 

  3. Franken, P.A., Hill, A.E., Peters, C.W., Weinreich, G.: Generation of optical harmonics. Phys. Rev. Lett. 7(4), 118 (1961)

    ADS  Google Scholar 

  4. Boyd, R.W.: Nonlinear Optics. Academic Press, Boca Raton (2020)

    Google Scholar 

  5. Ralph, T.C., Gilchrist, A., Milburn, G.J., Munro, W.J., Glancy, S.: Quantum computation with optical coherent states. Phys. Rev. A 68(4), 042319 (2003)

    ADS  Google Scholar 

  6. Bartlett, S.D., Sanders, B.C.: Universal continuous-variable quantum computation: requirement of optical nonlinearity for photon counting. Phys. Rev. A 65(4), 042304 (2002)

    ADS  Google Scholar 

  7. Vahlbruch, H., Chelkowski, S., Danzmann, K., Schnabel, R.: Quantum engineering of squeezed states for quantum communication and metrology. New J. Phys. 9(10), 371 (2007)

    ADS  Google Scholar 

  8. Marino, A.M., Stroud, C.R.: Deterministic secure communications using two-mode squeezed states. Phys. Rev. A 74(2), 022315 (2006)

    ADS  Google Scholar 

  9. Nielsen, M.A., Chuang, I.L.: Quantum computation and quantum information. Cambridge University Press, Cambridge (2010)

  10. Cardoso, F.R., Rossatto, D.Z., Fernandes, G.P.L.M., Higgins, G., Villas-Boas, C.J.: Superposition of two-mode squeezed states for quantum information processing and quantum sensing. Phys. Rev. A 103(6), 062405 (2021)

    ADS  MathSciNet  Google Scholar 

  11. Hillery, M.: Quantum cryptography with squeezed states. Phys. Rev. A 61(2), 022309 (2000)

    ADS  MathSciNet  Google Scholar 

  12. Huang, H., Zhu, S.Y., Zubairy, M.S.: Noise-free amplification of squeezed light via atomic coherence. Phys. Rev. A 52(5), 4155 (1995)

    ADS  Google Scholar 

  13. Mehmet, M., Ast, S., Eberle, T., Steinlechner, S., Vahlbruch, H., Schnabel, R.: Squeezed light at 1550 nm with a quantum noise reduction of 12.3 db. Opt. Express 19(25), 25763–25772 (2011)

    ADS  Google Scholar 

  14. Weyrauch, M., Voronov, V.G.: Reduction of quantum noise in optical interferometers using squeezed light. Opt. Spectrosc. 111, 709–712 (2011)

    ADS  Google Scholar 

  15. Glauber, R.J.: The quantum theory of optical coherence. Phys. Rev. 130(6), 2529 (1963)

    ADS  MathSciNet  Google Scholar 

  16. de Matos Filho, R.L., Vogel, W.: Nonlinear coherent states. Phys. Rev. A 54(5), 4560 (1996)

    ADS  Google Scholar 

  17. Man’ko, V.I., Marmo, G., Sudarshan, E.C.G., Zaccaria, F.: f-oscillators and nonlinear coherent states. Phys. Scr. 55(5), 528 (1997)

    ADS  Google Scholar 

  18. Roknizadeh, R., Tavassoly, M.K.: The construction of some important classes of generalized coherent states: the nonlinear coherent states method. J. Phys. A: Math. Gen. 37(33), 8111 (2004)

    ADS  MathSciNet  Google Scholar 

  19. Safaeian, O., Tavassoly, M.K.: Deformed photon-added nonlinear coherent states and their non-classical properties. J. Phys. A: Math. Theor. 44(22), 225301 (2011)

    ADS  MathSciNet  Google Scholar 

  20. Karimi, A., Tavassoly, M.K.: Quantum engineering and nonclassical properties of su (1, 1) and su (2) entangled nonlinear coherent states. JOSA B 31(10), 2345–2353 (2014)

    ADS  Google Scholar 

  21. Tavassoly, M.K.: On the non-classicality features of new classes of nonlinear coherent states. Opt. Commun. 283(24), 5081–5091 (2010)

    ADS  Google Scholar 

  22. Roy, B., Roy, P.: New nonlinear coherent states and some of their nonclassical properties. J. Opt. B: Quantum Semiclass. Opt. 2(1), 65 (2000)

    ADS  MathSciNet  Google Scholar 

  23. Belfakir, A., Hassouni, Y.: Bipartite entanglement of generalized Barut–Girardello nonlinear coherent states. Quantum Inf. Process. 20, 1–19 (2021)

    ADS  MathSciNet  Google Scholar 

  24. Michler, P., Kiraz, A., Becher, C., Schoenfeld, W.V., Petroff, P.M., Zhang, L., Hu, E., Imamoglu, A.: A quantum dot single-photon turnstile device. Science 290(5500), 2282–2285 (2000)

    ADS  Google Scholar 

  25. Pisanello, F., Martiradonna, L., Leménager, G., Spinicelli, P., Fiore, A., Manna, L., Hermier, Jean-Pierre., Cingolani, R., Giacobino, E., De Vittorio, M., et al.: Room temperature-dipolelike single photon source with a colloidal dot-in-rod. Appl. Phys. Lett. 96(3), 033101 (2010)

    ADS  Google Scholar 

  26. Kurtsiefer, C., Mayer, S., Zarda, P., Weinfurter, H.: Stable solid-state source of single photons. Phys. Rev. Lett. 85(2), 290 (2000)

    ADS  Google Scholar 

  27. Liew, T.C.H., Savona, V.: Single photons from coupled quantum modes. Phys. Rev. Lett. 104(18), 183601 (2010)

    ADS  Google Scholar 

  28. Amazioug, M., Daoud, M., Singh, S.K., Asjad, M.: Strong photon antibunching effect in a double-cavity optomechanical system with intracavity squeezed light. Quantum Inf. Process. 22(8), 301 (2023)

    ADS  MathSciNet  Google Scholar 

  29. Wen-ju, Gu., Li, Gao-xiang, Yang, Ya.-ping: Generation of squeezed states in a movable mirror via dissipative optomechanical coupling. Phys. Rev. A 88(1), 013835 (2013)

    ADS  Google Scholar 

  30. Zagoskin, A.M., Il’Ichev, E., McCutcheon, M.W., Young, J.F., Nori, F.: Controlled generation of squeezed states of microwave radiation in a superconducting resonant circuit. Phys. Rev. Lett. 101(25), 253602 (2008)

    ADS  Google Scholar 

  31. Turnbull, M.T., Petrov, P.G., Embrey, C.S., Marino, A.M., Boyer, V.: Role of the phase-matching condition in nondegenerate four-wave mixing in hot vapors for the generation of squeezed states of light. Phys. Rev. A 88(3), 033845 (2013)

    ADS  Google Scholar 

  32. Rhoads, J.F., Shaw, S.W.: The impact of nonlinearity on degenerate parametric amplifiers. Appl. Phys. Lett. 96(23), 234101 (2010)

    ADS  Google Scholar 

  33. Milburn, G., Walls, D.F.: Production of squeezed states in a degenerate parametric amplifier. Opt. Commun. 39(6), 401–404 (1981)

    ADS  Google Scholar 

  34. Lamprou, T.H., Liontos, I., Papadakis, N.C., Tzallas, P.: A perspective on high photon flux nonclassical light and applications in nonlinear optics. High Power Laser Sci. Eng. 8, 42 (2020)

  35. Shan, L., Ren, J., Zhang, Q., Liu, Q., Ma, Y., Gong, Q., Gu, Y.: Generation and modulation of non-classical light in a strongly coupled photon-emitter system. Photonics Res. 10(4), 989–998 (2022)

    Google Scholar 

  36. Gonoskov, I., Sondenheimer, R., Hünecke, C., Kartashov, D., Peschel, U., Gräfe, S.: Nonclassical light generation and control from laser-driven semiconductor intraband excitations. Phys. Rev. B 109(12), 125110 (2024)

    ADS  Google Scholar 

  37. Dwyer, S.E., Mansell, G.L., McCuller, L.: Squeezing in gravitational wave detectors. Galaxies 10(2), 46 (2022)

    ADS  Google Scholar 

  38. Bondurant, R.S., Shapiro, J.H.: Squeezed states in phase-sensing interferometers. Phys. Rev. D 30(12), 2548 (1984)

    ADS  Google Scholar 

  39. Abadie, J., Abbott, B.P., Abbott, R., Abbott, T.D., Abernathy, M., et al.: A gravitational wave observatory operating beyond the quantum shot-noise limit. Nat. Phys. 7(12), 962–965 (2011)

    Google Scholar 

  40. Grote, H., Danzmann, K., Dooley, K.L., Schnabel, R., Slutsky, J., Vahlbruch, H.: First long-term application of squeezed states of light in a gravitational-wave observatory. Phys. Rev. Lett. 110(18), 181101 (2013)

    ADS  Google Scholar 

  41. Oberreiter, L., Gerhardt, I.: Light on a beam splitter: more randomness with single photons. Laser Photonics Rev. 10(1), 108–115 (2016)

    ADS  Google Scholar 

  42. Scully, M.O., Zubairy, M.S.: Quantum Optics. Cambridge University Press, Cambridge (1997)

    Google Scholar 

  43. Momeni-Demneh, M., Mahdifar, A., Roknizadeh, R.: Nonlinear optical effects on the atom-field interaction based on the nonlinear coherent states approach. JOSA B 39(5), 1353–1363 (2022)

    ADS  Google Scholar 

  44. Esposito, M., Ranadive, A., Planat, L., Leger, S., Fraudet, D., Jouanny, V., Buisson, O., Guichard, W., Naud, C., Aumentado, J., et al.: Observation of two-mode squeezing in a traveling wave parametric amplifier. Phys. Rev. Lett. 128(15), 153603 (2022)

    ADS  Google Scholar 

  45. Chen, P., Briggs, I., Hou, S., Fan, L.: Ultra-broadband quadrature squeezing with thin-film lithium niobate nanophotonics. Opt. Lett. 47(6), 1506–1509 (2022)

    ADS  Google Scholar 

  46. Jiang, K., Wei, L.F.: Generating antibunching light by beam-splitting photon number resolved detection from the superbunching squeezed vacuum. Phys. Lett. A 403, 127396 (2021)

    MathSciNet  Google Scholar 

  47. Hillery, M.: Amplitude-squared squeezing of the electromagnetic field. Phys. Rev. A 36(8), 3796 (1987)

    ADS  MathSciNet  Google Scholar 

  48. Boddeda, R., Glorieux, Q., Bramati, A., Pigeon, S.: Generating strong anti-bunching by interfering nonclassical and classical states of light. J. Phys. B: At. Mol. Opt. Phys. 52(21), 215401 (2019)

    ADS  Google Scholar 

  49. Park, T., Stokowski, H., Ansari, V., Gyger, S., Multani, K.K.S., Celik, O.T., Hwang, A.Y., Dean, D.J., Mayor, F., McKenna, T.P., et al.: Single-mode squeezed-light generation and tomography with an integrated optical parametric oscillator. Sci. Adv. 10(11), 1814 (2024)

    ADS  Google Scholar 

  50. Vaswani, C., Mootz, M., Sundahl, C., Mudiyanselage, D.H., Kang, J.H., Yang, X., Cheng, D., Huang, C., Kim, R.H.J., Liu, Z., et al.: Terahertz second-harmonic generation from lightwave acceleration of symmetry-breaking nonlinear supercurrents. Phys. Rev. Lett. 124(20), 207003 (2020)

    ADS  Google Scholar 

  51. Danylov, A.A., Goyette, T.M., Waldman, J., Coulombe, M.J., Gatesman, A.J., Giles, R.H., Goodhue, W.D., Qian, X., Nixon, W.E.: Frequency stabilization of a single mode terahertz quantum cascade laser to the kilohertz level. Opt. Express 17(9), 7525–7532 (2009)

    ADS  Google Scholar 

  52. Walls, D.F., Milburn, G.J.: Generation and applications of squeezed light. Quantum Optics 137–175 (1994)

  53. Rauf, Z., Zubairy, M.S.: Phase fluctuations in a degenerate parametric amplifier. Phys. Rev. A 36(3), 1481 (1987)

    ADS  Google Scholar 

  54. Imamoḡlu, A., Schmidt, H., Woods, G., Deutsch, M.: Strongly interacting photons in a nonlinear cavity. Phys. Rev. Lett. 79(8), 1467 (1997)

Download references

Author information

Authors and Affiliations

  1. Laser and Optics Group, Faculty of Physics, Yazd University, Yazd, Iran

    Azadeh Noury & M. K. Tavassoly

Authors
  1. Azadeh Noury
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. M. K. Tavassoly
    View author publications

    You can also search for this author inPubMed Google Scholar

Contributions

MKT presented the idea, MKT and AN wrote the manuscript text, and AN prepared figures; however, all authors analyzed the results and reviewed the manuscript.

Corresponding author

Correspondence to M. K. Tavassoly.

Ethics declarations

Conflict of interest

The authors declare no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Noury, A., Tavassoly, M.K. Amplification of squeezing and anti-bunching in the output beams from a degenerate parametric amplifier: linear and nonlinear coherent state approaches. Quantum Inf Process 23, 234 (2024). https://doi.org/10.1007/s11128-024-04446-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-024-04446-2

Keywords