Abstract
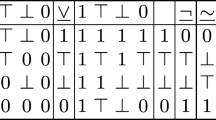
Metacompleteness is used to prove properties such as the disjunction property and the existence property in the area of relevant logics. On the other hand, the disjunction property of several basic propositional substructural logics over FL has been proved using the cut elimination theorem of sequent calculi and algebraic characterization. The present paper shows that Meyer’s metavaluational technique and Slaney’s metavaluational technique can be applied to basic predicate intuitionistic substructural logics and basic predicate involutive substructural logics, respectively. As a corollary of metacompleteness, the disjunction property, the existence property, and the admissibility of certain rules in such logics can be proved.
Similar content being viewed by others
References
Brady, R.T. (ed.), Relevant Logics and Their Rivals, Volume II, Ashgate Publishing Limited, Aldershot, 2003.
Ciabattoni, A., N. Galatos, and K. Terui, From axioms to analytic rules in nonclassical logics, Proceedings of the 2008 23rd Annual IEEE Symposium on Logic in Computer Science (LICS’08), 2008, pp. 229–240.
Dunn, J. M., and G. Restall, Relevance logic, in D. Gabbay and F. Guenthner, (eds.), Handbook of Philosophical Logic, 2nd edition, Vol. 6, Kluwer, Dordrecht, 2002, pp.1–128.
Galatos, N., P. Jipsen, T. Kowalski, and H. Ono, Residuated Lattices: An Algebraic Glimpse at Substructural Logics, Elsevier, 2007.
Harrop R: Concerning formulas of the types \({A \rightarrow B \vee C.\,A \rightarrow \exists xB(x)}\) in intuitionistic systems of logic, Journal of Symbolic Logic 25, 27–32 (1960)
Kleene S. C.: Disjunction and existence under implication in elementary intuitionistic formalisms. Journal of Symbolic Logic 27, 11–18 (1962)
Kreisel G., Putnum H: Eine Unableitbarkeitsbeweismethode für den intuitionistischen Aussagenkalkül. Zeitschrift für Mathematische Logik und Grundlagen der Mathematik 3, 74–78 (1957)
Meyer R. K.: Metacompleteness. Notre Dame Journal of Formal Logic 17, 501–516 (1976)
Restall, G., An Introduction to Substructural Logics, Routledge, 2000.
Schoroeder-Heister, P., and K. Došsen, (eds.), Substructural Logics, Oxford University Press, 1993.
Slaney J. K: A Metacompleteness theorem for contraction-free relevant logics. Studia Logica 43, 159–168 (1984)
Slaney J. K.: Reduced models for relevant logics without WI. Notre Dame Journal of Formal Logic 28, 395–407 (1987)
Souma D: An algebraic approach to the disjunction property of substructural logics. Notre Dame Journal of Formal Logic 48, 489–495 (2007)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Seki, T. Metacompleteness of Substructural Logics. Stud Logica 100, 1175–1199 (2012). https://doi.org/10.1007/s11225-012-9458-z
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11225-012-9458-z