Abstract
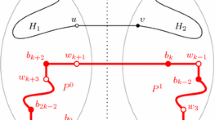
A k -container C(u,v) of a graph G is a set of k disjoint paths between u and v. A k-container C(u,v) of G is a k * -container if it contains all vertices of G. A graph G is k * -connected if there exists a k *-container between any two distinct vertices of G. Therefore, a graph is 1*-connected (respectively, 2*-connected) if and only if it is Hamiltonian connected (respectively, Hamiltonian). A graph G is super spanning connected if there exists a k *-container between any two distinct vertices of G for every k with 1≤k≤κ(G) where κ(G) is the connectivity of G. A bipartite graph G is k * -laceable if there exists a k *-container between any two vertices from different partite set of G. A bipartite graph G is super spanning laceable if there exists a k *-container between any two vertices from different partite set of G for every k with 1≤k≤κ(G). In this paper, we prove that the enhanced hypercube Q n,m is super spanning laceable if m is an odd integer and super spanning connected if otherwise.
Similar content being viewed by others
References
Akers SB, Krishnamurthy B (1989) A group-theoretic model for symmetric interconnection networks. IEEE Trans Comp 38:555–566
Albert M, Aldred REL, Holton D (2001) On 3*-connected graphs. Australas J Combin 24:193–208
Bondy JA, Murty USR (1980) Graph Theory with Applications. North-Holland, New York
Chang CH, Lin CK, Huang HM, Hsu LH (2004) The super laceability of the hypercubes. Inf Process Lett 92:15–21
Chen YC, Tsai CH, Hsu LH, Tan JJM (2004) On some super fault-tolerant Hamiltonian graphs. Appl Math Comput 148:729–741
El-Amawy A, Latifi S (1991) Properties and performance of folded hypercubes. IEEE Trans Parrallel Distributed Syst 2:31–42
Harary F, Lewinter M (1987) Hypercube and other recursively defined hamiltonian laceabe graphs. Congressus Nemerantium 60:81–84
Hsu HC, Lin CK, Huang HM, Hsu LH (2006) The spanning connectivity of the (n,k)-star graphs. Int J Foundations Comput Sci 17:415–434
Hsu DF (1994). On container width and length in graphs, groups, and networks. IEICE Trans Fundam E77-A:668–680
Leighton FT (1992) Introduction to Parallel Algorithms and Architecture: Arrays ⋅ Trees ⋅ Hypercubes. Morgan Kaufmann, San Mateo
Lewinter M, Widulski W (1997) Hyper-hamilton laceable and caterpillar product graphs. Comput Math Appl 34:99–104
Lin CK, Huang HM, Hsu LH (2005) The super connectivity of the pancake graphs and the super laceability of the star graphs. Theor Comput Sci 339:257–271
Lin CK, Huang HM, Hsu LH (2007) On the spanning connectivity of graphs. Discrete Math 307:285–289
Lin CK, Tan JJM, Hsu DF, Hsu LH (2007) On the spanning connectivity and spanning laceability of hypercube-like networks. Theor Comput Sci 381:218–229
Lin CK, Huang HM, Tan JJM, Hsu LH (2008) On spanning connected graphs. Discrete Math 308:1330–1333
Menger K (1927) Zur allgemeinen Kurventheorie. Fundam Mathethatical 10:95–115
Sun CM, Lin CK, Hung HM, Hsu LH (2006) Mutually indepedent hamiltonian paths and cyales in hypercubes. J Interconnect Networks 7:235–256
Tsai CH, Tan JJM, Hsu LH (2004) The super-connected property of recursive circulant graphs. Inf Process Lett 91:293–298
Tzeng NF, Wei S (1991) Enhanced hypercubes. IEEE Trans Comput 40:284–294
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Chang, CH., Lin, CK., Tan, J.J.M. et al. The super spanning connectivity and super spanning laceability of the enhanced hypercubes. J Supercomput 48, 66–87 (2009). https://doi.org/10.1007/s11227-008-0206-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11227-008-0206-0