Skip to main content

Advertisement

Springer Nature Link
Log in

Facial image encryption scheme based on improved 4-D hyperchaotic system

  • Published:
The Journal of Supercomputing Aims and scope Submit manuscript

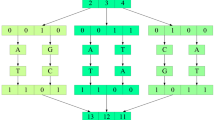
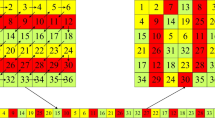
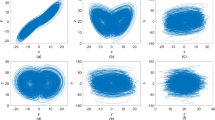
Abstract

To solve the security problem of facial image transmission and storage, we propose a facial image encryption algorithm based on an improved 4-D hyperchaotic system. First, a new 4-D hyperchaotic system is obtained by improving the Chen chaotic system, and then the facial region in the image is recognized and encrypted via the Haar cascade face detection technique. The encryption process is divided into two stages: the scrambling phase uses circular layered and bit-plane splicing V-selective scrambling to alter pixel values, and the diffusion phase employs bit-level DNA cross-encoding and arithmetic operations. These techniques increase the complexity and randomness of encryption, increasing its resistance to brute-force attack. Finally, the encrypted facial region is combined with the non-face region. The experimental results show that the number of pixel change rates and the unified average change intensity of the encrypted image are near ideal, with an information entropy of 7.9976 bits. Security analysis confirms that the algorithm can withstand various attacks.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig.7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Algorithm 1
Fig. 14
Fig. 15
Algorithm 2
Algorithm 3
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25

Similar content being viewed by others

Data availability

The data used to support the findings of this study are included in the article.

References

  1. Dai WY, Li BB, Du QY et al (2024) Chaos-based index-of-min hashing scheme for cancellable biometrics security. IEEE Trans Inf Forensics Secur 19:8982–8997

    Article  MATH  Google Scholar 

  2. Fu SC, Dong F, Shen D et al (2024) Joint optimization of device selection and resource allocation for multiple federations in federated edge learning. IEEE Trans Serv Comput 17(1):251–262

    Article  MATH  Google Scholar 

  3. Wang Y, Quan C, Tay CJ (2014) Nonlinear multiple-image encryption based on mixture retrieval algorithm in Fresnel domain. Optics Communications 330:91–98

    Article  MATH  Google Scholar 

  4. Zahmoul R, Ejbali R, Zaied M (2017) Image encryption based on new Beta chaotic maps. Opt Lasers Eng 96:39–49

    Article  Google Scholar 

  5. Shao Z, Liu X, Yao Q et al (2020) Multiple-image encryption based on chaotic phase mask and equal modulus decomposition in quaternion gyrator domain. Signal Processing: Image Communication 80:115662

    Google Scholar 

  6. Shao Z, Li L, Li B et al (2024) Quaternion-based 2D-DOST and stacked principal component analysis network for multimodal face recognition. Appl Soft Comput 166:112154

    Article  MATH  Google Scholar 

  7. Lorenz EN, Haman K (1996) The essence of chaos. Pure Appl Geophys 147(3):598–599

    MATH  Google Scholar 

  8. Zhang Y (2018) The unified image encryption algorithm based on chaos and cubic S-Box. Inf Sci 450:361–377

    Article  MathSciNet  MATH  Google Scholar 

  9. Kumar CM, Vidhya R, Brindha M (2022) An efficient chaos based image encryption algorithm using enhanced thorp shuffle and chaotic convolution function. Appl Intell 52(3):2556–2585

    Article  MATH  Google Scholar 

  10. Guo Z, Chen HS, Zhou L et al (2024) Optical image encryption and authentication scheme with computational ghost imaging. Appl Math Model 131:49–66

    Article  MathSciNet  MATH  Google Scholar 

  11. Fridrich J (1998) Symmetric ciphers based on two-dimensional chaotic maps. Int J Bifurcation Chaos 8(06):1259–1284

    Article  MathSciNet  MATH  Google Scholar 

  12. Hu LL, Chen MX, Wang MM et al (2024) A multi-image encryption scheme based on block compressive sensing and nonlinear bifurcation diffusion. Chaos, Solitons Fractals 188:115521

    Article  MATH  Google Scholar 

  13. Matthews R (1989) On the derivation of a “chaotic” encryption algorithm. Cryptologia 13(1):29–42

    Article  MathSciNet  MATH  Google Scholar 

  14. Hua Z, Zhu Z, Chen Y et al (2021) Color image encryption using orthogonal Latin squares and a new 2D chaotic system. Nonlinear Dyn 104(4):4505–4522

    Article  MATH  Google Scholar 

  15. Wang X, Su Y, Xu M et al (2022) A new image encryption algorithm based on Latin square matrix. Nonlinear Dyn 107:1277–1293

    Article  MATH  Google Scholar 

  16. Wei F, Xiangyu Z, Jing Z et al (2022) Image encryption algorithm based on plane-level image filtering and discrete logarithmic transform. Mathematics 10(15):2751–2751

    Article  MATH  Google Scholar 

  17. Li B, Liao X, Jiang Y (2018) A novel image encryption scheme based on logistic map and dynatomic modular curve. Multimedia Tools Appl 77:8911–8938

    Article  MATH  Google Scholar 

  18. Hua Z, Zhou Y (2016) Image encryption using 2D logistic-adjusted-Sine map. Inf Sci 339:237–253

    Article  MATH  Google Scholar 

  19. Shen YX, Tang C, Xu M et al (2021) Optical selective encryption based on the FRFCM algorithm and face biometric for the medical image. Optics Laser Technol 138:106911

    Article  MATH  Google Scholar 

  20. Teng L, Du L, Leng Z et al (2024) Chaotic image encryption based on partial face recognition and DNA diffusion. Appl Intell 54(21):10360–10373

    Article  MATH  Google Scholar 

  21. Xue H, Du J, Li S et al (2018) Region of interest encryption for color images based on a hyperchaotic system with three positive Lyapunov exponets. Opt Laser Technol 106:506–516

    Article  MATH  Google Scholar 

  22. Liu Y, Zhang J, Han D et al (2020) A multidimensional chaotic image encryption algorithm based on the region of interest. Multimedia Tools Appl 79:1–37

    MATH  Google Scholar 

  23. Ullah N, Javed A, Ghazanfar MA et al (2022) A novel DeepMaskNet model for face mask detection and masked facial recognition. J King Saud Univ-Comput Inform Sci 34(10):9905–9914

    MATH  Google Scholar 

  24. Wang X, Guan N, Liu P (2022) A selective image encryption algorithm based on a chaotic model using modular sine arithmetic. Optik 258:168955

    Article  MATH  Google Scholar 

  25. Meng F, Wu G (2024) A color image encryption and decryption scheme based on extended DNA coding and fractional-order 5D hyper-chaotic system. Expert Syst Appl 254:124413

    Article  MATH  Google Scholar 

  26. Li D, Li J, Di X (2022) A novel exponential one-dimensional chaotic map enhancer and its application in an image encryption scheme using modified ZigZag transform. J Inform Security Appl 69:103304

    MATH  Google Scholar 

  27. Zhang X, Hu Y (2021) Multiple-image encryption algorithm based on the 3D scrambling model and dynamic DNA coding. Opt Laser Technol 141:107073

    Article  MATH  Google Scholar 

  28. Jithin KC, Sankar S (2020) Colour image encryption algorithm combining Arnold map, DNA sequence operation, and a Mandelbrot set. J Inform Security Appl 50:102428

    MATH  Google Scholar 

  29. Zhang Q, Guo L, Wei X (2010) Image encryption using DNA addition combining with chaotic maps. Math Comput Model 52(11–12):2028–2035

    Article  MathSciNet  MATH  Google Scholar 

  30. Alexan W, Mamdouh E, Aboshousha A et al (2024) Stegocrypt: A robust tri-stage spatial steganography algorithm using TLM encryption and DNA coding for securing digital images. IET Image Proc 18(13):4189–4206

    Article  MATH  Google Scholar 

  31. Xu J, Zhao B, Wu Z (2022) Research on color image encryption algorithm based on bit-plane and Chen chaotic system. Entropy 24(2):186

    Article  MATH  Google Scholar 

  32. Demirkol A, Sahin M, Karakaya B et al (2024) Real time hybrid medical image encryption algorithm combining memristor-based chaos with DNA coding. Chaos, Solitons Fractals 183:114923

    Article  MathSciNet  MATH  Google Scholar 

  33. Kaur B, Jindal S (2015) Accelerating CBIR system using graphics processing unit in OpenCV environment. Int J Comput Appl 975:8887

    MATH  Google Scholar 

  34. Viola P, Jones M (2001) Rapid object detection using a boosted cascade of simple features, In: Proceedings of the 2001 IEEE computer society conference on computer vision and pattern recognition. CVPR 2001. IEEE, 1: 1.

  35. Zafeiriou S, Zhang C, Zhang Z (2015) A survey on face detection in the wild: past, present and future. Comput Vis Image Underst 138:1–24

    Article  MATH  Google Scholar 

  36. Zhang X, Liu Q (2014) Face detection: a survey. Comput Vis Image Underst 95(3):299–324

    MATH  Google Scholar 

  37. Liu H, Teng L, Unar S et al (2024) Fingerprint image encryption based on chaos and nonlinear dynamic “X” model diffusion. J Inform Security Appl 82:103723

    MATH  Google Scholar 

  38. Barboza R (2007) Dynamics of a hyperchaotic lorenz system. Int J Bifurc Chaos17(12):4285–4294

    Article  MathSciNet  MATH  Google Scholar 

  39. Wang SM, Sun BC, Wang YM et al (2024) Image encryption algorithm using multi-base diffusion and a new four-dimensional chaotic system. Multimedia Tools Appl 83(4):10039–10060

    Article  MATH  Google Scholar 

  40. Su Y, Wang X (2022) Characteristic analysis of new four-dimensional autonomous power system and its application in color image encryption. Multimedia Syst 28:553–571

    Article  MATH  Google Scholar 

  41. Feng W, Zhang J, Chen Y et al (2024) Exploiting robust quadratic polynomial hyperchaotic map and pixel fusion strategy for efficient image encryption. Expert Syst Appl 246:123190

    Article  Google Scholar 

  42. Feng W, Wang Q, Liu H et al (2023) Exploiting newly designed fractional-order 3D Lorenz chaotic system and 2D discrete polynomial hyper-chaotic map for high-performance multi-image encryption. Fractal Fract 7(12):887

    Article  MATH  Google Scholar 

  43. Rezaei B, Ghanbari H, Enayatifar R (2023) An image encryption approach using tuned Henon chaotic map and evolutionary algorithm. Nonlinear Dyn 111(10):9629–9647

    Article  MATH  Google Scholar 

  44. Wang X, Xu D (2014) A novel image encryption scheme based on Brownian motion and PWLCM chaotic system. Nonlinear Dyn 75:345–353

    Article  MATH  Google Scholar 

  45. Luo Y, Du M, Liu J (2015) A symmetrical image encryption scheme in wavelet and time domain. Commun Nonlinear Sci Numer Simul 20(2):447–460

    Article  MATH  Google Scholar 

  46. Belazi A, Kharbech S, Aslam MN et al (2022) Improved Sine-Tangent chaotic map with application in medical images encryption. J Inform Security Appl 66:103131

    MATH  Google Scholar 

  47. Zhou L, Lin Z, Tan F (2024) Multiple-image encryption scheme based on a new 2D hyperchaotic map with blurred pixels. Phys Scr 99(4):045247

    Article  Google Scholar 

  48. Ur Rehman A, Liao X, Wang H (2021) An innovative technique for image encryption using tri-partite graph and chaotic maps. Multimedia Tools Appl 80(14):21979–22005

    Article  MATH  Google Scholar 

  49. Chai X, Fu X, Gan Z et al (2019) A color image cryptosystem based on dynamic DNA encryption and chaos. Signal Process 155:44–62

    Article  MATH  Google Scholar 

  50. Wu J, Liao X, Yang B (2018) Image encryption using 2D Hénon-Sine map and DNA approach. Signal Process 153:11–23

    Article  MATH  Google Scholar 

  51. Panwar A, Biban G, Chugh R et al (2024) An efficient image encryption model based on 6D hyperchaotic system and symmetric matrix for color and gray images. Heliyon 10:e31618

    Article  MATH  Google Scholar 

  52. Verma V, Kumar S (2024) Quantum image encryption algorithm based on 3D-BNM chaotic map. Nonlinear Dynam 113:1–27

    MATH  Google Scholar 

  53. Huang X, Tang J, Zhang Z (2024) Efficient and secure image encryption algorithm using 2D LIM map and Latin square matrix. Nonlinear Dyn 112:1–21

    Article  MATH  Google Scholar 

  54. Guo Z, Sun P (2022) Improved reverse zigzag transform and DNA diffusion chaotic image encryption method. Multimedia Tools Appl 81(8):11301–11323

    Article  MATH  Google Scholar 

  55. Zhao MJ, Luo YB, Yuan Z et al (2024) A fast color image encryption scheme based on the new chaotic structure and dynamic strong S-boxes. Nonlinear Dynam. https://doi.org/10.1007/s11071-024-10541-x

    Article  MATH  Google Scholar 

  56. Long GQ, Chai XL, Gan ZH et al (2023) Exploiting one-dimensional exponential Chebyshev chaotic map and matching embedding for visually meaningful image encryption. Chaos, Solitons Fractals 176:114111

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by the National Natural Science Foundation of China under Grants 62102374 and 62072417 and the Henan Provincial Science and Technology Research Project under Grant 242102210141.

Author information

Authors and Affiliations

  1. College of Electrical Information Engineering, Zhengzhou University of Light Industry, Zhengzhou, 450000, Henan, China

    Xuncai Zhang & Mengrui Liu

  2. College of Building Environment Engineering, Zhengzhou University of Light Industry, Zhengzhou, 450000, Henan, China

    Ying Niu

Authors
  1. Xuncai Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Mengrui Liu
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Ying Niu
    View author publications

    You can also search for this author inPubMed Google Scholar

Contributions

X.C.Z. and M.R.L. conceived the concept; M.R.L wrote the codes, performed the simulations and analyzed the data; X.C.Z. discussed the data. Y.N. contributed to refining the ideas and carrying out additional analyses and revisions. All authors contributed to the writing of the paper.

Corresponding author

Correspondence to Ying Niu.

Ethics declarations

Conflicts of interest

The authors declare that they have no conflicts of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, X., Liu, M. & Niu, Y. Facial image encryption scheme based on improved 4-D hyperchaotic system. J Supercomput 81, 454 (2025). https://doi.org/10.1007/s11227-025-06943-2

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11227-025-06943-2

Keywords