Skip to main content

Advertisement

Springer Nature Link
Log in

High-capacity quantum wireless multi-hop network communication based on hyperentangled Bell states

  • Published:
The Journal of Supercomputing Aims and scope Submit manuscript

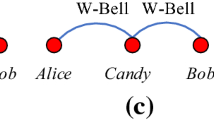
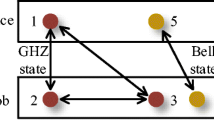
Abstract

Quantum wireless multi-hop networks are a fundamental component of the global quantum communication infrastructure. However, existing quantum communication protocols are constrained by limitations in capacity and efficiency, primarily due to their reliance on a single degree of freedom for encoding quantum information. In contrast, the protocol presented in this study leverages hyperentangled Bell states across both polarization and spatial-mode degrees of freedom, thereby overcoming these limitations. Compared to traditional approaches that employ bipartite entanglement within a single polarization degree of freedom, the proposed protocol significantly enhances the communication capacity. This innovative approach not only improves the overall efficiency of quantum communication but also contributes to the scalability of quantum multi-hop networks, offering a promising solution to the challenges encountered by current quantum communication protocols.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

All data generated or analyzed during this study are included in this published article.

References

  1. Cheng S-T, Wang C-Y, Tao M-H (2005) Quantum communication for wireless wide-area networks. IEEE J Sel Areas Commun 23(7):1424–1432

    Article  MATH  Google Scholar 

  2. Li Z-Z, Xu G, Chen X-B, Sun X, Yang Y-X (2016) Multi-user quantum wireless network communication based on multi-qubit ghz state. IEEE Commun Lett 20(12):2470–2473

    Article  MATH  Google Scholar 

  3. Yu X-T, Xu J, Zhang Z-C (2012) Routing protocol for wireless ad hoc quantum communication network based on quantum teleportation

  4. Yu X-T, Xu J, Zhang Z-C (2013) Distributed wireless quantum communication networks. Chin Phys B 22(9):090311

    Article  MATH  Google Scholar 

  5. Wang K, Yu X-T, Lu S-L, Gong Y-X (2014) Quantum wireless multihop communication based on arbitrary bell pairs and teleportation. Phys Rev A 89(2):022329

    Article  Google Scholar 

  6. Wang K, Gong Y-X, Yu X-T, Lu S-L (2014) Addendum to “quantum wireless multihop communication based on arbitrary bell pairs and teleportation’’. Phys Rev A 90(4):044302

    Article  Google Scholar 

  7. Gao X-Q, Zhang Z-C, Sheng B (2018) Multi-hop teleportation in a quantum network based on mesh topology. Front Phys 13:1–7

    Article  MATH  Google Scholar 

  8. Shi L-H, Yu X-T, Cai X-F, Gong Y-X, Zhang Z-C (2015) Quantum information transmission in the quantum wireless multihop network based on werner state. Chin Phys B 24(5):050308

    Article  MATH  Google Scholar 

  9. Xiong P-Y, Yu X-T, Zhang Z-C, Zhan H-T, Hua J-Y (2017) Routing protocol for wireless quantum multi-hop mesh backbone network based on partially entangled ghz state. Front Phys 12:1–10

    Article  MATH  Google Scholar 

  10. Xiong P-Y, Yu X-T, Zhan H-T, Zhang Z-C (2016) Multiple teleportation via partially entangled ghz state. Front Phys 11:1–8

    Article  MATH  Google Scholar 

  11. Zhan H-T, Yu X-T, Xiong P-Y, Zhang Z-C (2016) Multi-hop teleportation based on w state and epr pairs. Chin Phys B 25(5):050305

    Article  MATH  Google Scholar 

  12. Zhang Z, Wang J, Sun M (2018) Multihop teleportation via the composite of asymmetric w state and bell state. Int J Theor Phys 57:3605–3620

    Article  MathSciNet  MATH  Google Scholar 

  13. Zou Z-Z, Yu X-T, Gong Y-X, Zhang Z-C (2017) Multihop teleportation of two-qubit state via the composite ghz-bell channel. Phys Lett A 381(2):76–81

    Article  MATH  Google Scholar 

  14. Zhou X-Z, Yu X-T, Zhang Z-C (2018) Multi-hop teleportation of an unknown qubit state based on w states. Int J Theor Phys 57:981–993

    Article  MathSciNet  MATH  Google Scholar 

  15. Cai X-F, Yu X-T, Shi L-H, Zhang Z-C (2014) Partially entangled states bridge in quantum teleportation. Front Phys 9:646–651

    Article  MATH  Google Scholar 

  16. Barreiro JT, Langford NK, Peters NA, Kwiat PG (2005) Generation of hyperentangled photon pairs. Phys Rev Lett 95(26):260501

    Article  MATH  Google Scholar 

  17. Ren B-C, Deng F-G (2014) Hyper-parallel photonic quantum computation with coupled quantum dots. Sci Rep 4(1):4623

    Article  MATH  Google Scholar 

  18. Ren B-C, Wang G-Y, Deng F-G (2015) Universal hyperparallel hybrid photonic quantum gates with dipole-induced transparency in the weak-coupling regime. Phys Rev A 91(3):032328

    Article  MATH  Google Scholar 

  19. Li T, Long G-L (2016) Hyperparallel optical quantum computation assisted by atomic ensembles embedded in double-sided optical cavities. Phys Rev A 94(2):022343

    Article  Google Scholar 

  20. Wei H-R, Deng F-G, Long GL (2016) Hyper-parallel toffoli gate on three-photon system with two degrees of freedom assisted by single-sided optical microcavities. Opt Express 24(16):18619–18630

    Article  Google Scholar 

  21. Ren B-C, Deng F-G (2017) Robust hyperparallel photonic quantum entangling gate with cavity qed. Opt Express 25(10):10863–10873

    Article  MATH  Google Scholar 

  22. Sheng Y-B, Deng F-G, Long GL (2010) Complete hyperentangled-bell-state analysis for quantum communication. Phys Rev A-Atomic, Mol , and Opt Phys 82(3):032318

    Article  MATH  Google Scholar 

  23. Ren B-C, Wei H-R, Hua M, Li T, Deng F-G (2012) Complete hyperentangled-bell-state analysis for photon systems assisted by quantum-dot spins in optical microcavities. Opt Express 20(22):24664–24677

    Article  MATH  Google Scholar 

  24. Wang T-J, Lu Y, Long GL (2012) Generation and complete analysis of the hyperentangled bell state for photons assisted by quantum-dot spins in optical microcavities. Phys Rev A-Atomic, Mol , and Opt Phys 86(4):042337

    Article  MATH  Google Scholar 

  25. Liu Q, Zhang M (2015) Generation and complete nondestructive analysis of hyperentanglement assisted by nitrogen-vacancy centers in resonators. Phys Rev A 91(6):062321

    Article  Google Scholar 

  26. Wang M, Yan F, Gao T (2018) Deterministic state analysis for polarization-spatial-time-bin hyperentanglement with nonlinear optics. Laser Phys Lett 15(12):125206

    Article  MATH  Google Scholar 

  27. Wang G-Y, Ren B-C, Deng F-G, Long G-L (2019) Complete analysis of hyperentangled bell states assisted with auxiliary hyperentanglement. Opt Express 27(6):8994–9003

    Article  MATH  Google Scholar 

  28. Li X-H, Ghose S (2017) Hyperentangled bell-state analysis and hyperdense coding assisted by auxiliary entanglement. Phys Rev A 96(2):020303

    Article  MATH  Google Scholar 

  29. Li X-H, Ghose S (2016) Self-assisted complete maximally hyperentangled state analysis via the cross-kerr nonlinearity. Phys Rev A 93(2):022302

    Article  MATH  Google Scholar 

  30. Zheng Y, Liang L, Zhang M (2019) Error-heralded generation and self-assisted complete analysis of two-photon hyperentangled bell states through single-sided quantum-dot-cavity systems. Sci China Phys , Mech & Astron 62:1–9

    Article  MATH  Google Scholar 

  31. Huang C-X, Hu X-M, Liu B-H, Zhou L, Sheng Y-B, Li C-F, Guo G-C (2022) Experimental one-step deterministic polarization entanglement purification. Sci Bull 67(6):593–597

    Article  MATH  Google Scholar 

  32. Yasir PA, Chandrashekar C (2022) Generation of hyperentangled states and two-dimensional quantum walks using j or q plates and polarization beam splitters. Phys Rev A 105(1):012417

    Article  MATH  Google Scholar 

  33. Zhao P, Yang M-Y, Zhu S, Zhou L, Zhong W, Du M-M, Sheng Y-B (2023) Generation of hyperentangled state encoded in three degrees of freedom. Sci China Phys, Mech & Astron 66(10):100311

    Article  Google Scholar 

  34. Zhao P, Yang M-Y, Zhu S, Zhou L, Zhong W, Du M-M, Sheng Y-B (2023) Generation of hyperentangled state encoded in three degrees of freedom. Sci China Phys, Mech & Astron 66(10):100311

    Article  Google Scholar 

  35. Zhao P, Ying J-W, Yang M-Y, Zhong W, Du M-M, Shen S-T, Li Y-X, Zhang A-L, Zhou L, Sheng Y-B (2024) Direct generation of multi-photon hyperentanglement. arXiv preprint arXiv:2406.08790

  36. Wang M, Guo H, Yan F, Gao T (2023) Quantum remote implementation with polarization-temporal hyperentanglement. Phys Rev Appl 20(4):044016

    Article  MATH  Google Scholar 

  37. Lu H-H, Alshowkan M, Myilswamy KV, Weiner AM, Lukens JM, Peters NA (2023) Generation and characterization of ultrabroadband polarization-frequency hyperentangled photons. Opt Lett 48(22):6031–6034

    Article  Google Scholar 

  38. Wang X-L, Cai X-D, Su Z-E, Chen M-C, Wu D, Li L, Liu N-L, Lu C-Y, Pan J-W (2015) Quantum teleportation of multiple degrees of freedom of a single photon. Nature 518(7540):516–519

    Article  MATH  Google Scholar 

  39. Wang G-Y, Ai Q, Ren B-C, Li T, Deng F-G (2016) Error-detected generation and complete analysis of hyperentangled bell states for photons assisted by quantum-dot spins in double-sided optical microcavities. Opt Express 24(25):28444–28458

    Article  Google Scholar 

  40. Wang T-J, Song S-Y, Long GL (2012) Quantum repeater based on spatial entanglement of photons and quantum-dot spins in optical microcavities. Phys Rev A-Atomic, Mol , Opt Phys 85(6):062311

    Article  MATH  Google Scholar 

  41. Kwiat PG, Weinfurter H (1998) Embedded bell-state analysis. Phys Rev A 58(4):2623

    Article  MathSciNet  MATH  Google Scholar 

  42. Walborn S, Pádua S, Monken C (2003) Hyperentanglement-assisted bell-state analysis. Phys Rev A 68(4):042313

    Article  MathSciNet  Google Scholar 

  43. Schuck C, Huber G, Kurtsiefer C, Weinfurter H (2006) Complete deterministic linear optics bell state analysis. Phys Rev Lett 96(19):190501

    Article  Google Scholar 

  44. Barbieri M, Vallone G, Mataloni P, De Martini F (2007) Complete and deterministic discrimination of polarization bell states assisted by momentum entanglement. Phys Rev A-Atomic, Mol, Opt Phys 75(4):042317

    Article  MATH  Google Scholar 

  45. Zeng Z, Zhu K-D (2020) Complete hyperentangled bell state analysis assisted by hyperentanglement. Laser Phys Lett 17(7):075203

    Article  Google Scholar 

  46. Zeng Z, Zhu K-D (2020) Complete hyperentangled state analysis using weak cross-kerr nonlinearity and auxiliary entanglement. New J Phys 22(8):083051

    Article  MathSciNet  MATH  Google Scholar 

  47. Sheng Y-B, Deng F-G (2010) Deterministic entanglement purification and complete nonlocal bell-state analysis with hyperentanglement. Phys Rev A-Atomic, Mol, Opt Phys 81(3):032307

    Article  MATH  Google Scholar 

  48. Sheng Y-B, Deng F-G (2010) One-step deterministic polarization-entanglement purification using spatial entanglement. Phys Rev A-Atomic, Mol, and Opt Phys 82(4):044305

    Article  MATH  Google Scholar 

  49. Li X-H (2010) Deterministic polarization-entanglement purification using spatial entanglement. Phys Rev A-Atomic, Mol, and Opt Phys 82(4):044304

    Article  MATH  Google Scholar 

  50. Deng F-G (2011) One-step error correction for multipartite polarization entanglement. Phys Rev A-Atomic, Mol, Opt Phys 83(6):062316

    Article  Google Scholar 

  51. Sheng Y-B, Zhou L (2014) Deterministic polarization entanglement purification using time-bin entanglement. Laser Phys Lett 11(8):085203

    Article  MATH  Google Scholar 

  52. Ren B-C, Du F-F, Deng F-G (2014) Two-step hyperentanglement purification with the quantum-state-joining method. Phys Rev A 90(5):052309

    Article  MATH  Google Scholar 

  53. Wang G-Y, Liu Q, Deng F-G (2016) Hyperentanglement purification for two-photon six-qubit quantum systems. Phys Rev A 94(3):032319

    Article  MATH  Google Scholar 

  54. Wang M, Yan F, Gao T (2020) Entanglement purification of two-photon systems in multiple degrees of freedom. Quantum Inf Process 19:1–18

    Article  MathSciNet  MATH  Google Scholar 

  55. Du F-F, Li T, Long G-L (2016) Refined hyperentanglement purification of two-photon systems for high-capacity quantum communication with cavity-assisted interaction. Ann Phys 375:105–118

    Article  MATH  Google Scholar 

  56. Hu X-M, Huang C-X, Sheng Y-B, Zhou L, Liu B-H, Guo Y, Zhang C, Xing W-B, Huang Y-F, Li C-F et al (2021) Long-distance entanglement purification for quantum communication. Phys Rev Lett 126(1):010503

    Article  MATH  Google Scholar 

  57. Ecker S, Sohr P, Bulla L, Huber M, Bohmann M, Ursin R (2021) Experimental single-copy entanglement distillation. Phys Rev Lett 127(4):040506

    Article  Google Scholar 

  58. Huang C-X, Hu X-M, Liu B-H, Zhou L, Sheng Y-B, Li C-F, Guo G-C (2022) Experimental one-step deterministic polarization entanglement purification. Sci Bull 67(6):593–597

    Article  MATH  Google Scholar 

  59. Cozzolino D, Da Lio B, Bacco D, Oxenløwe LK (2019) High-dimensional quantum communication: benefits, progress, and future challenges. Adv Quantum Technol 2(12):1900038

    Article  MATH  Google Scholar 

  60. Wu X-D, Zhou L, Zhong W, Sheng Y-B (2020) High-capacity measurement-device-independent quantum secure direct communication. Quantum Inf Process 19:1–14

    Article  MathSciNet  MATH  Google Scholar 

  61. Li L-Y, Wang T-J, Wang C (2020) The analysis of high-capacity quantum secure direct communication using polarization and orbital angular momentum of photons. Mod Phys Lett B 34(02):2050017

    Article  MathSciNet  MATH  Google Scholar 

  62. Zhao R-T, Cheng L-L (2020) High capacity information transmission with single-photon in polarization and spatial mode degrees of freedom over a collective noisy channel. Laser Phys 30(6):065204

    Article  MATH  Google Scholar 

  63. Pan H-M (2020) Improvement of high-capacity three-party quantum secret sharing with single photons in both the polarization and the spatial-mode degrees of freedom. Int J Theor Phys 59(7):2208–2213

    Article  MathSciNet  MATH  Google Scholar 

  64. Lai H, Pieprzyk J, Luo M-X, Zhan C, Pan L, Orgun MA (2020) High-capacity (2, 3) threshold quantum secret sharing based on asymmetric quantum lossy channels. Quantum Inf Process 19(5):157

    Article  MathSciNet  MATH  Google Scholar 

  65. Amitonova LV, Tentrup TB, Vellekoop IM, Pinkse PW (2020) Quantum key establishment via a multimode fiber. Opt Express 28(5):5965–5981

    Article  Google Scholar 

  66. Khorasani FM, Houshmand M, Anzabi-Nezhad NS (2020) Authenticated controlled quantum secure direct communication protocol based on five-particle brown states. Int J Theor Phys 59(5):1612–1622

    Article  MathSciNet  MATH  Google Scholar 

  67. Nape I, Otte E, Vallés A, Rosales-Guzmán C, Cardano F, Denz C, Forbes A (2018) Self-healing high-dimensional quantum key distribution using hybrid spin-orbit bessel states. Opt Express 26(21):26946–26960

    Article  Google Scholar 

  68. Nawaz M, Ikram M et al (2018) Remote state preparation through hyperentangled atomic states. J Phys B: Atomic, Mol Opt Phys 51(7):075501

    Article  MATH  Google Scholar 

  69. Cañas G, Vera N, Cariñe J, González P, Cardenas J, Connolly P, Przysiezna A, Gómez E, Figueroa M, Vallone G et al (2017) High-dimensional decoy-state quantum key distribution over multicore telecommunication fibers. Phys Rev A 96(2):022317

    Article  Google Scholar 

  70. Steinlechner F, Ecker S, Fink M, Liu B, Bavaresco J, Huber M, Scheidl T, Ursin R (2017) Distribution of high-dimensional entanglement via an intra-city free-space link. Nat Commun 8(1):1–7

    Article  Google Scholar 

  71. Williams BP, Sadlier RJ, Humble TS (2017) Superdense coding over optical fiber links with complete bell-state measurements. Phys Rev Lett 118(5):050501

    Article  Google Scholar 

  72. Zeng H, Du M-M, Zhong W, Zhou L, Sheng Y-B (2024) High-capacity device-independent quantum secure direct communication based on hyper-encoding. Fundam Res 4(4):851–857

    Article  MATH  Google Scholar 

  73. Sheng Y-B, Zhou L, Long G-L (2022) One-step quantum secure direct communication. Sci Bull 67(4):367–374

    Article  MATH  Google Scholar 

  74. Zhou L, Sheng Y-B (2022) One-step device-independent quantum secure direct communication. Sci China Phys, Mech & Astron 65(5):250311

    Article  MathSciNet  MATH  Google Scholar 

  75. Ying J-W, Zhou L, Zhong W, Sheng Y-B (2022) Measurement-device-independent one-step quantum secure direct communication. Chin Phys B 31(12):120303

    Article  MATH  Google Scholar 

  76. Lo H-K, Chau HF (1999) Unconditional security of quantum key distribution over arbitrarily long distances. Science 283(5410):2050–2056

    Article  MATH  Google Scholar 

  77. Bennett CH, Brassard G, Popescu S, Schumacher B, Smolin JA, Wootters WK (1996) Purification of noisy entanglement and faithful teleportation via noisy channels. Phys Rev Lett 76(5):722

    Article  MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by the Youth Fund Project of Beijing Wuzi University (No. 2022XJQN21), and R&D Program of Beijing Municipal Education Commission(KM202310037003).

Author information

Authors and Affiliations

  1. School of Information, Beijing Wuzi University, Beijing, 101149, China

    Yongli Yang & Yueming Su

  2. Faculty of Information Technology, Beijing University of Technology, Beijing, 100124, China

    Yuguang Yang

Authors
  1. Yongli Yang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yuguang Yang
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Yueming Su
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yueming Su.

Ethics declarations

Confict of interest

The authors declare that they have no confict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, Y., Yang, Y. & Su, Y. High-capacity quantum wireless multi-hop network communication based on hyperentangled Bell states. J Supercomput 81, 641 (2025). https://doi.org/10.1007/s11227-025-07082-4

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11227-025-07082-4

Keywords