Skip to main content
Springer Nature Link
Log in

Ultrasonic digital signal processing simulation in viscoelastic medium with generalized parametric function

  • Published:
Telecommunication Systems Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

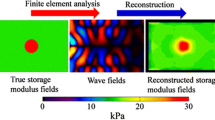
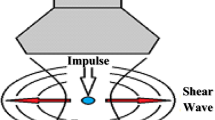
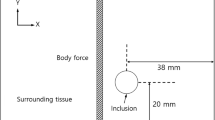
Abstract

This paper describes a simulation of signal pathway in an ultrasonic A-scan. It includes transient diffraction acoustic field, transducer response, absorption and dispersion in viscoelastic medium with arbitrary distribution function. The approach selected here aims at developing digital computer simulation of the physical process that underlies these effects. The soft tissue is considered as a viscoelastic medium, and the relaxation theory has been used to calculate the absorption and dispersion data in the tissue. A signal processing technique is developed in this paper to estimate the degradation in range of resolution due to wave absorption.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Zhang, M., Castaneda, B., Wu, Z., Nigwekar, P., Joseph, J. V., Rubens, D. J., & Parker, K. J. (2007). Congruence of imaging estimators and mechanical measurements of viscoelastic properties of soft tissues. Ultrasound in Medicine & Biology, 33(10), 1617–1631.

    Article  Google Scholar 

  2. Park, E., & Maniatty, A. M. (2006). Shear modulus reconstruction in dynamic elastography: time harmonic case. Physics in Medicine and Biology, 51, 3697–3721.

    Article  Google Scholar 

  3. Yang, X. Church, C. C. (2006) A simple viscoelastic model for soft tissues in the frequency range 6–20 MHz. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 53, 1404–1411.

    Article  Google Scholar 

  4. Nasseri, S., Bilston, L. E., & Phan-Thien, N. (2002). Viscoelastic properties of pig kidney in shear, experimental results and modelling. Rheologica Acta, 41, 180–192.

    Article  Google Scholar 

  5. Fields, S., & Dunn, F. (1976). Correlation of echographic visualizability of tissue with biological composition and physiological state. The Journal of the Acoustical Society of America, 60, 1409–1412.

    Article  Google Scholar 

  6. Kallel, F., & Ophir, J. (1997). A least-squares estimator for elastography. Ultrasonic Imaging, 19, 195–208.

    Article  Google Scholar 

  7. Alam, S. K., Ophir, J., & Konofagou, E. E. (1998). An adaptive strain estimator for elastography. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 45, 461–472.

    Article  Google Scholar 

  8. Konofagou, E. E., Varghese, T., Ophir, J., & Alam, S. K. (1999). Power spectral strain estimators in elastography. Ultrasound in Medicine & Biology, 25(7), 1115–1129.

    Article  Google Scholar 

  9. Varghese, T., Konofagou, E. E., Ophir, J., Alam, S. K., & Bilgen, M. (2000). Direct strain estimation in elastography using spectral cross-correlation. Ultrasound in Medicine & Biology, 26(9), 1525–1537.

    Article  Google Scholar 

  10. Céspedes, I., & Ophir, J. (1993). Reduction of image noise in elastography. Ultrasonic Imaging, 15(2), 89–102.

    Google Scholar 

  11. Ophir, J., Alam, S. K., Garra, B., Kallel, F., Konofagou, E., Krouskop, T., & Varghese, T. (1999). Elastography: ultrasonic estimation and imaging of the elastic properties of tissues. Proceedings—Institution of Mechanical Engineers, 213, 203–233.

    Article  Google Scholar 

  12. Gennisson, J.-L., Deffieux, T., Macé, E., Montaldo, G., Fink, M., & Tanter, M. (2010). Viscoelastic and anisotropic mechanical properties of in vivo muscle tissue assessed by supersonic shear imaging. Ultrasound in Medicine & Biology, 36(5), 789–801.

    Article  Google Scholar 

  13. Chen, Y. (2010). Acoustical transmission line model for ultrasonic transducers for wide-bandwidth application. Acta Mechanica Solida Sinica, 23(2), 124–134.

    Google Scholar 

  14. Nguyen, V., Naili, S., & Sansalone, V. (2010). Simulation of ultrasonic wave propagation in anisotropic cancellous bone immense in fluid. Wave Motion, 47(2), 117–129.

    Article  Google Scholar 

  15. Nadarajah, S., & Gupta, A. K. (2007). A generalized gamma distribution with application to drought data. Mathematics and Computers in Simulation, 74, 1–7.

    Article  Google Scholar 

  16. Kak, A. C., & Dines, K. A. (1978). Signal processing of broadband pulsed ultrasound: measurement of attenuation of soft biological tissues. IEEE Transactions on Biomedical Engineering, BME-25, 321–344.

    Article  Google Scholar 

  17. Ophir, J., & Jaeger, P. (1982). Spectral shifts of ultrasonic propagation through media with nonlinear dispersive attenuation. Ultrasonic Imaging, 4, 282–289.

    Google Scholar 

  18. Obberhettinger, F. (1961). On transient solution of the baffled piston problem. Journal of Research of the National Bureau of Standards, 65-B, 1–6.

    Google Scholar 

  19. Stepanishen, P. R. (1971). Transient radiation from piston in an infinite planar baffle. The Journal of the Acoustical Society of America, 49, 1629–1638.

    Article  Google Scholar 

  20. Lewis, G. K. (1980). A matrix technique for analyzing the performance of multilayer front matched and backed piezoelectric ceramic transducers. Acoustical Imaging, 8, 395.

    Article  Google Scholar 

  21. Ali, M. G. S. (1999). Discrete time model of acoustic waves transmitted through layers. Journal of Sound and Vibration, 224(2), 349.

    Article  Google Scholar 

  22. Matheson, J. (1971). Molecular acoustics. London: Wiley-Interscience.

    Google Scholar 

  23. Pauly, H., & Schwan, H. P. (1971). Mechanism of absorption of ultrasound in liver tissue. The Journal of the Acoustical Society of America, 50, 692–698.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, Zagazig University, P.O. Box 44519, Zagazig, Egypt

    R. M. Farouk

  2. Physics Department, Faculty of Science, Minia University, Minia, Egypt

    M. G. S. Ali

Authors
  1. R. M. Farouk
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. M. G. S. Ali
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to R. M. Farouk.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Farouk, R.M., Ali, M.G.S. Ultrasonic digital signal processing simulation in viscoelastic medium with generalized parametric function. Telecommun Syst 52, 1449–1456 (2013). https://doi.org/10.1007/s11235-011-9622-1

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11235-011-9622-1

Keywords