Skip to main content

Advertisement

Springer Nature Link
Log in

A unified framework for performance evaluation over generalized fading channels

  • Published:
Telecommunication Systems Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

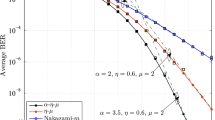
In this paper, we present a unified framework to analyze the performance of the average bit error probability (BEP) and the outage probability over generalized fading channels. Specifically, we assume that the probability density function (PDF) of the instantaneous signal-to-noise ratio \(\zeta \) is given by the product of: power function, exponential function, and the modified Bessel function of the first kind, i.e., \(f_{\zeta }(\zeta )=\zeta ^{\lambda -1}exp\left( -a\zeta ^{\beta }\right) I_{v}\left( b\zeta ^{\beta }\right) \). Based on this PDF, we obtain a novel closed-form expression for the average BEP over such channels perturbed by an additive white generalized Gaussian noise (AWGGN). Note that other well-known noise types can be deduced from the AWGGN as special cases such as Gaussian noise, Laplacian noise, and impulsive noise. Furthermore, we obtain a novel closed-form expression for the outage probability. As an example of such channels, and without loss of generality, we analyze the performance of the average BEP and the outage probability over the \(\eta \)–\(\mu \) fading channels. Analytical results accompanied with Monte-Carlo simulations are provided to validate our analysis.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Notes

  1. Note that the BFHF in (15) converges if the following conditions are satisfied [23]: \(\rho _{1}=\sum _{j=1}^{p_{1}}\alpha _{j}+\sum _{j=1}^{p_{2}}\gamma _{j}-\sum _{j=1}^{q_{1}}\beta _{j}-\sum _{j=1}^{q_{2}}\delta _{j}\le 0, \rho _{2}=\sum _{j=1}^{p_{1}}A_{j}+\sum _{j=1}^{p_{2}}E_{j}-\sum _{j=1}^{q_{1}}B_{j}-\sum _{j=1}^{q_{2}}F_{j}\le 0, \Omega _{1}=-\sum _{j=n_{1}+1}^{p_{1}}\alpha _{j}-\sum _{j=1}^{q_{1}}\beta _{j}+\sum _{j=1}^{m_{2}}\delta _{j}-\sum _{j=m_{2}+1}^{p_{2}}\delta _{j}+\sum _{j=1}^{n_{2}}\gamma _{j}-\sum _{n_{2}+1}^{p_{2}}\gamma _{j}>0, \Omega _{2}=-\sum _{j=n_{1}+1}^{p_{1}}A_{j}-\sum _{j=1}^{q_{1}}B_{j}+\) \(\sum _{j=1}^{m_{3}}F_{j}-\sum _{j=m_{3}+1}^{p_{3}}F_{j}+\sum _{j=1}^{n_{3}}E_{j}-\sum _{n_{3}+1}^{p_{3}}E_{j}>0, |\mathrm{arg}~x|<\pi \Omega _{1}/2; \mathrm{and}~|\mathrm{arg}~y|<\pi \Omega _{2}/2\). It is straightforward to show that the parameters of the BFHF in (15) satisfies these sufficient conditions, and therefore the BFHF converges.

References

  1. Kassam, S. A. (1988). Signal detection in non-gaussian noise. Berlin: Springer.

    Book  Google Scholar 

  2. Chapeau-Blondeau, F. (2000). Nonlinear test statistic to improve signal detection in non-Gaussian noise. IEEE Signal Processing Letters, 7(7), 205–207.

    Article  Google Scholar 

  3. Soury, H., Yilmaz, F., & Alouini, M.-S. (2012). Average bit error probability of binary coherent signaling over generalized fading channels subject to additive generalized Gaussian noise. IEEE Communications Letters, 16(6), 785–788.

    Article  Google Scholar 

  4. Soury, H., Yilmaz, F., & Alouini, M. S. (2013). Error rates of \(M\)-PAM and \(M\)-QAM in generalized fading and generalized Gaussian noise environments. IEEE Communications Letters, 17(10), 1932–1935.

    Article  Google Scholar 

  5. Soury, H. & Alouini, M.-S. (2014). On the symbol error rate of \(M\)-ary MPSK over generalized fading channels with additive Laplacian noise. In Proceedings of the IEEE ISIT (pp. 2879–2883).

  6. Hu, B. & Beaulieu, N. C. (2008). On characterizing multiple access interference in TH-UWB systems with impulsive noise model. In Proceedings of the IEEE radio wireless symposium (pp. 879–882).

  7. Fiorina, J. (2006). A simple IR-UWB receiver adapted to multi-user interferences. In Proceedings of the IEEE Globecom (pp. 1–4).

  8. Dhibi, Y., & Kaiser, T. (2006). On the impulsiveness of multiuser interferences in Th-ppm-uwb systems. IEEE Transactions on Signal Processing, 54(7), 2853–2857.

    Article  Google Scholar 

  9. Thompson, M. W., & Chang, H. S. (1994). Coherent detection in Laplace noise. IEEE Transactions on Aerospace and Electronic Systems, 30(2), 452–461.

    Article  Google Scholar 

  10. Li T.H. & Song, K. S. (1990). Estimation of the frequency of sinusoidal signals in Laplace noise. In Proceedings of the IEEE international symposium on information theory (pp. 1786–1790).

  11. Helstrom, C. W. (1989). Detectability of signals in Laplace noise. IEEE Transactions on Aerospace and Electronic Systems, 25(2), 190–196.

  12. Marks, R. J., Wise, G. L., Haldeman, D. G., & Whited, J. L. (1978). Detection in Laplace noise. IEEE Transactions on Aerospace and Electronic Systems, AES–14(6), 866–871.

    Article  Google Scholar 

  13. Simon, M. K., & Alouini, M. (2005). Digital communications over fading channels. New York: Wiley.

    Google Scholar 

  14. Ansari, I.S., Yilmaz, F., Alouini, M.-S. & Kucur, O. (2014). New results on the sum of gamma random variates with application to the performance of wireless communication systems over nakagami-m fading channels. Transactions on Emerging Telecommunications Technologies. doi:10.1002/ett.2912.

  15. Efthymoglou, G., Ermolova, N., & Aalo, V. (2010). Channel capacity and average error rates in generalised-\({K}\) fading channels. IET Communications, 4(11), 1364–1372.

    Article  Google Scholar 

  16. Ermolova, N. Y. & Tirkkonen, O. (2013). Performance analysis of communication systems over generalized \(\alpha \)-\(\lambda \)-\(\eta \)-\(\mu \) fading radio channels. In Proceedings of the 77th IEEE Vehicular Technology VTC (pp. 1–5).

  17. da Costa, D. B., & Yacoub, M. D. (2008). Moment generating functions of generalized fading distributions and applications. IEEE Communications Letters, 12(2), 112–114.

    Article  Google Scholar 

  18. Badarneh, O. S. (2015). Error rate analysis of \(m\)-ary phase shift keying in \(\alpha \)-\(\eta \)-\(\mu \) fading channels subject to additive Laplacian noise. IEEE Communications Letters, 19(7), 1253–1256.

    Article  Google Scholar 

  19. Salahat, E. & Hakam, A. (2014). Novel unified expressions for error rates and ergodic channel capacity analysis over generalized fading subject to AWGGN. In Proceedings of the IEEE GlobeCom (pp. 3976–3982).

  20. Salahat, E. & Saleh, H. (2014). Novel average bit error rate analysis of generalized fading channels subject to additive white generalized Gaussian noise. In Proceedings of the GlobalSIP (pp. 1107–1111).

  21. Papazafeiropoulos, A. K. & Kotsopoulos, S. A. (2009). The \(\alpha \)-\(\lambda \)-\(\mu \)-\(\eta \): A generalized fading distribution In Procedings of the IEEE WCNC (pp. 404–409).

  22. Gradshteyn, I. S., & Ryzhik, I. M. (2007). Table of integrals, series, and products (7th ed.). California: Academic Press.

    Google Scholar 

  23. Mathai, A., Saxena, R., & Haubold, H. J. (2010). The H-function theory applications. New York: Springer.

    Google Scholar 

  24. Chaudhry, M. A., & Zubair, S. M. (2002). On a class of incomplete gamma function with applications. London: Chapman & Hall and CRC.

    Google Scholar 

  25. Prudnikov, A. P., Brychkov, Y. A., & Marichev, O. I. (1990). Integrals, and series: More special functions (3rd ed.). New York: Gordon & Breach Science.

    Google Scholar 

  26. Kilbas, A., & Saigo, M. (2004). H-transforms : Theory and applications (analytical method and special function) (1st ed.). London: CRC Press.

    Book  Google Scholar 

  27. Prudnikov, A. P., Brychkov, Y. A., & Marichev, O. I. (1990). Integrals, and series: More special functions (1st ed.). New York: Gordon & Breach Science.

    Google Scholar 

  28. Yacoub, M. D. (2007). The \(\kappa \)-\(\mu \) distribution and the \(\eta \)-\(\mu \) distribution. IEEE Antennas and Propagation Magazine, 49(1), 68–81.

    Article  Google Scholar 

  29. Morales-Jiménez, D., & Paris, J. F. (2010). Outage probability analysis for \(\eta \)-\(\mu \) fading channels. IEEE Communication Letters, 14(6), 521–523.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Electrical Engineering Department, Faculty of Engineering, University of Tabuk, P.O.Box:741, 71491, Tabuk, Saudi Arabia

    Osamah S. Badarneh & Fares S. Almehmadi

  2. Electrical Engineering Department, Jordan University of Science and Technology, Irbid, 22110, Jordan

    Taimour Aldalgamouni

Authors
  1. Osamah S. Badarneh
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Taimour Aldalgamouni
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Fares S. Almehmadi
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Osamah S. Badarneh.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Badarneh, O.S., Aldalgamouni, T. & Almehmadi, F.S. A unified framework for performance evaluation over generalized fading channels. Telecommun Syst 64, 669–678 (2017). https://doi.org/10.1007/s11235-016-0199-6

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11235-016-0199-6

Keywords