Joint impacts of hardware impairments, imperfect CSIs, and interference constraints on underlay cooperative cognitive networks with reactive relay selection

Abstract

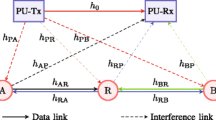
In this paper, we investigate joint impacts of hardware impairments, imperfect channel state information (CSI) and interference constraints at secondary users in an underlay decode-and-forward cognitive network with multiple primary receivers, called as JIC protocol. A best secondary relay is derived from the reactive relay selection which maximizes signal-to-interference-and-distortion-and-noise ratios from a candidates relay set to a secondary destination over Rayleigh fading channels. The exact and asymptotic closed-form outage probability expressions are obtained to evaluate the system performance of the JIC protocol, and then are verified by the Monte Carlo simulations. Contributions show performance degradation of the JIC protocol due to the hardware impairments, the imperfect CSIs, and the interference constraints, and the significant performance improvement with respect to optimal impairment levels and optimal relay locations as well as the increase in the number of the secondary relays. In addition, insightful discussions with the conventional direct transmission are provided. Finally, the exact and asymptotic closed-form expressions are valid to the simulation results.

Appendices

Appendices

1.1 Appendix A: Proof of Lemma 1

When $\left( {1 - \theta {K^2}} \right) > 0$ or $\theta < {K^{ - 2}}$, $\Pr \left[ {\left. D \right| \theta < {K^{ - 2}}} \right]$ is changed as

$$\begin{aligned}&\Pr \left[ {\left. D \right| \theta< {K^{ - 2}}} \right] \nonumber \\&\quad = \Pr \left[ {\underbrace{\,\mathop {\max }\limits _{u = n + 1,n + 2,\ldots ,N} {g_{SS,S{R_u}}}}_U } \right. \nonumber \\&\qquad \left. < {\underbrace{\upsilon \left( {\theta ,{\lambda _1}} \right) + \tau \left( \theta \right) {\psi _{SS}}}_\Phi \le \underbrace{\mathop {\min }\limits _{v = 1,2,\ldots ,n} {g_{SS,S{R_v}}}}_V} \right] \nonumber \\&\quad = \int \limits _{\upsilon \left( {\theta ,{\lambda _1}} \right) }^\infty {{f_\Phi }\left( x \right) \times {F_U}\left( x \right) \times \left\{ {1 - {F_V}\left( x \right) } \right\} dx} \end{aligned}$$

(A.1)

where ${f_\Phi }\left( x \right)$ is the pdf of the RV $\Phi$, and ${F_U}\left( x \right)$ and ${F_V}\left( x \right)$ are the CDFs of the RVs U and V, respectively.

The CDF ${F_U}\left( x \right)$ is manipulated and obtained as

$$\begin{aligned}&{F_U}(x) \nonumber \\&\quad = \Pr \left[ {U< x} \right] = \Pr \left[ {\mathop {\max }\limits _{u = n + 1,n + 2,\ldots ,N} {g_{SS,S{R_u}}}< x} \right] \nonumber \\&\quad = \prod \limits _{u = n + 1}^N {\Pr \left[ {{g_{SS,S{R_u}}} < x} \right] } = \prod \limits _{u = n + 1}^N {{F_{{g_{SS,S{R_u}}}}}\left( x \right) } \nonumber \\&\quad = {\left( {1 - {e^{{{ - x} / {{\lambda _{sr}}}}}}} \right) ^{N - n}} = \sum \limits _{p = 0}^{N - n} {\left( {\begin{array}{*{20}{c}} p\\ {N - n} \end{array}} \right) {{\left( { - 1} \right) }^p} \times {e^{{{ - px} / {{\lambda _{sr}}}}}}} \end{aligned}$$

(A.2)

Similarly, the CDF ${F_V}\left( x \right)$ is also obtained as

$$\begin{aligned}&{F_V}(x)\nonumber \\&\quad = \Pr \left[ {V< x} \right] = \Pr \left[ {\mathop {\min }\limits _{v = 1,2,\ldots ,n} {g_{SS,S{R_v}}}< x} \right] \nonumber \\&\quad = 1 - \Pr \left[ {\mathop {\min }\limits _{v = 1,2,\ldots ,n} {g_{SS,S{R_v}}} \ge x} \right] \nonumber \\&\quad = 1 - \prod \limits _{v = 1}^n {\Pr \left[ {{g_{SS,S{R_v}}} \ge x} \right] } \nonumber \\&\quad = 1 - \prod \limits _{v = 1}^n {\left\{ {1 - \Pr \left[ {{g_{SS,S{R_v}}} < x} \right] } \right\} } \nonumber \\&\quad = 1 - \prod \limits _{v = 1}^n {\left\{ {1 - {F_{{g_{SS,S{R_v}}}}}\left( x \right) } \right\} } = 1 - {e^{{{ - nx} / {{\lambda _{sr}}}}}} \end{aligned}$$

(A.3)

To have ${f_\Phi }\left( x \right)$ in (A.1), firstly, the CDF of the RV $\Phi$ is solved as

$$\begin{aligned}&{F_\Phi }(x)\nonumber \\&\quad = \Pr \left[ {\upsilon \left( {\theta ,{\lambda _{sr}}} \right) + \tau \left( \theta \right) {\psi _{SS}}< x} \right] \nonumber \\&\quad = \Pr \left[ {\upsilon \left( {\theta ,{\lambda _{sr}}} \right) + \tau \left( \theta \right) \mathop {\max }\limits _{k = 1,2,\ldots ,M} {g_{SS,P{R_k}}}< x} \right] \,\nonumber \\&\quad = \Pr \left[ {\mathop {\max }\limits _{k = 1,2,\ldots ,M} {g_{SS,P{R_k}}}< \frac{{x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right) }}{{\tau \left( \theta \right) }}} \right] \nonumber \\&\quad = \left\{ \begin{array}{ll} 0,&{}\quad x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \le 0\\ \prod \limits _{k = 1}^M {\Pr \left[ {{g_{SS,P{R_k}}} < \frac{{x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right) }}{{\tau \left( \theta \right) }}} \right] } ,&{}\quad x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right)> 0 \end{array} \right. \nonumber \\&\quad = \left\{ \begin{array}{ll} 0,&{}\quad x \le \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \\ \prod \limits _{k = 1}^M {{F_{{g_{SS,P{R_k}}}}}\left( {\frac{{x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right) }}{{\tau \left( \theta \right) }}} \right) } ,&{}\quad x> \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \end{array} \right. \nonumber \\&\quad = \left\{ \begin{array}{ll} 0,&{}\quad x \le \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \\ {\left( {1 - {e^{ - \frac{{x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right) }}{{\tau \left( \theta \right) {\lambda _{sp}}}}}}} \right) ^M},&{}\quad x> \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \end{array} \right. \nonumber \\&\quad = \left\{ \begin{array}{ll} 0,&{}\quad x \le \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \\ \sum \limits _{q = 0}^M {\left( {\begin{array}{*{20}{c}} q\\ M \end{array}} \right) \times {{\left( { - 1} \right) }^q} \times {e^{ - \frac{{\left( {x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right) } \right) q}}{{\tau \left( \theta \right) {\lambda _{sp}}}}}}} ,&\quad x > \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \end{array} \right. \end{aligned}$$

(A.4)

where $\left( {\begin{array}{*{20}{c}} a\\ b \end{array}} \right)$ is a binomial coefficient $\left( {\left( {\begin{array}{*{20}{c}} a\\ b \end{array}} \right) = \frac{{b!}}{{a! \times \left( {b - a} \right) !}}} \right)$.

Hence, the pdf ${f_\Phi }\left( x \right)$ is derived as

$$\begin{aligned}&{f_\Phi }\left( x \right) = \frac{{\partial {F_\Phi }(x)}}{{\partial x}} = \nonumber \\&\quad \left\{ \begin{array}{ll} 0\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,,\,\,x \le \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \\ \frac{1}{{\tau \left( \theta \right) {\lambda _{sp}}}} \times \sum \limits _{q = 1}^M {\left( {\begin{array}{*{20}{c}} q\\ M \end{array}} \right) \times {{\left( { - 1} \right) }^{q + 1}}} \\ \times q \times {e^{ - \frac{{\left( {x - \upsilon \left( {\theta ,{\lambda _{sr}}} \right) } \right) q}}{{\tau \left( \theta \right) {\lambda _{sp}}}}}},x > \upsilon \left( {\theta ,{\lambda _{sr}}} \right) \end{array} \right. \end{aligned}$$

(A.5)

Substituting (A.2), (A.3) and (A.5) into (A.1), we obtain as

$$\begin{aligned}&\Pr \left[ {\left. D \right| \theta < {K^{ - 2}}} \right] \nonumber \\&\quad = \frac{1}{{\tau \left( \theta \right) {\lambda _{sp}}}}\sum \limits _{p = 0}^{N - n} {\sum \limits _{q = 1}^M {\left( {\begin{array}{*{20}{c}} p\\ {N - n} \end{array}} \right) \left( {\begin{array}{*{20}{c}} q\\ M \end{array}} \right) } } \nonumber \\&\qquad \times {\left( { - 1} \right) ^{p + q + 1}} \times q \times {e^{{{\upsilon \left( {\theta ,{\lambda _{sr}}} \right) q} / {\left( {\tau \left( \theta \right) {\lambda _{sp}}} \right) }}}}\nonumber \\&\qquad \times \int \limits _{\upsilon \left( {\theta ,{\lambda _{sr}}} \right) }^\infty {{e^{{{ - nx} / {{\lambda _{sr}}}} - {{px} / {{\lambda _{sr}}}}{{ - qx} / {\left( {\tau \left( \theta \right) {\lambda _{sp}}} \right) }}}}dx} \end{aligned}$$

(A.6)

Solving (A.6), Lemma 1 is proven.

1.2 Appendix B: Proof of Lemma 2

The CDF ${F_{{\gamma _{X,Z}}}}\left( {\left. x \right| x < {K^{ - 2}}} \right)$ of the RV ${\gamma _{X,Z}}$ is expressed and manipulated at the top of next page, where ${f_{{\psi _X}}}\left( y \right)$ is the pdf of the RV ${\psi _X}$, and similarly as in (A.4) and (A.5) in “Appendix A”, ${f_{{\psi _X}}}\left( y \right)$ is obtained from the formula of ${\psi _X}$ in (5) as

$$\begin{aligned}&{F_{{\gamma _{X,Z}}}}\left( {\left. x \right| x< {K^{ - 2}}} \right) \nonumber \\&\quad = \Pr \left[ {\left. {{\gamma _{X,Z}}< x} \right| x< {K^{ - 2}}} \right] \nonumber \\&\quad = \Pr \left[ {\left. {\frac{{Q{g_{X,Z}}}}{{{K^2}Q{g_{X,Z}} + Q\left( {1 + {K^2}} \right) \left( {1 - {\rho ^2}} \right) {\lambda _{X,Z}} + {\rho ^2}\left( {1 + K_t^2} \right) {\psi _X}}}< x} \right| }\right. \nonumber \\&\qquad \left. {x< {K^{ - 2}}} \right] \nonumber \\&\quad = \Pr \left[ \left. Q{g_{X,Z}}\left( {1 - x{K^2}} \right)< xQ\left( {1 + {K^2}} \right) \left( {1 - {\rho ^2}} \right) {\lambda _{X,Z}} \right. \right. \nonumber \\&\qquad \left. \left. + x{\rho ^2}\left( {1 + K_t^2} \right) {\psi _X} \right| x< {K^{ - 2}} \right] \nonumber \\&\quad = \Pr \left[ {\left. {{g_{X,Z}}< \upsilon \left( {x,{\lambda _{X,Z}}} \right) + \tau \left( x \right) \times {\psi _X}} \right| x< {K^{ - 2}}} \right] \nonumber \\&\quad = \int \limits _0^\infty {{f_{{\psi _X}}}\left( y \right) \times {F_{{g_{X,Z}}}}\left( {\left. {\upsilon \left( {x,{\lambda _{X,Z}}} \right) + \tau \left( x \right) \times y} \right| x < {K^{ - 2}}} \right) dy} \end{aligned}$$

(B.1)

$$\begin{aligned}&{f_{{\psi _X}}}\left( y \right) \nonumber \\&\quad = \frac{{\partial {F_{{\psi _X}}}\left( y \right) }}{{\partial y}} = \frac{{\partial \Pr \left[ {{\psi _X}< y} \right] }}{{\partial y}}\nonumber \\&\quad = \frac{{\partial \Pr \left[ {\mathop {\max }\limits _{k = 1,2,\ldots ,M} {g_{X,P{R_k}}}< y} \right] }}{{\partial y}} \nonumber \\&\quad = \frac{{\partial \prod \limits _{k = 1}^M {\Pr \left[ {{g_{X,P{R_k}}} < y} \right] } }}{{\partial y}}\nonumber \\&\quad = \frac{{\partial \prod \limits _{k = 1}^M {{F_{{g_{X,P{R_k}}}}}\left( y \right) } }}{{\partial y}} = \frac{{\partial {{\left( {1 - {e^{ - {y \big / {{\lambda _{X,P{R_k}}}}}}}} \right) }^M}}}{{\partial y}}\nonumber \\&\quad = \frac{1}{{{\lambda _{X,P{R_k}}}}} \times \sum \limits _{t = 1}^M {\left( {\begin{array}{*{20}{c}} t\\ M \end{array}} \right) \times {{\left( { - 1} \right) }^{t + 1}} \times t \times {e^{ - {{ty} \big / {{\lambda _{X,P{R_k}}}}}}}} \end{aligned}$$

(B.2)

Substituting (B.2) into (B.1), ${F_{{\gamma _{X,Z}}}}\left( {\left. x \right| x < {K^{ - 2}}} \right)$ is obtained as

$$\begin{aligned}&{F_{{\gamma _{X,Z}}}}\left( {\left. x \right| x < {K^{ - 2}}} \right) \nonumber \\&\quad = \int \limits _0^\infty {{f_{{\psi _X}}}\left( y \right) \times \left\{ {1 - {e^{{{ - \left( {\upsilon \left( {x,{\lambda _{X,Z}}} \right) + \tau \left( x \right) \times y} \right) } / {{\lambda _{X,Z}}}}}}} \right\} dy} \nonumber \\&\quad = 1 - \frac{{{e^{{{ - \upsilon \left( {x,{\lambda _{X,Z}}} \right) } / {{\lambda _{X,Z}}}}}}}}{{{\lambda _{X,P{R_k}}}}}\sum \limits _{t = 1}^M {\left( {\begin{array}{*{20}{c}} t\\ M \end{array}} \right) \times {{\left( { - 1} \right) }^{t + 1}} \times t} \nonumber \\&\qquad \times \int \limits _0^\infty {{e^{ - {{ty} / {{\lambda _{X,P{R_k}}}}}{{ - \tau \left( x \right) y} / {{\lambda _{X,Z}}}}}}dy} \end{aligned}$$

(B.3)

By solving (B.3), Lemma 2 is proven.