Skip to main content

Advertisement

Springer Nature Link
Log in

Temporal Scale Spaces

  • Published:
International Journal of Computer Vision Aims and scope Submit manuscript

Abstract

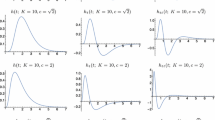
In this paper we discuss how to define a scale space suitable for temporal measurements. We argue that such a temporal scale space should possess the properties of: temporal causality, linearity, continuity, positivity, recursitivity as well as translational and scaling covariance. It is shown that these requirements imply a one parameter family of convolution kernels. Furthermore it is shown that these measurements can be realized in a time recursive way, with the current data as input and the temporal scale space as state, i.e. there is no need for storing earlier input. This family of measurement processes contains the diffusion equation on the half line (that represents the temporal scale) with the input signal as boundary condition on the temporal axis. The diffusion equation is unique among the measurement processes in the sense that it is preserves positivity (in the scale domain) and is locally generated. A numerical scheme is developed and relations to other approaches are discussed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  • Aczél, J. and Dhombres, J. (1989). Functional Equations in Several Variables. Encyclopaedia of Mathematics and its Applications. Cambridge University Press.

  • Feller, W. (1966). An Introduction to Probability Theory and its Application, vol. 2. John Willey & Sons, Inc.

  • Florack, L.M.J. (1997). Image Structure. Series in Mathematical Imaging and Vision. Kluwer Academic Publishers, Dordrecht, Netherlands.

    Google Scholar 

  • Hille, E. and Phillips, R.S. (1957). Functional Analysis and Semi-Groups. American Mathematical Society.

  • Koenderink, J.J. (1988). Scale-time. Biological Cybernetics 58:169–162.

    Google Scholar 

  • Koenderink, J.J., Kappers, A., and van Doorn, A.J. (1992). Local operations: The embodiment of geometry. Artificial and Biological Vision Systems, Basic Research Series, Orban, G.A. and Nagel, H.H. (Eds.), Springer Verlag, pp. 1–23.

  • Lindeberg, T. and Fagerström, D. (1996). Scale-space with causal time direction. In Proc. 4th European Conference on Computer Vision, vol. 1064, Cambridge, UK, Springer Verlag, Berlin, pp. 229–240.

  • Pauwels, E.J., VanGool, L.J., Fiddelaers, P., and Moons, T. (1995). An extended class of scale-invariant and recursive scale space filters. PAMI 17(7):691–701.

    Google Scholar 

  • Podlubny, I. (1999). Fractional Differential Equations. Academic Press.

  • Richtmyer, R.D. and Morton, K.W. (1967). Difference Methods for Initial-Value Problems, 2nd edition. Interscience Publishers.

  • Salden, A.H., Haar Romeny, B.M. ter and Viergever, M.A. (1998). Linear scale-space theory from physical principles. JMIV, 9(2):103–139.

    Article  Google Scholar 

  • Samko, S.G., Kilbas, A.A., and Marichev, O.I. (1992). Fractional Integrals and Derivatives : Theory and Applications. Gordon and Breach Science Publishers, cop., Yverdon.

  • Saulyev, V.K. (1964). Integration of Equatioons of Parabolic Type by the Method of Nets. Pergamon Press.

  • Treves, F. (1967). Topological Vector Spaces, Distributions and Kernels. Academic Press.

  • Weickert, J., Ishikawa, S., Imiya, A. (1997). On the history of gaussian scale-space axiomatics. Gaussian Scale-Space Theory, 45–59.

Download references

Author information

Authors and Affiliations

  1. Computational Vision and Active Perception Laboratory (CVAP), Department of Numerical Analysis and Computing Science, KTH (Royal Institute of Technology), SE-100 44, Stockholm, Sweden

    Daniel Fagerström

Authors
  1. Daniel Fagerström
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Daniel Fagerström.

Additional information

First online version published in June, 2005

Rights and permissions

Reprints and permissions

About this article

Cite this article

Fagerström, D. Temporal Scale Spaces. Int J Comput Vision 64, 97–106 (2005). https://doi.org/10.1007/s11263-005-1837-8

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11263-005-1837-8

Keywords