Optimal simultaneous wireless information and energy transfer in OFDMA decode-and-forward relay networks

Abstract

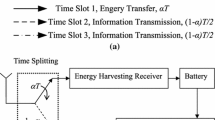
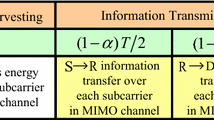
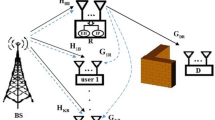
In this paper, we consider simultaneous wireless information and energy transfer in an orthogonal-frequency-division-multiple-access decode-and-forward relay network, in which an energy-constrained relay node harvests energy from a source node and uses the harvested energy to forward information to multiple destination nodes. Our objective is to maximize the end-to-end sum rate by resource allocation, subject to transmit power constraint at the source and energy-harvesting (EH) constraint at the relay. A non-convex and mixed-integer programming (MIP) problem is formulated to optimize time-switching (TS) ratios of EH and information decoding at the relay, TS ratio of information transmission from relay to destinations, subcarrier allocation as well as power allocation (PA) over all subcarriers at source and relay. We propose to decouple this problem into a convex problem and an MIP problem in fractional form. To solve the MIP problem, we transform it into an equivalent optimization problem in subtractive form which has a tractable solution. As a result, we propose a novel scheme to achieve jointly optimal TS ratios, subcarrier allocation and PA. Simulation results verify the optimality of our proposed resource allocation scheme.

Appendices

Appendix 1

Proof of Proposition 2

By introducing a slack variable $\theta$, we firstly rewrite problem (20) equivalently as

$$\begin{aligned}&\min : \; \theta \nonumber \\&\quad \mathrm{s.t.} \quad \nonumber \\&\quad \left[ \begin{array}{cc} \max \limits _{\mathbf {P}^{\mathrm{R}},\Phi } &{}\frac{G\sum _{k=1}^K \sum _{n=1}^N\phi _{k,n}^{\mathrm{RD}} \log \left( 1+P^{\mathrm{R}}_{k,n} \gamma ^{\mathrm{RD}}_{k,n}\right) }{\sum _{k=1}^K\sum _{n=1}^{N}P^{\mathrm{R}}_{k,n}+G}\le \theta \\ \quad \mathrm{s.t.} &{}P^{\mathrm{R}}_{k,n}\ge 0, \forall k\in {\mathcal {K}},n\in {\mathcal {N}}, \\ &{}\sum _{k=1}^K \phi _{k,n}^{\mathrm{RD}} = 1, \; \forall n \in {\mathcal {N}}, \\ &{}\phi _{k,n}^{\mathrm{RD}} \in \{0,1\}, \forall k\in {\mathcal {K}},n\in {\mathcal {N}}. \end{array} \right] \end{aligned}$$

(36)

This holds since minimizing $\theta$ is the same as finding the least upper bound of the objective function of problem (20), which is equal to the maximum value of objective function [23]. The problem (36) is further recast as [23]

$$\begin{aligned}&\min : \; \theta \nonumber \\&\quad \mathrm{s.t.} \nonumber \\&\quad \left[ \begin{array}{cc} \min \limits _{\mathbf {P}^{\mathrm{R}},\Phi } &{}F\left( \mathbf {P}^{\mathrm{R}},\Phi ,\theta \right) \ge 0 \qquad \qquad \quad \\ \quad \mathrm{s.t.} &{}P^{\mathrm{R}}_{k,n}\ge 0, \forall k\in {\mathcal {K}},n\in {\mathcal {N}}, \\ &{}\sum _{k=1}^K \phi _{k,n}^{\mathrm{RD}} = 1, \; \forall n \in {\mathcal {N}}, \quad \\ &{}\phi _{k,n}^{\mathrm{RD}} \in \{0,1\}, \forall k\in {\mathcal {K}},n\in {\mathcal {N}} \end{array}\right] \end{aligned}$$

(37)

where $F\left( \mathbf {P}^{\mathrm{R}},\Phi ,\theta \right)$ is defined in (22). This holds since $\sum _{k=1}^K\sum _{n=1}^{N}P^{\mathrm{R}}_{k,n}+G$ is strictly positive. Then, $\theta$ is a true upper bound if the problem

$$\begin{aligned} \min \limits _{\mathbf {P}^{\mathrm{R}},\Phi } \;&F\left( \mathbf {P}^{\mathrm{R}},\Phi ,\theta \right) \\ \mathrm{s.t.} \;&P^{\mathrm{R}}_{k,n}\ge 0, \forall k\in {\mathcal {K}}, n\in {\mathcal {N}}, \\&\sum _{k=1}^K \phi _{k,n}^{\mathrm{RD}} = 1, \; \forall n \in {\mathcal {N}}, \\&\phi _{k,n}^{\mathrm{RD}} \in \{0,1\}, \forall k\in {\mathcal {K}},n\in {\mathcal {N}} \end{aligned}$$

has a non-negative optimal value. Specially, if and only if $\theta =\overline{\theta }$, where $\overline{\theta }$ is the solution of the equation $T(\overline{\theta })=0$, in which $T(\theta )$ is defined in (23), the optimal value of problem (20) is $\overline{\theta }$, and $\left( \overline{\mathbf {P}}^{\mathrm{R}}, \overline{\Phi }\right) = \arg \max _{\mathbf {P}^{\mathrm{R}},\Phi } F\left( \mathbf {P}^{\mathrm{R}},\Phi ,\overline{\theta }\right)$ is the optimal solution to problem (20). The proof is completed. □

Appendix 2

Proof of Proposition 3

Introduce a variable

$$ \varphi =\frac{G}{\sum _{k=1}^{K}\sum _{n=1}^{N}P_{k,n}^{\mathrm{R}}+G},$$

(38)

Then $\varphi \sum _{k=1}^{K} \sum _{n=1}^{N}P_{k,n}^{\mathrm{R}}=(1-\varphi ) G$. Since $0\le \varphi \le 1$, it follows

$$ P_{k,n}^{\mathrm{R}}\le \sum _{k=1}^{K} \sum _{n=1}^{N}P_{k,n}^{\mathrm{R}} =\frac{1-\varphi }{\varphi }G \le \frac{1+\varphi }{\varphi }G. $$

(39)

In addition, according to (38), the objective function of problem (20) can be rewritten as

$$\hat{R}=\varphi \sum _{k=1}^K \sum _{n=1}^N\phi _{k,n}^{\mathrm{RD}} \log \left( 1+P^{\mathrm{R}}_{k,n} \gamma ^{\mathrm{RD}}_{k,n}\right)$$

(40)

It is obvious that allowing all destinations to receive data information over all subcarriers, which results in $\phi _{k,n}^{\mathrm{RD}}=1$ for all $k\in K$ and $n \in N$, will increase the end-to-end achievable sum rate. Then we have

$$\hat{R}\le \varphi \sum _{k=1}^K\sum _{n=1}^N \log \left( 1+P^{\mathrm{R}}_{k,n} \gamma ^{\mathrm{RD}}_{k,n}\right) \le KN \varphi \log \left( 1+S+S/\varphi \right).$$

(41)

with $S=G \max _{k,n}\left\{ \gamma ^{\mathrm{RD}}_{k,n}\right\}$. Since $1+S+S/\varphi \le \left( 1+S\right) \left( 1+1/\varphi \right)$ and $\varphi \le 1$, we have

$$\begin{aligned} \hat{R}&\le KN \varphi \log \left( 1+S\right) +N \varphi \log \left( 1+1/\varphi \right) \nonumber \\&\le KN\log \left( 1+S\right) +KN \varphi \log \left( 1+1/\varphi \right) . \end{aligned}$$

(42)

It can be verified that $f(\varphi )=\varphi \log \left( 1+1/\varphi \right)$ is concave. The maximum value of $f(\varphi )$ is obtained by letting $df(\varphi )/d\varphi =0$. Thus, we have $\exp (1/(1+\varphi ))=(1+\varphi )/\varphi$. Using the Lambert ${\mathcal {W}}$ Function [24], the solution to the problem $\max _{\varphi }f(\varphi )$ is $\varphi =\vartheta ={\mathcal {W}}\left( -\frac{1}{e}\right)$.□