Skip to main content

Advertisement

Springer Nature Link
Log in

A Generalized Modified Suzuki Model with Sectored and Inhomogeneous Diffuse Scattering Component

  • Published:
Wireless Personal Communications Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

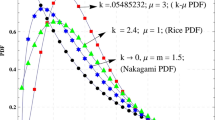
This paper presents a mixture stochastic process by multiplying a Weibull process with a lognormal one. The first one models the possible scattering non-uniformities of the channel, whereas the second accounts for the slow term variations of the local mean due to shadowing. Moreover we incorporate sectored arrival of multipath power (anisotropic scattering) via a correlation scheme between the zero mean Gaussian processes, generating the Rayleigh part of the Weibull process. An exact solution for the mixture probability density function (PDF) will be given, together with approximate solutions for the second order statistics, level crossing rate (LCR) and average duration of fades (ADF’s). The validity of the approximate solutions will be tested via an efficient simulation scheme, which implements the analytical model on a digital computer. Finally a curve fitting of the LCR to real world data, drawn from channel measurements, will demonstrate the flexibility and usefulness of the proposed model.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Braun W.R., Dersch U. (1991). A physical mobile radio channel model. IEEE Transactions on Vehicular Technology 40(2): 472–482

    Article  Google Scholar 

  2. Krantzik, A., & Wolf, D. (1990). Distribution of the fading-intervals of modified suzuki processes. In L. Torres, E. Masgrau, & M. A. Lagunas (Eds.), Signal processing V: Theories and applications (pp. 361–364). Amsterdam, The Netherlands: Elsevier.

  3. Suzuki H. (1977). A statistical model for urban radio propagation. IEEE Transactions on Communications 25(7): 673–680

    Article  Google Scholar 

  4. Hansen F., Meno F.I. (1977). Mobile fading-Rayleigh and lognormal superimposed. IEEE Transactions on Vehicular Technology 26(4): 332–335

    Article  Google Scholar 

  5. Loo C. (1985). A statistical model for a land mobile satellite link. IEEE Transactions on Vehicular Technology 34(3): 122–127

    Article  Google Scholar 

  6. Patzold M. (2002). Mobile fading channels. Chichester UK, Wiley

    Google Scholar 

  7. Clarke R.H. (1968). A statistical theory of mobile-radio reception. Bell System Technical Journal 47, 957–1000

    Google Scholar 

  8. Patzold M., Killat U., Laue F. (1998). An extended suzuki model for land mobile satellite channels and its statistical properties. IEEE Transactions on Vehicular Technology 47(2): 617–630

    Article  Google Scholar 

  9. Hashemi H. (1993). The indoor radio propagation channel. Proceedings of the IEEE 81(7): 943–968

    Article  Google Scholar 

  10. Adawi N.S., et al. (1988). Coverage prediction for mobile radio systems operating in the 800/900 MHz frequency range. IEEE Transactions on Vehicular Technology 37(issue 1): 3–72

    Google Scholar 

  11. Babitch F., Lombardi G. (2000). Statistical analysis and characterization of the indoor propagation channel. IEEE Transactions on Communications 48(3): 455–464

    Article  Google Scholar 

  12. Bithas P.S., Karagiannidis G.K., Sagias N.C., Mathiopoulos P.T., Kotsopoulos S.A., Corazza G.E. (2005). Performance analysis of a class of GSC receivers over nonidentical Weibull fading channels. IEEE Transactions on Vehicular Technology 54(6): 1963–1970

    Article  Google Scholar 

  13. Yacoub, M. D. (2002).The α–μ distribution: A general fading distribution. In Proc. IEEE International Symposium on Personal, Indoor, Mobile Radio Communications. IEEE PIMRC, Lisbon, Portugal, Sept. 2002.

  14. Sagias N.C., Karagiannidis G.K. (2005). Gaussian class multivariate Weibull distributions: Theory and applications in fading channels. IEEE Transactions on Information Theory 51(10): 3608–3619

    Article  MathSciNet  Google Scholar 

  15. Sagias N.S., Zogas D.A., Karagiannidis G.K. (2005). Selection diversity receivers over nonidentical Weibull fading channels. IEEE Transactions on Vehicular Technology 54(6): 2146–2151

    Article  Google Scholar 

  16. Fraidenraich G., Yacoub M.D., Santos Filho J.S. (2004). Second-order statistics of maximal-ratio and equal-gain combining in Weibull fading. IEEE Communication Letters 9(6): 499–501

    Article  Google Scholar 

  17. Sagias N.S., Zogas D.A., Karagiannidis G.K., Tombras G.S. (2004). Channel capacity and second order statistics in Weibull fading. IEEE Communication Letters 8(6): 377–379

    Article  Google Scholar 

  18. Patzold, M., & Nquyen, V. D. (2004). A spatial simulation model for shadow fading processes in mobile radio channels. In IEEE International Symposium on Personal, Indoor and Mobile Radio Communications. IEEE PIMRC 2004, Barcelona, Spain, Vol. 3, pp. 1832–1838, 5–8 Sept. 2004.

  19. Patzold, M., & Yang, K. (2006). An exact solution for the level crossing rate of shadow fading processes modeled by using the sum of sinusoids principle. In Proc. 9th International Symposium on Wireless Multimedia Communications. WPMC 2006, San Diego, USA, Sept. 2006, pp. 188–193.

  20. Karadimas, P., & Kotsopoulos, S. A. (2008). A simplified Weibull-lognormal fading channel: Analysis, simulation and validation. IEEE Transactions on Vehicular Technology (under revision).

  21. Ismail, M. H., & Matalgah, M. M. (2005). Outage probability analysis in cellular systems with noisy Weibull-faded lognormal-shadowed links. In Proceedings of the 10th IEEE Symposium on Computers and Communications (ISCC’05), pp. 269–274.

  22. Ismail, M. H., & Matalgah, M. M. (2005). Outage probability in multiple access systems with Weibull-faded lognormal-shadowed communication links. In Vehicular Technology Conference, 2005. VTC-2005-Fall. 2005, Vol. 4, pp. 2129–2133.

  23. Patzold, M., Killat, U., Laue, F., & Li, Y. (1996). A new and optimal method for the derivation of deterministic simulation models for mobile radio channels. In Proc. IEEE 46th Veh. Technol. Conf., VTC 96. Atlanta, Georgia, USA, pp. 1423–1427, April/May 1996.

  24. Patzold M., Killat U., Li Y., Laue F. (1997). Modeling, analysis and simulation of nonfrequency-selective mobile radio channels with asymmetrical doppler power spectral density shapes. IEEE Transactions on Vehicular Technology 46(2): 494–507

    Article  Google Scholar 

  25. Papoulis, A., & Pillai, S. U. (2002). Probability, random variables and stochastic processes (4th ed.). McGraw-Hill.

  26. Siqueira G.L., Vasquez E.J.A. (2000). Local and global signal variability statistics in a mobile urban environment. Wireless Personal Communications 15(issue 1): 61–78

    Article  Google Scholar 

  27. Gradshteyn I.S., Ryzhik I.M. (2000). Tables of integrals, series and products (6th ed). New York, Academic Press

    Google Scholar 

  28. Jakes, W. C. (1974). Microwave mobile communications. Wiley.

  29. Patzold M., Killat U., Laue F., Li Y. (1998). On the statistical properties of deterministic simulation models for mobile fading channels. IEEE Transactions on Vehicular Technology 47(1): 254–269

    Article  Google Scholar 

  30. Yacoub M.D., da Costa D.B., Dias U.S., Fraidenraich G. (2005). Joint statistics of two correlated Weibull variates. IEEE Antennas and Wireless Propagation Letters 4, 129–132

    Article  Google Scholar 

  31. Butterworth, J. S., & Matt, E.E. (1983). The characterization of propagation effects for land mobile satellite services. In Intern. Conf. Satellite Systems for Mobile Commun. Navigations, pp. 51–54, June 1983.

Download references

Author information

Authors and Affiliations

  1. Wireless Telecommunications Laboratory, Department of Electrical and Computer Engineering, University of Patras, 26500, Rio-Patras, Greece

    Petros Karadimas & Stavros A. Kotsopoulos

Authors
  1. Petros Karadimas
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Stavros A. Kotsopoulos
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Petros Karadimas.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Karadimas, P., Kotsopoulos, S.A. A Generalized Modified Suzuki Model with Sectored and Inhomogeneous Diffuse Scattering Component. Wireless Pers Commun 47, 449–469 (2008). https://doi.org/10.1007/s11277-008-9493-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11277-008-9493-2

Keywords