Abstract
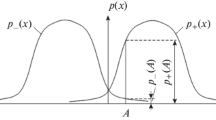
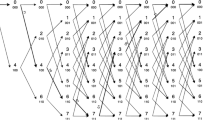
In this paper we propose a method that aims at reducing the complexity of convolutional and turbo decoding. Some calculations performed in decoding processing can be eliminated based on reliability thresholds. For convolutional and turbo decoding, the complexity is proportional to the number of branches in the trellis. For convolutional decoding, based on the Viterbi algorithm, we define reliability thresholds for the received samples of the signal and show that is possible to eliminate some branches in the trellis and consequently to reduce the complexity. For turbo decoding based on MAP algorithm, we set a threshold to classify each information bit log likelihood ratio (LLR). When the LLR is reliable, we take a decision on information bits and eliminate some branches in the trellis. Furthermore, we also define a criterion for stopping decoding wich further reduces the complexity. In this paper we show that it is possible to reduce decoding complexity of convolutional codes almost 80 % without performance degradation when compared to Viterbi algorithm over Rayleigh fading channels. In turbo decoding, we show that complexity varies with \({E_{b}}/{N_{0}}\) and it is reduced when more iterations are computed, tending to zero for higher iterations.











Similar content being viewed by others
References
van Berkel, C. H. (2009). Multi-core for mobile phones. In Proceedings of the design, automations. Test Europe conference exhibition (pp. 1260–1265).
Kienle, F., Wehn, N., & Meyr, H. (2011). On complexity, energy- and implementation-efficiency of channel decoders. IEEE Transactions on Communications, 99, 3301–3310.
Haccoun, D., Caron, M., & Nabli, M. (1999). Complexity reduction of the Viterbi algorithm using doubly complementary convolutional codes. In IEEE conference proceedings (pp. 408–411).
Dany, J.-C., et al. (2004). Low complexity algorithm for the decoding of convolutional codes of any rate. IEEE Conference Proceedings, 1, 547–551.
Wu, J., Vojcic, B. R., & Wang, Z. (2007). Turbo decoding complexity reduction by symbol selection and partial iterations. In IEEE GLOBECOM proceedings.
Frey, B. J. & Kschischang, F. R. (1998). Early detection and trellis splicing: Reduced-complexity iterative decoding. IEEE Journal on Selected Areas in Communications, 16, 153–159.
Jie, J., & Tsui, C. (2006). Low complexity SST Viterbi decoder. In IEEE vehicular technology conference (pp. 1–2).
Shin, Sooyoung Kim, & Lee, Soo In. (1999). Efficient complexity reduction technique in trellis decoding algorithm. IEEE Electronis Letters, 35, 16–17.
Ma, Z., Mow, W. H., & Fan, P. (2005). On the complexity reduction of turbo decoding for wideband CDMA. IEEE Transactions on Wireless Communications, 4, 353–356.
Shibutani, A., Suda, H., & Adachi, F. (1999). Complexity reduction of turbo decoding. IEEE Vehicular Technology Conference, 3, 1570–1574.
Minowa, T., & Imai, H. (2000). Reduced-complexity iterative decoding of high-rate turbo codes. IEEE Globecom, 1, 193–197.
Viterbi, A. J. (1967). Error bounds for convolutional codes and an asymptotically optimum decoding algorithm. IEEE Transactions on Information Theory, IT–13, 260–269.
Bahl, L. R., Cocke, J., Jelinek, F., & Raviv, J. (1974). Optimal decoding of linear codes for minimizing symbol error rate. IEEE Transactions on Information Theory, 20, 284–287.
Frison, C. I. (2009). Sub-optimal multiuser detector based on reliable samples. M. Eng. Thesis, Faculty of Electrical and Computer Engineering - Unicamp, Campinas, Brazil (in Portuguese).
Woodard, J. P., & Hanzo, L. (2000). Comparative study of turbo decoding techniques: An overview. IEEE Transactions on Vehicular Technology, 49, 2208–2233.
Berrou, C., Glavieux, A. & Thitimajshima, P. (1993). Near Shannon limit error-correcting coding and decoding: Turbo-codes. In Proceedings of the IEEE international conference communications (pp. 1064–1070).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Mataveli, L.O., de Almeida, C. Complexity Reduction of Convolutional and Turbo Decoding Based on Reliability Thresholds. Wireless Pers Commun 82, 1279–1290 (2015). https://doi.org/10.1007/s11277-015-2282-9
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11277-015-2282-9