Performance Analysis of Multiantenna MC DS CDMA System Over Correlated η–µ Multipath Fading Channels

Abstract

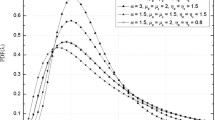
In wireless communication channels, the performance of the wireless communication systems deteriorate due to the severe influence of interference, multipath fading, path loss, shadowing, and noise, respectively. In this paper, we investigate the performance of the system transmitting over correlated η–µ frequency selective fading channels in terms of average error rates. Based on moment generating function approach, closed form expressions for average error probabilities for the system are derived and represented in terms of Appell’s hypergeometric functions and Lauricella’s multivariate hypergeometric functions. In addition, probability density function based approach is employed to determine the formulae for average error probabilities of the system operating over correlated η–µ multipath fading channels. The numerical results reveal that, the effects of correlation, decaying power factor and signal constellation size can be reduced using frequency, spatial antennas and path diversities, respectively. Furthermore, we confirm the correctness of the analytical approaches through Monte Carlo simulation technique.

Appendices

Appendix 1

The MGF of the instantaneous SINR at the output of the RAKE receiver is derived in this appendix. The envelope (R) of the fading signal is modelled by η–µ fading distribution. Then, the random variable γ = ‖R ²‖ has the PDF of η–µ distribution. Hence, a set of N_rM_tUL_r variates of η–µ random variables is equivalent to a set of 2µ independent Gaussian random variable vectors with N_rM_tUL_r dimensions. Since the instantaneous signal to interference noise ratio is given as in (8a)

$$\gamma_{s} = \gamma_{c} \sum\limits_{n = 1}^{{N_{t} }} {\sum\limits_{m = 1}^{{M_{t} }} {\sum\limits_{j = 1}^{U} {\sum\limits_{l = 1}^{{L_{r} }} {\sum\limits_{i = 1}^{2\mu } {R_{nmjli}^{2} } } } } }$$

(49)

where $R_{nmjli}^{2} = R_{nmjli}^{T} R_{nmjli} = X_{nmjli}^{T} X_{nmjli} + Y_{nmjli}^{T} Y_{nmjli}$, X_i1=nmjl = [X_i11, X_i12, …, X_i2µ]^T where i ₁  = nmjl = 1, 2, …, N_rM_tUL_r represent dependent inphase random variables. Hence, X_i1k are independent and identically distributed Gaussian random variables with zero mean and variance $E[X_{i1k}^{2} ]$, k = 1, 2, …, 2µ. Similarly, Y_i1=nmjl = [Y_i11, Y_i12, …, Y_i12µ]^T denote dependent quadrature random variables. Therefore, Y_i1k are independent identically distributed Gaussian random variables with zero mean and variance $E[Y_{i1k}^{2} ]$ [27]. Both are mutually independent Gaussian variables with $E[X_{nmjli} ] = E[Y_{nmjli} ] = 0, E[X_{nmjli}^{2} ] = \rho_{x}^{2} , E[Y_{nmjli}^{2} ] = \rho_{y}^{2}$, µ is the number of clusters of multipath. Therefore, the instantaneous SINR can be rewritten as

$$\gamma_{s} = \gamma_{c} \sum\limits_{n = 1}^{{N_{r} }} {\sum\limits_{m = 1}^{{M_{t} }} {\sum\limits_{j = 1}^{U} {\sum\limits_{l = 1}^{{L_{r} }} {\sum\limits_{i = 1}^{2\mu } {X_{nmjli}^{2} } } } } } + \gamma_{c} \sum\limits_{n = 1}^{{N_{r} }} {\sum\limits_{m = 1}^{{M_{t} }} {\sum\limits_{j = 1}^{U} {\sum\limits_{l = 1}^{{L_{r} }} {\sum\limits_{i = 1}^{2\mu } {Y_{nmjli}^{2} } } } } }$$

(50)

Considering the inphase random variates $X_{i1k}^{T} X_{i1k}$, similarly for quadrature random $Y_{i1k}^{T} Y_{i1k}$. For the case of inphase random variates, we assume the arbitrary covariance matrix as C_xx, then we can form a scalar quadratic function of vector X, i.e., suppose ${\text{Z}} = {\mathbf{X}}_{nmli}^{T} B_{nmli} {\mathbf{X}}_{nmli}$, where B_nmli is a 2µ by 2µ matrix. Hence, the joint PDF of $[{\text{X}}_{\text{nmlil}}^{2} + {\text{X}}_{{{\text{nmli}}2}}^{2} + \cdots + X_{{{\text{nmli}}2\upmu}}^{2} ]$ is [16, 28]

$$f\left( {X_{nmli} } \right) = \frac{1}{{\sqrt {\left( {2\pi } \right)^{2\mu } \det \left( {C_{xx} } \right)} }} \, \exp \left( { - \frac{1}{2}X_{nmli}^{T} C_{nmli}^{ - 1} X_{nmli} } \right)$$

(51)

Then, the MGF of Z is

$$\begin{aligned} \Phi_{x} (s) & = E\left[ {e^{{ - sX_{nmli}^{T} B_{nmli} X_{nmli} }} } \right] = \int\limits_{0}^{\infty } {\frac{1}{{\sqrt {\left( {2\pi } \right)^{2\mu } \det \left( {C_{xx} } \right)} }}} {\text{ e}}^{{ - \frac{ 1}{ 2}\left( {X_{nmli}^{T} C_{xx}^{ - 1} X_{nmli} } \right)}} {\text{ e}}^{{ - sX_{nmli}^{T} B_{nmli} X_{nmli} }} {\text{dX}}_{\text{nmli}} \\ & = \int\limits_{0}^{\infty } {\frac{1}{{\sqrt {\left( {2\pi } \right)^{2\mu } \det \left( {C_{xx} } \right)} }}} {\text{ e}}^{{ - \frac{ 1}{ 2}\left( {X_{nmli}^{T} \left( {C_{xx}^{ - 1} + 2sB_{nmli} } \right)X_{nmli} } \right)}} {\text{ dX}}_{\text{nmli}} \\ & = \frac{{\sqrt {\left( {\det \left( {F^{ - 1} } \right)} \right)} }}{{\sqrt {\left( {\det \left( {C_{xx} } \right)} \right)} }} \, \int\limits_{ 0}^{\infty } {\frac{ 1}{{\sqrt {\left( { 2\pi } \right)^{2\mu } \left( {\det \left( F \right)} \right)} }}} {\text{ e}}^{{ - \frac{ 1}{ 2}X^{T} F^{ - 1} X}} {\text{ dX}} \\ & = \frac{1}{{\det \left( {I_{nmli} + 2sB_{nmli} Cxx} \right)^{\mu } }} \\ \end{aligned}$$

(52)

where det denotes determinant. Therefore, for N_rM_tUL_r diversity branches, we have

$$\Phi_{x} (s) = \prod\limits_{n = 1}^{{N_{r} }} {\prod\limits_{m = 1}^{{M_{t} }} {\prod\limits_{i = 1}^{U} {\prod\limits_{l = 1}^{{L_{r} }} {\frac{1}{{\det \left( {I_{nmil} + 2sB_{nmil} C_{xx}^{nmil} } \right)^{{\mu_{nmil} }} }}} } } }$$

(53)

Similarly, for Y_nmil (quadrature Gaussian variates), we have

$$\Phi_{y} (s) = \prod\limits_{n = 1}^{{N_{r} }} {\prod\limits_{m = 1}^{{M_{t} }} {\prod\limits_{i = 1}^{U} {\prod\limits_{l = 1}^{{L_{r} }} {\frac{1}{{\det \left( {I_{nmil} + s2B_{nmil} D_{yy}^{nmil} } \right)^{{\mu_{nmil} }} }}} } } } ,$$

(54)

where D is the matrix. Therefore, overall MGF of instantaneous SINR at the RAKE receiver output is

$$\Phi_{x + y} (s) = \prod\limits_{n = 1}^{{N_{r} }} {\prod\limits_{m = 1}^{{M_{t} }} {\prod\limits_{i = 1}^{U} {\prod\limits_{l = 1}^{{L_{r} }} {\left( {\frac{1}{{\det \left( {I_{nmil} + 2sB_{nmil} C_{xx}^{nmil} } \right)^{{\mu_{nmil} }} \det \left( {I_{nmil} + 2sB_{nmil} D_{yy}^{nmil} } \right)^{{\mu_{nmil} }} }}} \right)} } } }$$

(55)

Appendix 2

In this appendix, we provide some hints for the solutions of (31), (36), (43), and (48), respectively. We consider

$$G_{1} = \int\limits_{0}^{\infty } {2Q\left( {\sqrt {2\gamma g} } \right)} \gamma^{i - 1} e^{ - \alpha \gamma } d\gamma = \int\limits_{0}^{\infty } {erfc\left( {\sqrt {g\gamma } } \right)} \gamma^{i - 1} e^{ - \alpha \gamma } d\gamma$$

(56)

Hence, we integrate using by parts

$$U = erfc\left( {\sqrt {g\gamma } } \right)\gamma^{i - 1} = \left( {\frac{2}{\sqrt \pi }\int\limits_{{\sqrt {g\gamma } }}^{\infty } {e^{{ - t^{2} }} } dt} \right)\gamma^{i - 1}$$

and dV = e ^−αγ dγ, then

$$dU = \left( { - \sqrt {\frac{g}{\pi }} \gamma^{i - 1.5} e^{ - g\gamma } + \left( {i - 1} \right)\gamma^{i - 2} erfc\left( {\sqrt {g\gamma } } \right)} \right)d\gamma$$

and $V = - \frac{1}{\alpha }e^{ - \alpha \gamma }$, then

$$\frac{{\left( {i - 1} \right)}}{\alpha }\int\limits_{0}^{\infty } {\gamma^{i - 2} } erfc\left( {\sqrt {g\gamma } } \right)e^{ - \alpha \gamma } d\gamma - \sqrt {\frac{g}{{\pi \alpha^{2} }}} \int\limits_{0}^{\infty } {\gamma^{i - 1.5} } e^{{ - \left( {\alpha + g} \right)\gamma }} d\gamma .$$

We have to integrate the first integral by parts, yields

$$\begin{aligned} & \frac{{\left( {i - 1} \right)\left( {i - 2} \right)}}{{\alpha^{2} }}\int\limits_{0}^{\infty } {\gamma^{i - 3} } erfc\left( {\sqrt {g\gamma } } \right)e^{ - \alpha \gamma } d\gamma - \frac{{\left( {i - 1} \right)}}{{\alpha^{2} }}\sqrt {\frac{g}{\pi }} \int\limits_{0}^{\infty } {\gamma^{i - 2.5} } e^{{ - \left( {\alpha + g} \right)\gamma }} d\gamma \\ & \quad - \sqrt {\frac{g}{{\pi \alpha^{2} }}} \int\limits_{0}^{\infty } {\gamma^{i - 1.5} } e^{{ - \left( {\alpha + g} \right)\gamma }} d\gamma \\ \end{aligned}$$

(57)

Therefore, the last two integrals are obtained from [29, 30, 31, Eq. (3.371)], again applying successive integration by parts to first integral, the general solution is given as

$$G_{1} = \frac{{\left( {i - 1} \right)!}}{{\alpha^{i} }}\left[ {1 - \sqrt {\frac{g}{{\pi \left( {g + \alpha } \right)}}} \sum\limits_{l = 0}^{i - 1} {\frac{{\alpha^{l} \Gamma \left( {l + 0.5} \right)}}{{l!\left( {g + \alpha } \right)^{l} }}} } \right]$$

(58)

Next, we consider the integral given below

$$G_{2} = \int\limits_{0}^{\infty } {2Q^{2} } \left( {\sqrt {2g\gamma } } \right)\gamma^{i - 1} e^{ - \alpha \gamma } d\gamma = \int\limits_{0}^{\infty } {erfc^{2} } \left( {\sqrt {g\gamma } } \right)\gamma^{i - 1} e^{ - \alpha \gamma } d\gamma$$

(59)

Then, let $z = \sqrt {g\gamma }$ and $\gamma = z^{2} /g,{\text{ d}}\gamma {\text{ = 2zdz/g}}$, we have

$$G_{2} = \frac{2}{{g^{i} }}\int\limits_{0}^{\infty } {z^{2i - 1} } erfc^{2} \left( z \right)e^{{ - kz^{2} }} dz$$

where k = α/g, then integrating by parts

$$U = erfc^{2} \left( z \right), \quad dU = - \frac{4}{\sqrt \pi }erfc\left( z \right)e^{{ - z^{2} }} dz$$

and

$$dV = z^{2i - 1} e^{{ - kz^{2} }} dz, \quad V = \int {z^{2i - 1} } e^{{ - kz^{2} }} dz$$

Hence, from [31, Eq. (2.326.11)]

$$V = - \frac{{\left( {i - 1} \right)!}}{{k^{i} }}e^{{ - kz^{2} }} \sum\limits_{l = 0}^{i - 1} {\frac{{\left( {kz^{2} } \right)^{l} }}{l!}} ,$$

then the integral becomes

$$\frac{{\left( {i - 1} \right)!}}{{k^{i} }} - \frac{{4\left( {i - 1} \right)!}}{{\sqrt \pi k^{i} }}\sum\limits_{l = 0}^{i - 1} {\frac{{k^{l} }}{l!}} \int\limits_{0}^{\infty } {erfc\left( z \right)} e^{{ - \left( {1 + k} \right)z^{2} }} dz,$$

then considering the integral part

$$y = \left( {1 + k} \right)z^{2} ,\quad z = \sqrt {\frac{1}{1 + k}} y^{0.5} ,\quad dz = 0.5\sqrt {\frac{1}{k + 1}} y^{ - 0.5} dy$$

and putting

$$erfc\left( {\sqrt {A\gamma } } \right) = \frac{{\Gamma \left( {0.5,A\gamma } \right)}}{\sqrt \pi }$$

Then from [27, 32, 31, Eq. (6.455.1)], the solution is

$$G_{2} = \left( {\frac{g}{\alpha }} \right)^{i} \left( {i - 1} \right)!\left[ {1 - \frac{4}{\pi }\left( {\frac{g}{2g + \alpha }} \right)\sum\limits_{l = 0}^{i - 1} {\frac{{\alpha^{l} }}{{\left( {2l + 1} \right)\left( {2g + \alpha } \right)^{l} }} \, {}_{2}F_{1} \left( {1,l + 1;l + 1.5;\frac{g + \alpha }{2g + \alpha }} \right)} } \right]$$

(60)

Therefore, the expressions for G₁ can be employed in (30), (35), while for both G₁ and G₂ in (42), and (47), respectively.