Abstract
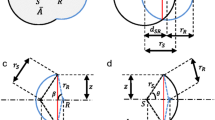
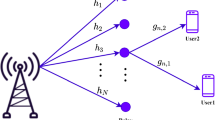
We investigate the optimal location of an adaptive decode and forward relay operating over a \(\kappa\)–\(\mu\) fading channel. The \(\kappa\)–\(\mu\) statistics provides a generalized line-of-sight propagation model which includes fading models like Rayleigh, Nakagami, Rician as special cases. We restrict our analysis to collinear relay placement, i.e. the relay node \((R_n)\) is on the same straight line between the source node \((S_n)\) and the destination node \((D_n)\). In the non-cooperative mode, \(D_n\) accepts only the two-hop transmission via \(R_n\) and discards any direct signal that may be available from \(S_n\). On the other hand, in the cooperative mode, \(D_n\) accepts both the replicas and combine them following either selection combining (SC) or maximum ratio combining (MRC). It is interesting to see that such cooperation does not always lead to energy saving, especially for small \(S_n-D_n\) separation. Also, worth mentioning the fact that MRC may not be optimal from the energy efficiency perspective, and SC can outperform MRC under certain channel conditions. In our paper, we also studied how parameters like spectral efficiency (R), path loss exponent (n), and fading parameters (\(\kappa ,\mu\)) affect the optimal relay placement location.









Similar content being viewed by others
Notes
For a DF relay we do not consider the \(S_n-R_n\) path SNR. For an amplify-and-forward (AF) relay, \(\gamma _{RD}\) should be replaced by end-to-end SNR \(\gamma _{SRD}\) [13].
For \(\kappa =1\), the \(\kappa\)–\(\mu\) distribution reduces to Rician distribution with \(\mu\) being equal to the Rician K factor [15].
We can achieve Rayleigh fading as a special case of \(\kappa\)–\(\mu\) fading by considering the values \(\kappa =0\) and \(\mu =1\) [16].
References
Li, G. Y., Xu, Z. K., Xiong, C., Yang, C. Y., Zhang, S. Q., Chen, Y., et al. (2011). Energy-efficient wireless communications: Tutorial, survey, and open issues. IEEE Wireless Communication Magazine, 18(6), 28–35.
Feng, D., Jiang, C., Lim, G., Cimini, L. J., Feng, G., & Li, G. Y. (2013). A survey of energy-efficient wireless communications. IEEE Communications Surveys & Tutorials, 15(1), 167–178.
Biswas, N., Ghosh, B., & Chandra, A. (2013). Energy efficient relay node placement in a eta-mu fading channel. In: Proceedings of IEEE conference on information & communication technologies (ICT) (pp. 1–5), Kumaracoil, TN, India.
Ghosh, B., Ghosh, A., Biswas, N., & Chandra, A. (2012). Placing the ‘third’ node: An energy efficiency perspective. In: Proceedings of IEEE international conference on computers and devices for communication (CODEC) (pp. 1–4), Kolkata, India.
Chandra, A., Biswas, S., Ghosh, B., Biswas, N., Brante, G., & Souza, R. D. (2014). Energy efficient relay placement in dual hop 802.15.4 networks. Wireless Personal Communications, 75(4), 1947–1967.
Ghosh, B., Chandra, A., & Mal, A. K. (2016). Energy efficient DF relay placement in a \(\alpha -\mu\) fading channel with cooperative and non-cooperative schemes. Radioengineering, 25(4), 749–756.
Moraes, A. C., DaCosta, D. B., & Yacoub, M. D. (2012). An outage analysis of multibranch diversity receivers with cochannel interference in \(\alpha\)–\(\mu\), \(\kappa\)–\(\mu\), and \(\eta\)–\(\mu\) fading scenarios. Wireless Personal Communications, 64(1), 3–19.
Yacoub, M. D. (2001). The \(\kappa{-}\mu\) distribution: A general fading distribution. In: Proceedings of IEEE vehicular technology conference (pp. 1–5), Atlantic City, NJ.
Chandra, A., Ghosh, B., Biswas, N., Brante, G., & Souza, R. D. (2013). Energy efficient relay placement for dual hop wireless transmission. International Journal of Electronics Letters, 1(4), 198–209.
Ghosh, B., Chandra, A., & Mal, A. K. (2017). Comparing energy efficiency of DF relay-assisted cooperative and non-cooperative short range wireless systems. In V. Nath (ed), Proceedings of international conference on nano-electronics, circuits & communication systems, Vol. 403 of Lecture Notes in Electrical Engineering (pp. 1–6). Springer.
Wang, S., & Nie, J. (2010). Energy efficiency optimization of cooperative communication in wireless sensor networks. EURASIP Journal on Wireless Communications and Networking, 2010(162326), 1–8.
Goldsmith, A. (2005). Wireless Communications. Cambridge: Cambridge University Press.
Brante, G., Kakitani, M. T., & Souza, R. D. (2011). Energy efficiency analysis of some cooperative and non-cooperative transmission schemes in wireless sensor networks. IEEE Transactions on Communications, 59(10), 2671–2677.
Lin, M., Wei, H., Ouyang, J., An, K., & Yuan, C. (2014). Performance analysis of a dual-hop cooperative relay network with co-channel interference. Radioengineering, 23(4), 1234–1240.
Yacoub, M. D. (2007). The \(\kappa{-}\mu\) distribution and the \(\eta{-}\mu\) distribution. IEEE Antennas and Propagation Magazine, 49(1), 1–14.
Kumbhani, B., & Kshetrimyum, R. S. (2015). MGF based approximate SER calculation of SM MIMO systems over generalized \(\kappa\)–\(\mu\) and \(\eta\)–\(\mu\) fading channels. Wireless Personal Communications, 83(3), 1903–1913.
Kumbhani, B., & Kshetrimyum, R. S. (2015). Performance analysis of MIMO systems with antenna selection over generalized \(\kappa\)–\(\mu\) fading channels. IETE Journal of Research, 62(1), 45–53.
Bhargav, N., da Silva, C. R. N., Chun, Y. J., Cotton, S. L., & Yacoub, M. D. (2017). Co-channel interference and background noise in \(\kappa{-}\mu\) fading channels. IEEE Communications Letters,. doi:10.1109/LCOMM.2017.2664806.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Ghosh, B., Chandra, A. & Mal, A.K. Optimal Location of Energy Efficient DF Relay Node in \(\varvec{\kappa}\)–\(\varvec{\mu }\) Fading Channel. Wireless Pers Commun 96, 669–682 (2017). https://doi.org/10.1007/s11277-017-4195-2
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11277-017-4195-2