Abstract
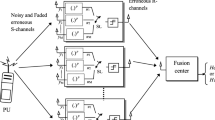
Spectrum sensing is an important task to find out the spectrum holes in a given radio spectrum. Spectrum sensing performance is improved with the aid of multiple cognitive radios (CRs) in the network and each CR having multiple antennas in different fading channels (Rayleigh, Hoyt and Weibull fading channel). In this paper, we have considered that each CR having multiple antennas and improved energy detector (IED) scheme is used for detection of primary user. Imperfect channel is considered between CRs and fusion center (i.e. in reporting channel) with an error probability rate (r). We have derived the novel expression for missed detection probability (P m ), closed form of expression to find out optimal value of number of CR users (N opt ), closed form of expression and procedure to find an optimal value of normalized threshold value (λ n,opt ), expression for optimal value of arbitrary power of received signal (P opt ) and calculation of minimum value of total error in different fading channels are provided. Performance is evaluated to find out (N opt ), (λ n,opt ), (P opt ) and to calculate minimum error value for various network parameters like SNR, r, λ n and multiple number of antennas (M). It is observed that detection performance is improved by using multiple antennas at each CR. Comparison between conventional energy detector and IED, comparison among Rayleigh, Hoyt and Weibull fading channels also provided for different cases of network parameters.











Similar content being viewed by others
Change history
14 February 2018
The last author’s affiliation was incorrect in the original publication. The correct affiliation is ECE Department, Marri Laxman Reddy Institute of Technology and Management, Dundigal, India.
References
Federal Communications Commission. (2002). Spectrum policy task force. ET Docket 02-135, November 2002.
Mitola, J., & Maguire, G. Q. (1999). Cognitive radio: Making software more personal. IEEE Personal Communications, 6(4), 13–18.
Yucek, T., & Arslan, H. A. (2009). survey of spectrum sensing algorithms for cognitive radio applications. IEEE Communications Surveys and Tutorials, 11(1), 116–130.
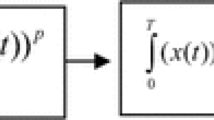
Urkowitz, H. (1967). Energy detection of unknown deterministic signals. Proceedings of the IEEE, 55(4), 523–531.
Lopez-Benitez, M., & Casadevall, F. (2012). Improved energy detection spectrum sensing for cognitive radio. IET Communications, 6(8), 785–796.
Ghasemi, A. & Sousa, E. S. (2005). Collaborative spectrum sensing for opportunistic access in fading environments. In Proceedings of 1st IEEE International Symposium on New Frontiers in Dynamic Spectrum Access Networks (pp. 131–136), Baltimore, USA.
Zhang, W., Mallik, R. K., & Letaief, K. B. (2009). Optimization of cooperative spectrum sensing with energy detection in cognitive radio networks. IEEE Transactions on Wireless Communications, 8(12), 5761–5766.
Singh, A., Bhatnagar, M. R., & Mallik. R. K. (2011). Cooperative spectrum sensing with an improved energy detector in cognitive radio network. In Proceedings of National Conference on Communications, January 2011 (pp. 1–5), Bangalore, India.
Singh, A., Bhatnagar, M. R., & Mallik, R. K. (2011). Optimization of cooperative spectrum sensing with an improved energy detector over imperfect reporting channels. In Proceedings of IEEE Vehicular Technology conference. September 2011, (pp. 1–5), San Francisco, USA.
Singh, A., Bhatnagar, M. R., & Mallik, R. K. (2012). Cooperative spectrum sensing in multiple antenna based cognitive radio network using an improved energy detector. IEEE Communications Letters, 16(1), 64–67.
Srinivas, N., Chandra, A., Roy, S. D., & Kundu, S. (2013). Performance of improved energy detector based cooperative spectrum sensing over Hoyt and Rician fading channels. IEICE Communication Express, 2(7), 319–324.
Digham, F. F., Alouini, M. -S., & Simon, M. K. (2003). On the energy detection of unknown signals over fading channels. In Proceedings of IEEE International Conference on Communications (ICC’03). May 2003 (pp. 3575–3579), Alaska, USA.
Ranjeeth, M., & Anuradha, S. (2016). Threshold based censoring of cognitive radios in Rician fading channel. Wireless Personal Communications, 93(2), 409–430.
Chen, Y. (2010). Improved energy detector for random signals in Gaussian noise. IEEE Transactions on Wireless Communications, 9(2), 558–563.
Ranjeeth, M., Anuradha, S., Srinivas, N., & Behera, S. (2016). Optimization of cooperative spectrum sensing based on improved energy detector with selection diversity in AWGN and Rayleigh fading. In IEEE conference on ICEEOT, Chennai, March-2016.
Simon, M. K., & Alouini, M.-S. (2004). Digital communication over fading channels (2nd ed.). New Jersey: Wiley.
Sofotasios, P. C., Fikadu, M. K., Ho-Van, K., & Valkama, M. (2013). Energy detection sensing of unknown signals over Weibull fading channels. In International Conference on Advanced Technologies for Communications (ATC-2013), (pp. 414–419).
Bhatnagar, M. R., Hjørungnes, A., & Debbah, M. (2010). Delay-tolerant decode-and-forward based cooperative communication over Ricean channels. IEEE Transactions on Wireless Communications, 9(4), 1277–1282.
Author information
Authors and Affiliations
Corresponding author
Additional information
A correction to this article is available online at https://doi.org/10.1007/s11277-018-5500-4.
Appendices
Appendix 1
1.1 Missed Detection Probability \((P_{m} )\) in Rayleigh Fading Channel
Probability density function for Rayleigh fading channel when the signal is present is given in [10] as:
Missed detection probability can be calculated for Rayleigh fading channel as [9]
where \(\gamma = {\raise0.7ex\hbox{${E_{s} \sigma_{h}^{2} }$} \!\mathord{\left/ {\vphantom {{E_{s} \sigma_{h}^{2} } {\sigma_{n}^{2} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\sigma_{n}^{2} }$}}\) is sensing channel SNR.
We have considered that each CR is having multiple antennas and selection combining (SC) scheme is used to select the maximum value of antenna among all antennas (M).
Using SC diversity scheme maximum value from all branches can be calculated as described in [10]
Under hypothesis \(H_{1}\): using SC diversity scheme missed detection probability can be calculated as:
Having multiple antennas and using SC diversity scheme at each CR, expression for \(P_{m}\) in Rayleigh fading channel is:
1.2 Missed Detection Probability \((P_{m} )\) in Hoyt Fading Channel
Probability density function for Hoyt fading channel when the signal is present is given in [11] as:
where \(\sigma_{1}^{2} = \frac{{E_{s} \sigma_{I}^{2} + \sigma_{n}^{2} }}{2}\) and \(\sigma_{2}^{2} = \frac{{E_{s} \sigma_{q}^{2} + \sigma_{n}^{2} }}{2}\). \(\sigma_{I} = \sqrt {\frac{{\varOmega q^{2} }}{{1 + q^{2} }}}\), \(\sigma_{q} = \sqrt {\frac{\varOmega }{{1 + q^{2} }}}\) and ‘q’ is the Hoyt fading parameter that ranges from 0 to 1.
Missed detection probability can be calculated for Hoyt fading channel as [11]
Missed detection probability can be calculated from CDF of Hoyt fading channel, it is given in [11], as
where \(A = {\kern 1pt} \,\left( {\frac{1}{4}} \right)\left( {\frac{1}{{\sigma_{2}^{2} }} + \frac{1}{{\sigma_{1}^{2} }}} \right)\), \(B = {\kern 1pt} \,\left( {\frac{1}{4}} \right)\left( {\frac{1}{{\sigma_{2}^{2} }} - \frac{1}{{\sigma_{1}^{2} }}} \right)\) and \(u_{1} = \sqrt {\left( {A - \sqrt {A^{2} - B}^{2} } \right)z^{2/p} }\),
Having multiple antennas and using SC diversity scheme at each CR, expression for \(P_{m}\) in Hoyt fading channel is:
1.3 Missed Detection Probability \((P_{m} )\) in Weibull Fading Channel
Probability density function for Weibull fading channel when the signal is present is given in [17] as:
Where \(C = {\raise0.7ex\hbox{$V$} \!\mathord{\left/ {\vphantom {V 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}\) and \(P = 1 + {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 C}}\right.\kern-0pt} \!\lower0.7ex\hbox{$C$}}\) V – is Weibull fading parameter.
Missed detection probability can be calculated for Weibull fading channel as [9]
where \(\gamma = {\raise0.7ex\hbox{${E_{s} \sigma_{h}^{2} }$} \!\mathord{\left/ {\vphantom {{E_{s} \sigma_{h}^{2} } {\sigma_{n}^{2} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\sigma_{n}^{2} }$}}\) is sensing channel SNR, \(C = {\raise0.7ex\hbox{$V$} \!\mathord{\left/ {\vphantom {V 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}\) and \(P = 1 + {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 C}}\right.\kern-0pt} \!\lower0.7ex\hbox{$C$}}\).
In above expression, if we substitute V = 2 in (40), it is similar to (27). It refers that if Weibull fading parameter value becomes (V = 2), then that distribution becomes to Rayleigh distribution.
Having multiple antennas and using SC diversity scheme at each CR, expression for \(P_{m}\) in Weibull fading channel is:
Appendix 2
2.1 Optimization of Normalized Threshold Value \((\lambda_{n,opt} )\) in Rayleigh Fading Channel
To find out optimal value of normalized threshold
For single antenna at each CR in the network;
Case (i) For M = 1, N = 1, q = 0.1, and p = 2 represent the single antenna with CED, \((\lambda_{n,opt} )\) value is:
Case (ii) For M = 1, N = 1, q = 0.1, and p = 3 represent the single antenna with IED, \((\lambda_{n,opt} )\) value is:
Case (iii) For M = 1, N = 1, q = 0.1, and p = 4 represent the single antenna with IED, \((\lambda_{n,opt} )\) value is:
2.2 Optimization of Normalized Threshold Value \((\lambda_{n,opt} )\) in Hoyt Fading Channel
To find out optimal value of normalized threshold
For single antenna at each CR in the network;
Where \(\gamma = {{E_{s} \sigma_{h}^{2} } \mathord{\left/ {\vphantom {{E_{s} \sigma_{h}^{2} } {\sigma_{n}^{2} }}} \right. \kern-0pt} {\sigma_{n}^{2} }}\) is sensing channel SNR.
2.3 Optimization of Normalized Threshold Value \((\lambda_{n,opt} )\) in Weibull Fading Channel
To find out optimal value of normalized threshold
For single antenna at each CR in the network;
Substituting N = 1, M = 1, q = 0.1 and (52), (53) in (51), then equating to zero we get an equation for optimal value normalized threshold value in the form of;
Appendix 3
3.1 Optimization of Arbitrary Power of Received Signal Value \((P_{opt} )\) in Rayleigh Fading Channel
To find out optimal value of arbitrary power of received signal
For single antenna at each CR in the network;
where \(\gamma = {{E_{s} \sigma_{h}^{2} } \mathord{\left/ {\vphantom {{E_{s} \sigma_{h}^{2} } {\sigma_{n}^{2} }}} \right. \kern-0pt} {\sigma_{n}^{2} }}\) is sensing channel SNR.
Substituting N = 1, M = 1 and (56), (57) in (55) results in
Substituting N = 2, M = 1 and (56), (57) in (55) results in
where\(x = \lambda^{{{\raise0.7ex\hbox{$2$} \!\mathord{\left/ {\vphantom {2 p}}\right.\kern-0pt} \!\lower0.7ex\hbox{$p$}}}}\) \(C_{1} = \left( {1 + \frac{1}{1 + \gamma }} \right)\) \(C_{2} = \left( {\frac{1}{1 + \gamma }} \right)\) \(C_{3} = \ln (C_{2} )\)Finally, expression for optimal value of arbitrary power of received signal is:
3.2 Optimization of Arbitrary Power of Received Signal Value \((P_{opt} )\) in Weibull Fading Channel
To find out optimal value of arbitrary power of received signal
For single antenna at each CR in the network;
where \(\gamma = {{E_{s} \sigma_{h}^{2} } \mathord{\left/ {\vphantom {{E_{s} \sigma_{h}^{2} } {\sigma_{n}^{2} }}} \right. \kern-0pt} {\sigma_{n}^{2} }}\) is sensing channel SNR.Substituting N = 1, M = 1, q = 0.1 and (62), (63) in (61) results in
Rights and permissions
About this article
Cite this article
Ranjeeth, M., Anuradha, S. & Nallagonda, S. Performance Analysis of Cooperative Spectrum Sensing Network Using Optimization Technique in Different Fading Channels. Wireless Pers Commun 97, 2887–2909 (2017). https://doi.org/10.1007/s11277-017-4640-2
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11277-017-4640-2