Skip to main content
SpringerLink
Log in

An Empirical Comparison of Encoding Algorithms of the Low Density Parity Check Code for Decode and Forward Protocol Relay System

  • Published:
Wireless Personal Communications Aims and scope Submit manuscript

Abstract

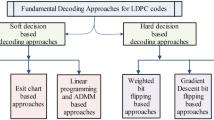
Decode and a forward (DF) protocol relay technique in cooperative communication is becoming a more attractive method to achieve a higher throughput requirement for wireless communication these days. But the high complexity operation is a major issue in the DF protocol relay processing system. The encoding operation which is a pre-processing stage of the decoding process consumes high complexity in the DF protocol relay system. Thus, it is important to tackle the encoding operation to ensure low complexity and good performance of the subsequent decoding operation in the DF protocol relay system. Thus far, very limited works have been reported on identifying a suitable encoding algorithm of encoding operation for the DF protocol relay system using the Low Density Parity Check (LDPC) code. In this paper, we present a suitable encoding algorithm for the DF protocol relay system using the LDPC code. To find out the most suitable encoding algorithm for the DF protocol relay system using LDPC code, we introduce the LDPC code encoding model. Eight different encoding algorithms based on Lower Upper and Orthogonal Upper algorithms presented in the LDPC code encoding model. The encoding execution time, the number of nonzero, and the pattern of nonzero was used to evaluate the computational complexity performance of the designed LDPC code encoding model. The most suitable encoding algorithm for the required criteria of the DF protocol relay system using the LDPC code is identified.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19

Similar content being viewed by others

References

  1. AboHashish, S. M. M., Rizk, R. Y., & Zaki, F. W. (2019). Energy efficiency optimization for relay deployment in multi-user LTE-advanced networks. Wireless Personal Communications, 108, 297–323.

    Article  Google Scholar 

  2. Kumar, P., & Dhaka, K. (2019). Average BER and resource allocation in wireless powered decode and forward relay system. IET Communications, 13(4), 379–386.

    Article  Google Scholar 

  3. Patil, M. V., Pawar, S. & Saquib, Z. (2020). Coding techniques for 5G networks: A review. In 3rd International conference on communication system, computing and IT applications (CSCITA) (pp. 208–213).

  4. Suud, J., Zen, H., Othman, A. K., & Ab Hamid, K. (2018). Decoding of decode and forward relay protocol using min-sum based low density parity check system. International Journal of Communication Networks and Information Security, 10(1), 199–212.

    Google Scholar 

  5. Nguyen, T. T. B., Tan, T. N., & Lee, H. (2019). Efficient QC-LDPC encoder for 5G new radio. Electronics, 8(6), 668.

    Article  Google Scholar 

  6. Kaji, Y. (2006). Encoding LDPC codes using the triangular factorization. EICE Transactions on Fundamentals of Electronics Communications and Computer Sciences, E89-A(10), 2510–2518.

    Article  Google Scholar 

  7. Neal, R. M. (1999). Sparse matrix methods and probabilistic inference algorithms the parity check matrix MA summer program on codes, systems, and graphical models (pp. 1–15).

  8. Vishnu, N. V., & Sajith, S. P. (2015). A memory efficient fully parallel QC-LDPC encoder. International Journal of Science and Research, 4(7), 323–327.

    Google Scholar 

  9. Reid, M. W. (2014). Pivoting for LU factorization. Retrieved June 11, 2017, from http://buzzard.ups.edu/courses/2014spring/420projects/math420-UPS-spring-2014-reid-LU-pivoting.pdf.

  10. Francis, J. G. F. (1962). The qr transformation-part 2. The Computer Journal, 4(4), 332–345.

    Article  Google Scholar 

  11. Krishnamoorthy, A. (2014). Symmetric QR algorithm with permutations. arXiv preprint arXiv:1402.5086 (Vol. 8, pp. 8–10).

  12. Matsumura, K., & Ohtsuki, T. (2011). Orthogonal beamforming using gram schmidt orthogonalization for multi-user mimo downlink system. EURASIP Journal on Wireless Communication and Networking. https://doi.org/10.1186/1687-1499-2011-41.

    Article  Google Scholar 

  13. Chen, F. C., Chang, R. C., Lin, C., Lin, K., & Huang, C. (2010). Iterative QR decomposition architecture using the modified gram-schmidt algorithm for MIMO systems. IEEE Transactions on Circuits and Systems I: Regular Papers, 57(5), 1095–1102.

    Article  MathSciNet  Google Scholar 

  14. Heath, R. W. J., Rusu, C., & Gonzalez Prelcic, N. (2016). Fast orthonormal sparsifying transforms based on householder reflectors. IEEE Transactions on Signal Processing, 64, 6589–6599.

    Article  MathSciNet  Google Scholar 

  15. Leung, C. S., & Cheung, K. F. (1991). Householder encoding for discrete bidirectional associative memory. IEEE International Joint Conference Neural Networks, 1, 237–241.

    Google Scholar 

  16. Merchant, F., Vatwani, T., Chattopadhyay, A., Raha, S., Nandy, S. K., Narayan, R., & Leupers, R. (2018). Efficient realization of givens rotation through algorithm architecture co-design for acceleration of qr factorization. arxiv:1803.05320 (pp. 1–12).

  17. Faverge, M., Herrmann, J., Langou, J., Lowery, B., Robert, Y., & Dongarra, J. (2015). Mixing LU and QR factorization algorithms to design high-performance dense linear algebra solvers. Journal of Parallel and Distributed Computing, 85, 32–46.

    Article  Google Scholar 

Download references

Acknowledgements

Jamaah Suud would like to acknowledge the Ministry of Higher Education Malaysia (MoHE) for their financial support through the HLP Ph.D. scholarship scheme and Universiti Malaysia Sarawak (UNIMAS) and Kuching Polytechnic Sarawak for providing the resources to carry out this research.

Author information

Authors and Affiliations

  1. Faculty of Engineering, University Malaysia Sarawak, Kota Samarahan, Kuching, Sarawak, Malaysia

    S. Jamaah, Z. Hushairi, O. Al-Khalid & A. H. Khairuddin

Authors
  1. S. Jamaah
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Z. Hushairi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. O. Al-Khalid
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. A. H. Khairuddin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Jamaah.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jamaah, S., Hushairi, Z., Al-Khalid, O. et al. An Empirical Comparison of Encoding Algorithms of the Low Density Parity Check Code for Decode and Forward Protocol Relay System. Wireless Pers Commun 117, 2007–2026 (2021). https://doi.org/10.1007/s11277-020-07955-2

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11277-020-07955-2

Keywords

Search

Navigation