Skip to main content

Advertisement

Springer Nature Link
Log in

Linear Algebraic Theory for Designing the Bus Topology to Enhance the Data Transmission Process

  • Published:
Wireless Personal Communications Aims and scope Submit manuscript

Abstract

Bus topology performs a significant part for describing the data transfer from one end to another in a single direction, where one node performs as a source and another end performs as the destination. The complexity of utilizing the bus topology is if the cable in the network has an issue then the entire network will be shut down. Moreover, high network traffic will arise which decreases the network performance because all the information is transferred in a single cable. The bus topology issue is complex to enhance the normal process but mathematically it is simple to reconstruct the function. Therefore, in this research data transmission of the bus topology can be designed by a novel linear algebraic theory. Besides, the linear algebraic theory for bus topology is validated by the specific theorems and the rank of the matrix is estimated by the proper calculations. The proposed design of bus topology is created by the MATLAB platform. Moreover, the created bus topology is validated in the Local Area Network (LAN) application for data transmission. Consequently, the proposed theory is compared with the other conventional techniques and proven the reliability of the novel method. Besides, the proposed bus topology design in LAN is compared with other existing methods in terms of throughput, delay, and packet delivery ratio. Therefore the results proved that the proposed scheme can attain better performance than the other conventional techniques.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

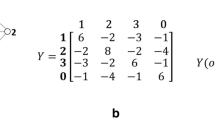
Source to Destination through the proposed bus topology

Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data Availability

Enquiries about data availability should be directed to the authors.

Notes

  1. The two vector spaces have invertible linear transformation which is called an isomorphism.

  2. Augmented matrix is the matrix which is used for performing the similar row operations on every given matrix.

  3. This is the process of solving the linear equation that has been changed into row-echelon form.

  4. Hermitian matrix is the complex square matrix which is equal to the conjugate transpose.

  5. variational theorem states that the trial energy can be larger or equal to the true energy.

References

  1. Ojha, R. P., Srivastava, P. K., Sanyal, G., & Gupta, N. (2021). Improved model for the stability analysis of wireless sensor network against malware attacks. Wireless Personal Communications, 116(3), 2525–2548. https://doi.org/10.1007/s11277-020-07809-x

    Article  Google Scholar 

  2. Kuruba, P., & Dushyantha, N. D. (2021). Stability control in polygon based topology formation and information gathering in satellite based wireless sensor network. Wireless Personal Communications, 120(4), 2491–2518. https://doi.org/10.1007/s11277-020-07568-9

    Article  Google Scholar 

  3. Sharma, S., & Verma, V. K. (2022). An integrated exploration on internet of things and wireless sensor networks. Wireless Personal Communications. https://doi.org/10.1007/s11277-022-09487-3

    Article  Google Scholar 

  4. Cheney, W., & Kincaid, D. (2009). Linear algebra: Theory and applications. The Australian Mathematical Society, 110.

  5. Ahmed, M. I., & Kannan, G. (2021). Secure end to end communications and data analytics in IoT integrated application using IBM Watson IoT platform. Wireless Personal Communications, 120(1), 153–168. https://doi.org/10.1007/s11277-021-08439-7

    Article  Google Scholar 

  6. Sundaram, K., & Vellupillai, S. (2021). Designing a novel star topology using operad linear differential theory. Wireless Personal Communications, 120(1), 565–585. https://doi.org/10.1007/s11277-021-08478-0

    Article  Google Scholar 

  7. Pade, J., & Tischendorf, C. (2019). Waveform relaxation: A convergence criterion for differential-algebraic equations. Numerical Algorithms, 81(4), 1327–1342. https://doi.org/10.1007/s11075-018-0645-5

    Article  MathSciNet  MATH  Google Scholar 

  8. Zhu, F., Zhang, C., Zheng, Z., & Farouk, A. (2021). Practical network coding technologies and softwarization in wireless networks. IEEE Internet of Things Journal, 8(7), 5211–5218. https://doi.org/10.1109/JIOT.2021.3056580

    Article  Google Scholar 

  9. Patil, D., Tesi, P., & Trenn, S. (2019). Indiscernible topological variations in DAE networks. Automatica, 101, 280–289. https://doi.org/10.1016/j.automatica.2018.12.012

    Article  MathSciNet  MATH  Google Scholar 

  10. Sriyananda, M. G. S., Wang, X., & Rao, R. K. (2021). Crowdsensing-assisted path loss estimation and management of dynamic coverage in 3D wireless networks with dense small cells. IEEE Access, 9, 112670–112685. https://doi.org/10.1109/ACCESS.2021.3100085

    Article  Google Scholar 

  11. Fang, Q., & Peng, J. (2018). Synchronization of fractional-order linear complex networks with directed coupling topology. Physica A: Statistical Mechanics and its Applications, 490, 542–553. https://doi.org/10.1016/j.physa.2017.08.050

    Article  MathSciNet  MATH  Google Scholar 

  12. Jogunola, O., Adebisi, B., Anoh, K., Ikpehai, A., Hammoudeh, M., Harris, G., & Gacanin, H. (2018). Distributed adaptive primal algorithm for P2P-ETS over unreliable communication links. Energies, 11(9), 2331. https://doi.org/10.3390/en11092331

    Article  Google Scholar 

  13. Zhang, J., Raza, M., Khalid, R., Parveen, R., & Ramírez-Asís, E. H. (2021). Impact of team knowledge management, problem solving competence, interpersonal conflicts, organizational trust on project performance, a mediating role of psychological capital. Annals of Operations Research. https://doi.org/10.1007/s10479-021-04334-3

    Article  Google Scholar 

  14. Li, H., Zheng, Y., & Alsaadi, F. E. (2019). Algebraic formulation and topological structure of Boolean networks with state-dependent delay. Journal of Computational and Applied Mathematics, 350, 87–97. https://doi.org/10.1016/j.cam.2018.10.003

    Article  MathSciNet  MATH  Google Scholar 

  15. Carrell, J. B. (2005). Fundamentals of linear algebra. The University of British Columbia.

  16. Anh, L. Q., & Van Hung, N. (2018). Stability of solution mappings for parametric bilevel vector equilibrium problems. Computational and Applied Mathematics, 37(2), 1537–1549. https://doi.org/10.1007/s40314-016-0411-z

    Article  MathSciNet  MATH  Google Scholar 

  17. Jagadeesh, H., Joshi, R., & Rao, M. (2021). Group secret-key generation using algebraic rings in wireless networks. IEEE Transactions on Vehicular Technology, 70(2), 1538–1553. https://doi.org/10.1109/TVT.2021.3054031

    Article  Google Scholar 

  18. Fika, P., Mitrouli, M., & Roupa, P. (2014). Estimates for the bilinear form Xt A−Y with applications to linear algebra problems. Electronic Transactions on Numerical Analysis, 43, 70–89.

    MathSciNet  MATH  Google Scholar 

  19. Garg, A., Makam, V., Oliveira, R., & Wigderson, A. (2019). Wigderson, Search problems in algebraic complexity, GCT, and hardness of generator for invariant rings. arXiv:1910.01251.

  20. Ibrar, M., Wang, L., Muntean, G. M., Shah, N., Akbar, A., & Ibrahim, K. (2021). SOSW: Scalable and optimal nearsighted location selection for fog node deployment and routing in SDN-based wireless networks for IoT systems. Annals of Telecommunications, 76(5), 331–341. https://doi.org/10.1007/s12243-021-00845-z

    Article  Google Scholar 

  21. Wang, W., Li, Y., Yu, T., Huang, B, & Yang, W. Q. (2021). The optimization design of wireless network router efficiency based on multi-attribute decision-making model. In 2021 International conference on digital society and intelligent systems (DSInS). IEEE. https://doi.org/10.1109/DSInS54396.2021.9670616

  22. Duan, G. (2021). Application of computer network multimedia technology in the teaching reform of advanced algebra. The international conference on cyber security intelligence and analytics. Springer, Cham. https://doi.org/10.1007/978-3-030-69999-4_89

    Article  Google Scholar 

  23. Hoang, T. M. (2021). A Study on Anomaly Data Traffic Detection Method for Wireless Sensor Networks. The international conference on intelligent systems & networks. Springer, Singapore. https://doi.org/10.1007/978-981-16-2094-2_52

    Article  Google Scholar 

  24. Swain, R. R., Khilar, P. M., & Dash, T. (2019). Neural network based automated detection of link failures in wireless sensor networks and extension to a study on the detection of disjoint nodes. Journal of Ambient Intelligence and Humanized Computing, 10(2), 593–610. https://doi.org/10.1007/s12652-018-0709-3

    Article  Google Scholar 

  25. Megalingam, R. K., Tantravahi, S., Tammana, H. S. S. K., Puram, H. S. R., & Ganta, S. (2021). Robot operating system integrated sensing system and forward kinematics of a robot manipulator of a rescue robot. In 2021 International Conference on Intelligent Technologies (CONIT). IEEE. https://doi.org/10.1109/CONIT51480.2021.9498567

  26. Giannini, J. A., Richard, D., Manning, M. L., & Lerner, E. (2021). Bond-space operator disentangles quasilocalized and phononic modes in structural glasses. Physical Review E, 104(4), 044905. https://doi.org/10.1103/PhysRevE.104.044905

    Article  Google Scholar 

  27. Khalid, W., Yu, H., Do, D. T., Kaleem, Z., & Noh, S. (2021). RIS-aided physical layer security with full-duplex jamming in underlay D2D networks. IEEE Access, 9, 99667–99679. https://doi.org/10.1109/ACCESS.2021.3095852

    Article  Google Scholar 

Download references

Acknowledgements

None.

Author information

Authors and Affiliations

  1. Department of Mathematics, University College of Engineering, Bharathidasan Institute of Technology (BIT) Campus, Anna University, Tiruchirapalli, Tamil Nadu, 620 024, India

    Kalaiselvi Sundaram

  2. Department of Mathematics, University College of Engineering Panruti (UCEP), 607 106, Panruti, Tamil Nadu, India

    Seenivasan Velupillai

Authors
  1. Kalaiselvi Sundaram
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Seenivasan Velupillai
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Kalaiselvi Sundaram.

Ethics declarations

Conflict of interest

The authors declare that they have no potential conflict of interest.

Ethical Approval

All applicable institutional and/or national guidelines for the care and use of animals were followed.

Informed Consent

For this type of study formal consent is not required.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sundaram, K., Velupillai, S. Linear Algebraic Theory for Designing the Bus Topology to Enhance the Data Transmission Process. Wireless Pers Commun 126, 401–420 (2022). https://doi.org/10.1007/s11277-022-09751-6

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11277-022-09751-6

Keywords