Performance Analysis of Distributed Reconfigurable Intelligent Surface Aided NOMA Systems

Abstract

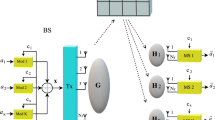
This paper investigates the performance of spatially distributed reconfigurable intelligent surface (RIS)-aided non-orthogonal multiple access (NOMA) systems over Rician fading channels, where the spatial locations of multiple RISs are modeled by invoking the stochastic geometry. Accurate and asymptotic closed-form expressions in terms of the outage probability and ergodic rate are derived based on an optimal selection scheme. Accordingly, diversity orders are obtained to gain more insights on the considered network. It is revealed by simulations that the outage behaviour of distributed RIS-NOMA system outperforms that of RIS-aided orthogonal multiple access (OMA) and conventional multiple relays-aided NOMA counterparts, and the ergodic rate of distributed RIS-NOMA is superior to that of distributed RIS-OMA in the low signal-to-noise ratio region. Additionally, the performance enhancements through more densely deployed of RISs and/or more reflecting elements of RIS as well as the increased Rician factors are corroborated by the simulations.

Appendix A: Proof of Theorem 1

In order to derive the OP of user f, we first calculate the CDF ${F_{{\gamma _f}}}\left( x \right)$. Based on ${\gamma _f} = {\max _{m \in \Psi }}\left\{ {{\gamma _{m,f}}} \right\}$, we have

$$\begin{aligned} {P_f} = \Pr \left( {{\gamma _f}< {\gamma _{t{h_f}}}} \right) = \prod \limits _{m = 1}^M {\Pr \left( {{\gamma _{m,f}} < {\gamma _{t{h_f}}}} \right) }. \end{aligned}$$

(A1)

Note that for the Rician variable $\vert {{h_{\eta ,n}}} \vert$, we have ${{\mathbb {E}}}\left( {\vert {{h_{\eta ,n}}} \vert } \right) = \sqrt{\frac{\pi }{{4\left( {K + 1} \right) }}} {\mathrm{{L}}_{\frac{1}{2}}}\left( { - K} \right)$. Let ${\zeta _n} = \vert {{h_{{rm}f,n}}} \vert \vert {{h_{s{rm},n}}} \vert$. Due to the fact that ${\textbf{h}_{srm}}$ and ${\textbf{h}_{rmf}}$ are independent and identically distributed, the mean and the variance of ${\zeta _n}$ can be, respectively, calculated as

$$\begin{aligned} \mu = {{\mathbb {E}}}\left\{ {\vert {{h_{{rm}f,n}}} \vert \vert {{h_{s{rm},n}}} \vert } \right\} = \frac{\pi }{{4\sqrt{\left( {{K_1} + 1} \right) \left( {{K_2} + 1} \right) } }}{\mathrm{{L}}_{\frac{1}{2}}}\left( { - {K_1}} \right) {\mathrm{{L}}_{\frac{1}{2}}}\left( { - {K_2}} \right) , \end{aligned}$$

(A2)

$$\begin{aligned} {\sigma ^2} ={{\mathbb {D}}}\left\{ {\vert {{h_{{rm}f,n}}} \vert \vert {{h_{s{rm},n}}} \vert } \right\} = 1 - \frac{{{\pi ^2}}}{{16\left( {{K_1} + 1} \right) \!\left( {{K_2} + 1} \right) }}\!{\left( {{\mathrm{{L}}_{\frac{1}{2}}}\left( { - {K_1}} \right) {\mathrm{{L}}_{\frac{1}{2}}}\left( { - {K_2}} \right) } \right) ^2}. \end{aligned}$$

(A3)

It is worth noting that the Rician distribution spans the range from Rayleigh fading ($K=0$) to no fading (constant amplitude) ($K=\infty$) [22], and when $K_1=K_2=0$, the mean and the variance of the cascade Rayleigh fading channel are written as $\mu = {\pi {\pi 4}}$ and ${\sigma ^2} = 1 - {{{\pi ^2}}{16}}$.

Define ${\zeta _N} = \sum \nolimits _{n = 1}^N {{\zeta _n}}$, since the PDF of ${\zeta _N}$ has a single maximum and a fast decaying tail, which can be tightly approximated by the first term of a Laguerre expansion as [23]

$$\begin{aligned} {f_{{\zeta _N}}}\left( x \right) = \frac{{{x^a}}}{{{b^{a + 1}}\Gamma \left( {a + 1} \right) }}\exp \left( { - \frac{x}{b}} \right) , \end{aligned}$$

(A4)

where $a = \frac{{N{\mu ^2}}}{{{\sigma ^2}}} - 1$ and $b = \frac{{{\sigma ^2}}}{\mu }$. Accordingly, the CDF of ${\zeta _N}$ can be expressed as

$$\begin{aligned} {F_{{\zeta _N}}}\left( x \right) = \frac{1}{{\Gamma \left( {a + 1} \right) }}\gamma \left( {a + 1,\frac{x}{b}} \right) . \end{aligned}$$

(A5)

Let ${X_m} = \zeta _N^2d_{{rm}f}^{ - \alpha }d_{sf}^{ - \alpha }$, we have

$$\begin{aligned} \Pr \left( {{\gamma _{m,f}}< {\gamma _{t{h_f}}}} \right) = \Pr \left( {{X_m} < \frac{{{\gamma _{t{h_f}}}}}{{\left( {{a_f} - {a_n}{\gamma _{t{h_f}}}} \right) \rho }}} \right) . \end{aligned}$$

(A6)

Furthermore, the CDF of ${X_m}$ can be given by

$$\begin{aligned} {F_{{X_m}}}\left( x \right) = \frac{2}{{{R^2}}}\int _0^R {\frac{1}{{\Gamma \left( {a + 1} \right) }}\gamma \left( {a + 1,\frac{{\sqrt{x{r^\alpha }d_{sf}^\alpha } }}{b}} \right) } rdr. \end{aligned}$$

(A7)

By utilizing the Gaussian-Chebyshev quadrature, Eq. (A.7) can be rewritten as

$$\begin{aligned} {F_{{X_m}}}\left( x \right) \approx \frac{\pi }{{2W}}\sum \limits _{w = 1}^W {\sqrt{1 - \theta _w^2} \left( {{\theta _w} + 1} \right) \frac{{\gamma \left( {a + 1,\frac{{\sqrt{x{c_w}} }}{b}} \right) }}{{\Gamma \left( {a + 1} \right) }}}. \end{aligned}$$

(A8)

Substituting Eqs. (A.8) into (A.1), we obtain Eq. (9). This completes the proof.