Skip to main content
Springer Nature Link
Log in

A General Sufficient Condition of Four Positive Solutions of the P3P Problem

  • Published:
Journal of Computer Science and Technology Aims and scope Submit manuscript

Abstract

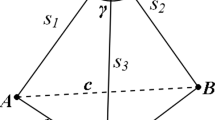
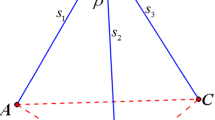
In this paper, it is proved that, given 3 control points A, B and C, if the camera's optical center O lies on one of the three planes perpendicular to the plane ABC and going through one of the three altitudes of the triangle ABC, and additionally its projection on the plane ABC is within the circumscribed circle of the triangle, that is, O is within the so-called “danger cylinder”, then the corresponding P3P problem O, (ABC) must have 4 positive solutions. This result is purely geometrical, and more instructive. It can bring some new insight into a better understanding of multiple-solution problem in the P$n$P problem, and could be used as some theoretical guide to arrange control points in real applications.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Fishler M A, Bolles R C. Random sample consensus: A paradigm for model fitting with applications to image analysis and automated cartography. Comm. ACM, 1981, 24(6): 381–395.

    MathSciNet  Google Scholar 

  2. Haralick R M, Lee C, Ottenberg K, Nölle M. Analysis and solutions of the three point perspective pose estimation problem. In Proc. IEEE Conf. Computer Vision and Pattern Recognition, Maui, Hawaii, 1991, pp.592–598.

  3. Horaud R, Conio B, Leboulleux O. An analytic solution for the perspective 4-point problem. In Proc. Conf. Computer Vision, Graphics, Image Processing, 1989, 47: 33–44.

  4. Wolfe W J, Mathis D, Sklair C W, Magee M. The perspective view of three points. IEEE Trans. Pattern Analysis and Machine Intelligence, 1991, 13(1): 66–73.

    Article  Google Scholar 

  5. Su C, Xu Y Q, Li H, Liu S Q. Necessary and sufficient condition of positive root number of perspective-three-point problem. Chinese J. Computers, 1998, 21(12): 1084–1095. (in Chinese)

    MathSciNet  Google Scholar 

  6. Quan L, Lan Z D. Linear N-point camera pose determination. IEEE Trans. Pattern Analysis and Machine Intelligence, 1999, 21(8): 774–780.

    Google Scholar 

  7. Dhome M, Richetin M, Lapreste J T, Rives G. Determination of the attitude of 3-D objects from a single perspective view. IEEE Trans. Pattern Analysis and Machine Intelligence, 1989, 11(12): 1265–1278.

    Article  Google Scholar 

  8. Abidi M A, Chandra T. A new efficient and direct solution for pose estimation using quadrangular targets: Algorithm and evaluation. IEEE Trans. Pattern Analysis and Machine Intelligence, 1995, 17(5): 534–538.

    Article  Google Scholar 

  9. Gao X S, Hou X R, Tang J L et al. Complete Solution classification for the perspective-three-point problem. IEEE Trans. Pattern Analysis and Machine Intelligence, 2003, 25(8): 930–943.

    Google Scholar 

  10. Fiore P D. Efficient linear solution of exterior orientation. IEEE Trans. Pattern Analysis and Machine Intelligence, 2001, 23(2): 140–148.

    Article  MathSciNet  Google Scholar 

  11. Ansar A, Daniilidis K. Linear pose estimation from points and lines. IEEE Trans. Pattern Analysis and Machine Intelligence, 2003, 25(5): 578–589.

    Article  Google Scholar 

  12. Abdel-Aziz Y I, Karara H M. Direct linear transformation into object space coordinates in close-range photogrammetry. In Proc. Symp. Close-Range Photogrammetry, 1971, pp.1–18.

  13. Thompson E H. Space resection: Failure cases. Photogrammetric Record, X, 1966, 27(X): 201–204.

  14. Hartley R I, Zisserman A. Multiple View Geometry in Computer Vision. Cambridge University Press, 2000.

Download references

Author information

Authors and Affiliations

  1. National Laboratory of Pattern Recognition, Institute of Automation, Chinese Academy of Sciences, Beijing, 100080, P.R. China

    Cai-Xia Zhang & Zhan-Yi Hu

Authors
  1. Cai-Xia Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Zhan-Yi Hu
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Cai-Xia Zhang.

Additional information

Supported by the National Natural Science Foundation of China under Grant Nos.60375006 and 60475009.

Cai-Xia Zhang received the B.S. and M.S. degrees from Shandong University, China, in 1999 and 2002 respectively. She is currently a Ph.D. candidate of National Laboratory of Pattern Recognition, Institute of Automation, Chinese Academy of Sciences. Her current research interests include computer vision, multiple view geometry, and image processing.

Zhan-Yi Hu received the B.S. degree in automation from the North China University of Technology in 1985, the Ph.D. degree (Docteur d'Etat) in computer science from the University of Liege, Belgium, in 1993. Since 1993, he has been with Institute of Automation, the Chinese Academy of Sciences. From May 1997 to May 1998, he was a visiting scholar to the Chinese University of Hong Kong. Dr. Hu now is a research professor of computer vision, a member of the Executive Expert Committee of the Chinese National High Technology R&D Program. His current research interests are in robot vision, including camera calibration, 3D reconstruction, and vision guided robot navigation. Dr. Hu has published more than 70 peer-reviewed papers on major national and international journals.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zhang, CX., Hu, ZY. A General Sufficient Condition of Four Positive Solutions of the P3P Problem. J Comput Sci Technol 20, 836–842 (2005). https://doi.org/10.1007/s11390-005-0836-0

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11390-005-0836-0

Keywords