Skip to main content
Springer Nature Link
Log in

Finite volume element method with Lagrangian cubic functions

  • Published:
Journal of Systems Science and Complexity Aims and scope Submit manuscript

Abstract

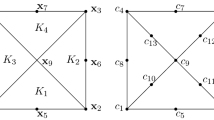
This paper establishes a new finite volume element scheme for Poisson equation on triangular meshes. The trial function space is taken as Lagrangian cubic finite element space on triangular partition, and the test function space is defined as piecewise constant space on dual partition. Under some weak condition about the triangular meshes, the authors prove that the stiffness matrix is uniformly positive definite and convergence rate to be O(h 3) in H 1-norm. Some numerical experiments confirm the theoretical considerations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

We’re sorry, something doesn't seem to be working properly.

Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Similar content being viewed by others

References

  1. R. H. Li, Z. Y. Chen, and W. Wu, Generalized Difference Methods for Differential Equations: Numerical Analysis of Finite Volume Element Methods, Marcel Dekker, New York, 2000.

    MATH  Google Scholar 

  2. R. E. Bank and D. J. Rose, Some error estimates for the box method, SIAM J. Numer. Anal., 1987, 24: 777–787.

    Article  MathSciNet  MATH  Google Scholar 

  3. Z. Cai, On the finite volume element method, Numer. Math., 1991, 58: 713–735.

    Article  MathSciNet  MATH  Google Scholar 

  4. Z. Cai, J. Douglas, and M. Park, Development and analysis of higher order finite volume methods over rectangles for elliptic equations, Adv. Comput. Math., 2003, 19: 3–33.

    Article  MathSciNet  MATH  Google Scholar 

  5. P. Chatzipantelidis, Finite volume methods for elliptic PDE’s: A new approach, M2AN, 2002, 36: 307–324.

    Article  MathSciNet  MATH  Google Scholar 

  6. S. H. Chou, Analysis and convergence of a covolume method for the generalized Stokes problem, Math. Comp., 1997, 36: 85–104.

    Article  Google Scholar 

  7. Z. Chen, C. He, and B. Wu, High order finite volume methods for singular perturbation problems, Science in China, Series A, 2008, 51(8): 1391–1400.

    Article  MathSciNet  MATH  Google Scholar 

  8. Z. Y. Chen, R. H. Li, and A. H. Zhou, A note on the optimal L 2-estimate of the finite volume element method, Adv. Comput. Math., 2002, 16: 291–302.

    Article  MathSciNet  MATH  Google Scholar 

  9. L. Chen, A new class of high order finite volume methods for second order elliptic equations, SIAM Journal on Numerical Analysis, 2010, 47(6): 4021–4043.

    Article  MathSciNet  MATH  Google Scholar 

  10. Z. Cai, J. Mandel, and S. Mccormick, The finite volume element for diffusion equations on general triangulations, SIAM J. Numer. Anal., 1991, 28: 392–402.

    Article  MathSciNet  MATH  Google Scholar 

  11. Z. Y. Chen, The error estimate of generalized difference method of 3rd-order Hermite type for elliptic partial differential equations, Northeastern Math. J., 1992, 8: 127–135.

    MATH  Google Scholar 

  12. R. E. Ewing, T. Lin, and Y. Lin, On the accuracy of finite volume element method based on piecewise linear polynomials, SIAM J. Numer. Anal., 2002, 39: 1865–1888.

    Article  MathSciNet  MATH  Google Scholar 

  13. Y. H. Li and R. H. Li, Generalized difference methods on arbitrary quadrilateral networks, J. Comput. Math., 1999, 17(6): 653–672.

    MathSciNet  MATH  Google Scholar 

  14. R. H. Li and P. Q. Zhu, Generalized difference methods for second order elliptic partial differential equations (I) — Triangle grids, Numer. Math. J. Chinese Universities, 1982, 2: 140–152.

    Google Scholar 

  15. T. Schmidt, Box schemes on quadrilateral meshes, Computing, 1993, 51: 271–292.

    Article  MathSciNet  MATH  Google Scholar 

  16. Y. H. Li and J. L. Lü, L 2-estimate of the finite volume element methods on quadrilateral meshes, Adv. Comput. Math., 2010, 33(2): 129–148.

    Article  MathSciNet  MATH  Google Scholar 

  17. J. C. Xu and Q. S. Zou, Analysis of linear and quadratic simplicial finite volume methods for elliptic equations, Numer. Math., 2009, 111(3): 469–492.

    Article  MathSciNet  MATH  Google Scholar 

  18. M. Yang, A second-order finite volume element method on quadrilateral meshes for elliptic equations, M2AN, 2007, 40: 1053–1067.

    Article  Google Scholar 

  19. M. Z. Tian and Z. Y. Chen, Quadratic element generalized difference methods for elliptic equations, Numer. Math. J. Chinese Universities, 1991, 2: 99–113.

    MathSciNet  Google Scholar 

  20. F. Liebau, The finite volume element method with quadratic basis functions, Computing, 1996, 57: 281–299.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Mathematics, Jilin University, Changchun, 130012, China

    Yuqiong Ding & Yonghai Li

Authors
  1. Yuqiong Ding
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yonghai Li
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yuqiong Ding.

Additional information

This research is supported by the ‘985’ programme of Jilin University, the National Natural Science Foundation of China under Grant Nos. 10971082 and 11076014.

This paper was recommended for publication by Editor Ningning YAN.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Ding, Y., Li, Y. Finite volume element method with Lagrangian cubic functions. J Syst Sci Complex 24, 991–1006 (2011). https://doi.org/10.1007/s11424-011-9113-1

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11424-011-9113-1

Key words