Abstract
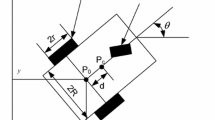
The article proposes a nonlinear optimal (H-infinity) control approach for the model of a tracked robotic vehicle. The kinematic model of such a tracked vehicle takes into account slippage effects due to the contact of the tracks with the ground. To solve the related control problem, the dynamic model of the vehicle undergoes first approximate linearization around a temporary operating point which is updated at each iteration of the control algorithm. The linearization process relies on first-order Taylor series expansion and on the computation of the Jacobian matrices of the state-space model of the vehicle. For the approximately linearized description of the tracked vehicle a stabilizing H-infinity feedback controller is designed. To compute the controller’s feedback gains an algebraic Riccati equation is solved at each time-step of the control method. The stability properties of the control scheme are proven through Lyapunov analysis. It is also demonstrated that the control method retains the advantages of linear optimal control, that is fast and accurate tracking of reference setpoints under moderate variations of the control inputs.
Similar content being viewed by others
References
Rigatos G and Busawon K, Robotic Manipulators and Vehicles: Control, Estimation and Filtering, Springer, New York, 2018.
Endo D, Okada Y, Nagatani K, et al., Path following control for tracked vehicles based on slip-compensating odometry, IEEE IROS 2007, The IEEE 2007 Conference on Intelligent Robots and Systems, San Diego, California, Dec. 2007.
Guo Y, Yu L, and Xu J, Robust finite-time trajectory tracking control of wheeled mobile robots with parametric uncertainties and disturbances, Journal of Systems Science and Complexity, 2019, 32(6): 1358–1374.
Yamauchi G, Nagatani K, and Fujino K, Slip-compensated odometry for tracked vehicle on loose and weak slope, RoboMech Journal, 2017, 4(27): 1–11.
Seron J, Martinez J L, Mandow A, et al., Automation of the arm-aided climbing maneuver for tracked mobile manipulators, IEEE Transactions on Industrial Electronics, 2014, 61(7): 3638–3647.
Martinez J L, Mandow A, Morales J, et al., Approximating Kinematics for tracked mobile robots, The International Journal of Robotics Research, 2005, 24(10): 867–878.
Yamauchi G, Suzuki D, and Nagatani K, Online slip parameter estimation for tracked vehicle odometry on loose slope, IEEE SSSR 2016, IEEE 2016 International Symposium on Safety, Security, and Rescue Robotics (SSRR) EPFL, Lausanne, Switzerland, 2016.
Cook J T, Ray L E, and Lever J H, Dynamics model for mobility optimization and control of off-road tractor convoys, IEEE ACC 2016, IEEE 2016 American Control Conference, Boston, Massachusets, 2016.
Sebastian B and Ben-Tzvi P, Active disturbance rejection control for handling slip in tracked vehicle locomotion, ASME Journal of Mechanisms and Robotics, 2019, 11: 1–12.
Tao W, Ou Y, and Feng H, Research on dynamics and stability in the stairs-climbing of a tracked mobile robot, International Journal of Advanced Robotic Systems, 2012, 9(146): 1–9.
Zou T, Angeles J, and Hassani F, Dynamic modeling and trajectory tracking control of unmanned tracked vehicles, Robotic and Autonomous Systems, 2018, 110: 102–111.
Liu Y and Liu G, Track-stair interaction analysis and online tipover prediction for a self-reconfigurable tracked mobile robot climbing stairs, IEEE/ASME Transactions on Mechatronics, 2009, 14(9): 528–538.
Liu Y and Liu G, Modeling of tracked mobile manipulators with consideration of track-terrain and vehicle-manipulator interactions, Robotics and Autonomous Systems, 2009, 57: 1065–1074.
Saeed P, Lawrence P D, Lowe D G, et al., An autonomous excavator with vision-based track slippage control, IEEE Transactions on Control Systems Technology, 2014, 13(1): 67–84.
Fan X, Singh S, Oppolzer F, et al., Integrated planning and control of large tracked vehicles in open terrain, 2010 IEEE Intl. Conference on Robotics and Automation, Anchorage, Alaska, USA, 2010.
Chen K, Kamezaki M, Katano T, et al., Compound locomotion control system combining crawling and walking for multi-crawler multi-arm robot to adapt unstructured and unknown terrain, Robomech Journal, 2018, 5(2): 1–17.
Zweiri Y H, Seneviratne L D, and Althoefer K, Modelling and control of an unmanned excavator vehicle, modelling and control of an unmanned excavator vehicle, Proc. IMeche, Part I: Journal of Systems and Control Engineering, Sage Publications, 2016, 227: 259–274.
Mai T and Wang Y, Adaptive force/motion control system based on recurrent fuzzy wavelet CMAC neural networks for condenser cleaning crawler-type mobile manipulator robot, IEEE Transactions on Control Systems Technology, 2014, 22(5): 1973–1982.
Sheng W, Chen H, and Xi N, Navigating a miniature crawler robot for engineered structure inspection, IEEE Transactions on Automation Science and Engineering, 2008, 5(2): 368–375.
Takai R, Yang L, and Noguchi N, Development of a crawler-type robot tractor using RTK-GPS and IMU, Engineering in Agriculture, Environment and Food, 2014, 7: 143–147.
Peng J, Yang Z, Wang Y, et al., Robust adaptive motion/force control scheme for crawler-type mobile manipulator with nonholonomic constraint based on sliding mode control approach, ISA Transactions, Elsevier, 2019.
Liu Y and Liu G, Interaction analysis and online tip-over avoidance for a reconfigurable tracked mobile modular manipulator negotiating slopes, IEEE/ASME Transactions on Mechatronics, 2010, 15(4): 623–635.
Zou M, Bai H, Wang Y, et al., Mechanical design of a self-adaptive transformable tracked robot for cable tunnel inspection, Proc. of 2016 IEEE Intl. Conf. on Mechatronics and Automation, Harbin, China, 2016.
Iossaqui J G, Camino J F, and Zampieri D E, A nonlinear control design for tracked robots with longitudinal slip, Proc. of the 18th World Congress The International Federation of Automatic Control, Milano, Italy, 2011.
Jiao J, Sun L, Kang W, et al., A sliding parameter estimation method based on UKF for agricultural tracked robot, IEEE ICSAI 2014, IEEE 2nd Intl. Conf. on Systems and Informatics, Shanghai, China, 2014.
Zhou L, Wang Z, Mok Y H, et al., Steering stability control for four-motor distributed drive high speed tracked vehicles, IEEE Access, 2020, 8: 94968–94983.
Wu J, Wang G, Zhou H, et al., Sutdy on electromechanical performance of steering of the electric articulated tracked vehicle, Journnal of Mechanical Science and Technology, 2019, 33(7): 3171–3185.
Ziye Z, Haiou L, Huiyan C, et al., Kinematics-aware model predictive control for autonomous high-speed tracked vehicles under the off-road conditions, Mechanical Systems and Signal Processing, 2019, 123: 333–350.
Li Z, Jing X, Sun B, et al., Autonomous navigation of a tracked mobile robot with novel passive bio-inspired suspension, IEEE/ASME Transactions on Mechatronics, 2009, 14(5): 528–538.
Rigatos G G and Tzafestas S G, Extended kalman filtering for fuzzy modelling and multi-sensor fusion, Mathematical and Computer Modelling of Dynamical Systems, Taylor & Francis, 2007, 13: 251–266.
Basseville M and Nikiforov I, Detection of Abrupt Changes: Theory and Applications, Prentice-Hall, Upper Saddle River, NJ, 1993.
Rigatos G and Zhang Q, Fuzzy model validation using the local statistical approach, Fuzzy Sets and Systems, 2009, 60(7): 882–904.
Rigatos G and Siano P, A new nonlinear H-infinity feedback control approach to the problem of autonomous robot navigation, Journal of Intelligent Industrial Systems, 2015, 1(3): 179–186.
Rigatos G, Siano P, Wira P, et al., Nonlinear H-infinity feedback control for asynchronous motors of electric trains, Journal of Intelligent Industrial Systems, 2015, 1(3): 85–98.
Rigatos G, Nonlinear Control and Filtering Using Differential Flatness Approaches: Applications to Electromechanicsl Systems, Springer, New York, 2015.
Rigatos G, Modelling and Control for Intelligent Industrial Systems: Adaptive Algorithms in Robotcs and Industrial Engineering, Springer, New York, 2011.
Rigatos G, Intelligent Renewable Energy Systems: Modelling and Control, Springer, New York, 2017.
Toussaint G T, Basar T, and Bullo F, H∞ optimal tracking control techniques for nonlinear underactuated systems, Proc. IEEE CDC 2000, 39th IEEE Conference on Decision and Control, Sydney Australia, 2000.
Yang T, Sun N, Chen H, et al., Neural network-based adaptive antiswing control of an underactuated ship-mounted crane with roll motions and input dead zones, IEEE Transactions on Neural Networks and Learning Systems, 2019, 31(3): 901–914.
Sun N, Fu Y, Yang T, et al., Nonlinear motion control of complicated dual rotary crane systems without velocity feedback: Design, analysis, and hardware experiments, IEEE Transactions on Automation Science and Engineering, 2020, 17(2): 1017–1029.
Author information
Authors and Affiliations
Corresponding author
Additional information
This research was supported by the Research “Advances in Applied Nonlinear Optimal Control” under Grant No. 6065.
This paper was recommended for publication by Editor HE Wei.
Rights and permissions
About this article
Cite this article
Rigatos, G. A Nonlinear Optimal Control Approach for Tracked Mobile Robots. J Syst Sci Complex 34, 1279–1300 (2021). https://doi.org/10.1007/s11424-021-0036-1
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11424-021-0036-1