Abstract
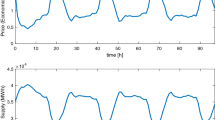
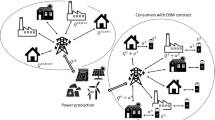
This paper solves a mean-field type hierarchical optimal control problem in electricity market. The authors consider n − 1 prosumers and one producer. The ith prosumer, for 1 < i < n, is a leader of the (i − 1)th prosumer and is a follower of the (i + 1)th prosumer. The first player (agent) is the follower at the bottom whereas the nth is the leader at the top. The problem is described by a linear jump-diffusion system of conditional mean-field type, where the conditioning is with respect to common noise, and a quadratic cost functional involving, the square of the conditional expectation of the controls of the agents. The authors provide a semi-explicit solution of the corresponding mean-field-type hierarchical control problem with common noise. Finally, the authors illustrate the obtained result via a numerical example with two different scenarios.
Similar content being viewed by others
References
Stackelberg H V, The theory of the market economy, Trans. by Peacock A J, London, William Hodge, Originally Published as Grundlagen der Theoretischen Volkswirtschaftlehre, 1948.
Simaan M and Cruz J B, On the stackelberg strategy in nonzero-sum games, Journal of Optimization Theory and Applications, 1973, 11(5): 533–555.
Bagchi A and Basar T, Stackelberg strategies in linear-quadratic stochastic differential games, Journal of Optimization Theory and Applications, 1981, 35(3): 443–464.
Pan L and Yong J, A differential game with multi-level of hierarchy, Journal of Mathematical Analysis and Applications, 1991, 161(2): 522–544.
Li N and Yu Z, Forward-backward stochastic differential equations and linear-quadratic generalized stackelberg games, SIAM Journal on Control and Optimization, 2018, 56(6): 4148–4180.
Simaan M and Cruz J, A stackelberg solution for games with many players, IEEE Transactions on Automatic Control, 1973, 18(3): 322–324.
Cruz J, Leader-follower strategies for multilevel systems, IEEE Transactions on Automatic Control, 1978, 23(2): 244–255.
Gardner B and Cruz J, Feedback stackelberg strategy for m-level hierarchical games, IEEE Transactions on Automatic Control, 1978, 23(3): 489–491.
Basar T and Selbuz H, Closed-loop stackelberg strategies with applications in the optimal control of multilevel systems, IEEE Transactions on Automatic Control, 1979, 24(2): 166–179.
Andersson D and Djehiche B, A maximum principle for sdes of mean-field type, Applied Mathematics & Optimization, 2011, 63(2011): 341–356.
Buckdahn R, Djehiche B, and Li J, A general stochastic maximum principle for SDEs of mean-field type, Applied Mathematics & Optimization, 2011, 64(2011): 197–216.
Tembine H, Risk-sensitive mean-field-type games with lp-norm drifts, Automatica, 2015, 59(2015): 224–237.
Tcheukam A and Tembine H, Mean-field-type games for distributed power networks in presence of prosumers, 2016 28th Chinese Control and Decision Conference (CCDC), 2016, 446–451.
Djehiche B, Tcheukam A, and Tembine H, Mean-field-type games in engineering, AIMS Electronics and Electrical Engineering, 2017, 1(1): 18–73.
Tembine H, Mean-field-type games, AIMS Math, 2017, 2(4): 706–735.
Duncan T and Tembine H, Linear-quadratic mean-field-type games: A direct method, Games, 2018, 9(7): 1–18.
Barreiro-Gomez J, Duncan T E, and Tembine H, Linear-quadratic mean-field-type games: Jump-diffusion process with regime switching, IEEE Transactions on Automatic Control, 2019, 64(10): 4329–4336.
Barreiro-Gomez J, Duncan T E, and Tembine H, Linear-quadratic mean-field-type games with multiple input constraints, IEEE Control Systems Letters, 2019, 3(3): 511–516.
Djehiche B, Barreiro-Gomez J, and Tembine H, Price dynamics for electricity in smart grid via mean-field-type games, Dynamic Games and Applications, 2020, 10(2020): 798–818.
Evans G C, A simple theory of competition, American Mathematical Monthly, 1922, 29(10): 371–380.
Ross C F, A mathematical theory of competition, American Journal of Mathematics, 1925, 47(3): 163–175.
Hobbs B F and Pang J, Nash-cournot equilibria in electric power markets with piecewise linear demand functions and joint constraints, Operations Research, 2007, 55(1): 113–127.
Metzler C, Hobbs B F, and Pang J, Nash-cournot equilibria in power markets on a linearized dc network with arbitrage: Formulations and properties, Networks and Spatial Economics, 2003, 3(2): 123–150.
Hobbs B E, Linear complementarity models of nash-cournot competition in bilateral and poolco power markets, IEEE Transactions on Power Systems, 2001, 16(2): 194–202.
Willems B, Modeling cournot competition in an electricity market with transmission constraints, The Energy Journal, 2002, 23(3): 95–125.
Hogan W, A market power model with strategic interaction in electricity networks, The Energy Journal, 1997, 18(4): 107–141.
Wanga B and Huang M, Mean field production output control with sticky prices: Nash and social solutions, Automatica, 2019, 100(2019): 90–98.
Jovanovic B, Selection and the evolution of industry, Econometrica, 1982, 50(3): 649–670.
Acknowledgements
We gratefully acknowledge support from Tamkeen under the NYU Abu Dhabi Research Institute grant CG002, and U.S. Air Force Office of Scientific Research under Grant No. FA9550-17-1-0259.
Author information
Authors and Affiliations
Corresponding authors
Additional information
This paper was recommended for publication by Editor GUO Jin.
Rights and permissions
About this article
Cite this article
Frihi, Z.E.O., Choutri, S.E., Barreiro-Gomez, J. et al. Hierarchical Mean-Field Type Control of Price Dynamics for Electricity in Smart Grid. J Syst Sci Complex 35, 1–17 (2022). https://doi.org/10.1007/s11424-021-0176-3
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11424-021-0176-3