Abstract
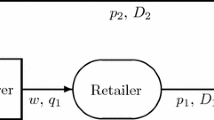
Meca, et al. (2004) studied a class of inventory games which arise when a group of retailers who observe demand for a common good decide to cooperate and make joint orders. In this paper, the authors extend their model to the situation where the manufacturer provides the retailers with a price discount on purchases in excess of a certain order quantity. The authors define the corresponding inventory game with quantity discount, and show that the inventory game has a nonempty core. Then, the authors propose a core allocation rule, the demand-proportionality rule, and characterize it by introducing efficiency, the retailer splitting property and continuity.
Similar content being viewed by others
References
Harris F W, Operations and cost, Factory Management Series, Chicago, Shaw & Co, 1915, 48–52.
Hax A C and Candea D, Production and Inventory Management, Prentice-Hall, Englewood Cliffs, NJ, 1984.
Tersine R J, Principles of Inventory and Materials Management, Elsevier, Amsterdam, 1994.
Meca A, Timmer J, García-Jurado I, et al., Inventory games, European Journal of Operational Research, 2004, 156(1): 127–139.
Dror M and Hartman B C, Survey of cooperative inventory games and extensions, Journal of the Operational Research Society, 2011, 62(4): 565–580.
Fiestras-Janeiro M G, García-Jurado I, Meca A, et al., Cost allocation in inventory transportation systems, Top, 2012, 20(2): 397–410.
Fiestras-Janeiro M G, García-Jurado I, Meca A, et al., Cooperation on capacitated inventory situations with fixed holding costs, European Journal of Operational Research, 2015, 241(3): 719–726.
Meca A, García-Jurado I, and Borm P, Cooperation and competition in inventory games, Mathematical Methods of Operations Research, 2003, 57(3): 481–493.
Meca A, A core-allocation family for generalized holding cost games, Mathematical Methods of Operations Research, 2007, 65(3): 499–517.
Li J, Feng H, and Zeng Y, Inventory games with permissible delay in payments, European Journal of Operational Research, 2014, 234(3): 694–700.
Fiestras-Janeiro M G, García-Jurado I, Meca A, et al., Centralized inventory in a farming community, Journal of Business Economics, 2014, 84(7): 983–997.
Guardiola L A, Meca A, and Puerto J, Production-inventory games and PMAS-games: Characterizations of the Owen point, Mathematical Social Sciences, 2008, 56(1): 96–108.
Guardiola L A, Meca A, and Puerto J, Production-inventory games: A new class of totally balanced combinatorial optimization games, Games and Economic Behavior, 2009, 65(1): 205–219.
O'Neill B, A problem of rights arbitration from the Talmud, Mathematical Social Sciences, 1982, 2(4): 345–371.
Young H P, Okada N, and Hashimoto T, Cost allocation in water resources development, Water Resources Research, 1982, 18(3): 463–475.
Shapley L S, A value for n-person games, Contributions to the Theory of Games II (Eds. by Kuhn H W and Tucker A W), Princeton University Press, Princeton, 1953, 307–317.
Author information
Authors and Affiliations
Corresponding author
Additional information
This research was supported by the National Natural Science Foundation of China under Grant Nos. 72071159, 71671140 and 71601156.
This paper was recommended for publication by Editor CAO Zhigang.
Rights and permissions
About this article
Cite this article
Li, W., Xu, G. & Su, J. Inventory Games with Quantity Discount. J Syst Sci Complex 34, 1538–1554 (2021). https://doi.org/10.1007/s11424-021-9235-z
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11424-021-9235-z