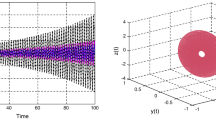
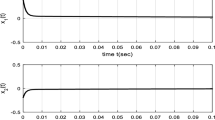
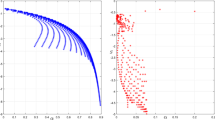
Abstract
This article focuses on the positivity and the asymptotic stability of fractional-order linear time-delay systems (FOLTDSs) which are composed of N (N ≥ 2) subsystems. Firstly, a sufficient and necessary condition is given to ensure the positivity of FOLTDSs. The solutions of the studied systems are obtained by using the Laplace transform method, and it can be observed that the positivity of FOLTDSs is completely determined by the series of matrices and independent of the magnitude of time-delays. Secondly, a theorem is given to prove the asymptotic stability of positive FOLTDSs. By considering the monotonicity and asymptotic properties of systems with constant time-delay, it is further shown that the asymptotic stability of positive FOLTDSs is independent of the time-delay. Next, a state-feedback controller, whose gain matrix is derived by resolving a linear programming question, is designed such that the state variables of the systems are nonnegative and asymptotically convergent. When the order of the FOLTDSs is greater than one, by utilizing a proposed property of Caputo derivative, a sufficient condition for the positivity of FOLTDS is presented. Finally, simulation examples are presented to verify the validity and practicability of the theoretical analysis.
Similar content being viewed by others
References
He J H and Ji F Y, Two-scale mathematics and fractional calculus for thermodynamics, Thermal Science, 2019, 23(4): 2131–2133.
Tarasov V E, On history of mathematical economics: Application of fractional calculus, Mathematics, 2019, 7(6): 1–28.
Wang K L, Wang K J, and He C H, Physical insight of local fractional calculus and its application to fractional Kdv—Burgers—Kuramoto equation, Fractals, 2019, 27(7): 1950122.
Mo L and Guo S, Consensus of linear multi-agent systems with persistent disturbances via distributed output feedback, Journal of Systems Science & Complexity, 2019, 32(3): 835–845.
Dinh C H, Mai V T, and Duong T H, New results on stability and stabilization of delayed Caputo fractional order systems with convex polytopic uncertainties, Journal of Systems Science & Complexity, 2020, 33(3): 563–583.
Ozarslan R, Ercan A, and Bas E, Novel fractional models compatible with real world problems, Fractal and Fractional, 2019, 3(2): 15.
D’Elia M, Du Q, Glusa C, et al., Numerical methods for nonlocal and fractional models, Acta Numerica, 2020, DOI: https://doi.org/10.1017/s096249292000001X.
Rajchakit G, Chanthorn P, Niezabitowski M, et al., Impulsive effects on stability and passivity analysis of memristor-based fractional-order competitive neural networks, Neurocomputing, 2020, 417: 290–301.
Meng B, Wang X, Zhang Z, et al., Necessary and sufficient conditions for normalization and sliding mode control of singular fractional-order systems with uncertainties, Science China Information Sciences, 2020, 63(5): 1–10.
Tolba M F, Saleh H, Mohammad B, et al., Enhanced FPGA realization of the fractional-order derivative and application to a variable-order chaotic system, Nonlinear Dynamics, 2020, 33: 584–603.
Chanthorn P, Rajchakit G, Ramalingam S, et al., Robust dissipativity analysis of hopfield-type complex-valued neural networks with time-varying delays and linear fractional uncertainties, Mathematics, 2020, 8(4): 595–616.
Rajchakit G, Kaewmesri P, Chanthorn P, et al., Global stability analysis of fractional-order quaternion-valued bidirectional associative memory neural networks, Mathematics, 2020, 8(5): 801–827.
Podlubny I, Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications, Elsevier, Amsterdam, 1998.
Veeresha P and Prakasha D G, Solution for fractional generalized Zakharov equations with Mittag-Leffler function, Results in Engineering, 2020, 5: 100085.
Anjum N and He J H, Laplace transform: Making the variational iteration method easier, Applied Mathematics Letters, 2019, 92: 134–138.
Wang H, Xiao M, Tao B, et al., Improving dynamics of integer-order small-world network models under fractional-order PD control, Science China Information Sciences, 2020, 63(1): 1–13.
Mesdoui F, Shawagfeh N, and Ouannas A, Global synchronization of fractional-order and integer-order n component reaction diffusion systems: Application to biochemical models, Mathematical Methods in the Applied Sciences, 2021, 44(1): 1003–1012.
Haider S S and Rehman M U, Construction of fixed point operators for nonlinear difference equations of non integer order with impulses, Fractional Calculus and Applied Analysis, 2020, 23(3): 886–907.
Zhang Q H and Lu J G, Positive real lemmas for singular fractional-order systems: The 0 < α < 1 case, IET Control Theory & Applications, 2020, 14(18): 2805–2813.
Xu J, Jiang J, and O’Regan D, Positive solutions for a class of p-Laplacian hadamard fractional-order three-point boundary value problems, Mathematics, 2020, 8(3): 1–13.
Asgharnia A, Jamali A, Shahnazi R, et al., Load mitigation of a class of 5-MW wind turbine with RBF neural network based fractional-order PID controller, ISA Transactions, 2020, 96: 272–286.
Marinangeli L, Alijani F, and HosseinNia S H, Fractional-order positive position feedback compensator for active vibration control of a smart composite plate, Journal of Sound and Vibration, 2018, 412: 1–16.
Behinfaraz R, Ghaemi S, and Khanmohammadi S, Adaptive synchronization of new fractional-order chaotic systems with fractional adaption laws based on risk analysis, Mathematical Methods in the Applied Sciences, 2019, 42(6): 1772–1785.
Liu J, Yin K, Yang D, et al., Stability analysis of switched positive systems with an impulse interval, Circuits, Systems, and Signal Processing, 2020, 40(2): 1005–1020.
Binid A, Elarbi Achhab M, and Laabissi M, Positive observers for linear positive systems in a Hilbert lattice space, IMA Journal of Mathematical Control and Information, 2021, 38(1): 143–158.
Yavuz M and Sene N, Stability analysis and numerical computation of the fractional predator-prey model with the harvesting rate, Fractal and Fractional, 2020, 4(3): 35.
Ji X, Lu J, Lou J, et al., A unified criterion for global exponential stability of quaternion-valued neural networks with hybrid impulses, International Journal of Robust and Nonlinear Control, 2020, 30(18): 8098–8116.
Wang G, Pei K, and Chen Y, Stability analysis of nonlinear hadamard fractional differential system, Journal of the Franklin Institute, 2019, 356(12): 6538–6546.
Khan H, Gómez-Aguilar J, Khan A, et al., Stability analysis for fractional order advection-reaction diffusion system, Physica A: Statistical Mechanics and Its Applications, 2019, 521: 737–751.
Ahmad M, Zada A, and Alzabut J, Stability analysis of a nonlinear coupled implicit switched singular fractional differential system with p-Laplacian, Advances in Difference Equations, 2019, 2019(1): 436.
Zeng H B, Liu X G, and Wang W, A generalized free-matrix-based integral inequality for stability analysis of time-varying delay systems, Applied Mathematics and Computation, 2019, 354: 1–8.
Wang Z and Liu X, Exponential stability of impulsive complex-valued neural networks with time delay, Mathematics and Computers in Simulation, 2019, 156: 143–157.
Liu H, Pan Y, Cao J, et al., Positivity and stability analysis for fractional-order delayed systems: A TS fuzzy model approach, IEEE Transactions on Fuzzy Systems, 2020, 29(4): 927–939.
Trinh H, Huong D C, and Nahavandi S, Observer design for positive fractional-order interconnected time-delay systems, Transactions of the Institute of Measurement and Control, 2019, 41(2): 378–391.
Shen J and Lam J, Stability and performance analysis for positive fractional-order systems with time-varying delays, IEEE Transactions on Automatic Control, 2015, 61(9): 2676–2681.
Liu H, Wang H, Cao J, et al., Composite learning adaptive sliding mode control of fractional-order nonlinear systems with actuator faults, Journal of the Franklin Institute, 2019, 356(916): 9580–9599.
Bourafa S, Abdelouahab M, and Moussaoui A, On some extended Routh-Hurwitz conditions for fractional-order autonomous systems of order α ∈ (0, 2) and their applications to some population dynamic models, Chaos, Solitons & Fractals, 2020, 133: 109623.
Li C and Deng W, Remarks on fractional derivatives, Applied Mathematics and Computation, 2007, 187(2): 777–784.
Le H V and Chu K T, Robust control of positive fractional-order interconnected systems with heterogeneous delays, Asian Journal of Control, 2019, 21(1): 596–608.
Wu H and Su H, Positive edge consensus of networked systems with input saturation, ISA Transactions, 2020, 96: 210–217.
Iqbal Z, Ahmed N, Baleanu D, et al., Positivity and boundedness preserving numerical algorithm for the solution of fractional nonlinear epidemic model of HIV/AIDS transmission, Chaos, Solitons & Fractals, 2020, 134: 109706.
Kaczorek T, Fractional positive continuous-time linear systems and their reachability, International Journal of Applied Mathematics and Computer Science, 2008, 18(2): 223–228.
Liu Y and Su H, Some necessary and sufficient conditions for containment of second-order multi-agent systems with sampled position data, Neurocomputing, 2020, 378: 228–237.
Shahri E S A, Alfi A, and Machado J T, Lyapunov method for the stability analysis of uncertain fractional-order systems under input saturation, Applied Mathematical Modelling, 2020, 81: 663–672.
Li H, Cheng J, Li H B, et al., Stability analysis of a fractional-order linear system described by the Caputo-Fabrizio derivative, Mathematics, 2019, 7(2): 200.
Chen L, Li T, Chen Y, et al., Robust passivity and feedback passification of a class of uncertain fractional-order linear systems, International Journal of Systems Science, 2019, 50(6): 1149–1162.
Berman A and Plemmons R J, Nonnegative Matrices in the Mathematical Sciences, SIAM, Philadelphia, USA, 1994, 9.
Tavazoei M S, On monotonic and nonmonotonic step responses in fractional order systems, IEEE Transactions on Circuits and Systems II: Express Briefs, 2011, 58(7): 447–451.
Author information
Authors and Affiliations
Corresponding author
Additional information
Data Availability
All data sets generated for this study are included in the manuscript.
Conflicts of Interest
The authors declare that they have no conflicts of interest.
This work was supported by the National Natural Science Foundation of China under Grant Nos. 61967001, 12062004, and 11771263, the Innovation Project of Guangxi University for Nationalities Graduate Education (gxun-chxps201908 and gxun-chxkc201903), and the Guangxi Natural Science Foundation (2019GXNS-FAA185007 and 2020GXNSFAA297240).
Rights and permissions
About this article
Cite this article
Hao, Y., Huang, C., Cao, J. et al. Positivity and Stability of Fractional-Order Linear Time-Delay Systems. J Syst Sci Complex 35, 2181–2207 (2022). https://doi.org/10.1007/s11424-022-1018-7
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11424-022-1018-7