Skip to main content
SpringerLink
Log in

Almost Disturbance Decoupling for HOFA Nonlinear Systems with Strict-Feedback Form

  • Published:
Journal of Systems Science and Complexity Aims and scope Submit manuscript

Abstract

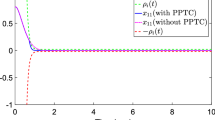
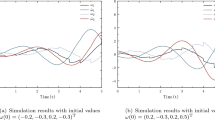
The article is devoted to the almost disturbance decoupling problem for high-order fully actuated (HOFA) nonlinear systems with strict-feedback form. Using the full-actuation feature of high-order fully actuated systems and Lyapunov stability theory, a state feedback control law and virtual control laws are designed. The unknown disturbances are handled by almost disturbance decoupling (ADD) method. Finally, the effectiveness of the control strategy is verified by stability analysis and simulation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Duan G R, High-order system approaches: Part I. Fully actuated systems and parametric designs, Acta Automatica Sinica, 2020, 46(7): 1333–1345.

    MATH  Google Scholar 

  2. Duan G R, High-order fully actuated system approaches: Part I. Models and basic procedure, International Journal of Systems Science, 2021, 52(2): 422–435.

    Article  MathSciNet  Google Scholar 

  3. Duan G R, High-order system approaches: Part II. Controllability and full-actuation, Acta Automatica Sinica, 2020, 46(8): 1571–1581.

    MATH  Google Scholar 

  4. Duan G R, High-order fully-actuated system approaches: Part II. Generalized strict-feedback systems, International Journal of Systems Science, 2021, 52(3): 437–454.

    Article  MathSciNet  Google Scholar 

  5. Duan G R, High-order fully-actuated system approaches: Part III. Robust control and high-order backstepping, International Journal of Systems Science, 2021, 52(5): 952–971.

    Article  MathSciNet  Google Scholar 

  6. Duan G R, High-order fully actuated system approaches: Part IV. Adaptive control and high-order backstepping, International Journal of Systems Science, 2021, 52(5): 972–989.

    Article  MathSciNet  Google Scholar 

  7. Duan G R, High-order fully actuated system approaches: Part V. Robust adaptive control, International Journal of Systems Science, 2021, 52(10): 2129–2143.

    Article  MathSciNet  Google Scholar 

  8. Duan G R, High-order fully-actuated system approaches: Part VI. Disturbance attenuation and decoupling, International Journal of Systems Science, 2021, 52(10): 2161–2181.

    Article  MathSciNet  Google Scholar 

  9. Duan G R, High-order fully-actuated system approaches: Part VII. Controllability, stabilisability and parametric designs, International Journal of Systems Science, 2021, 52(14): 3091–3114.

    Article  MathSciNet  Google Scholar 

  10. Duan G R, High-order fully-actuated system approaches: Part VIII. Optimal control with application in spacecraft attitude stabilisation, International Journal of Systems Science, 2021, 53(1): 54–73.

    Article  MathSciNet  Google Scholar 

  11. Duan G R, High-order fully-actuated system approaches: Part IX. Generalised PID control and model reference tracking, International Journal of Systems Science, 2021, DOI: https://doi.org/10.1080/00207721.2021.1970277.

  12. Duan G R, High-order fully-actuated system approaches: Part X. Basics of discrete-time systems, International Journal of Systems Science, 2021, DOI: https://doi.org/10.1080/00207721.2021.1975848.

  13. Duan G R, High-order system approaches — III. Super-observability and observer design, Acta Automatica Sinica, 2020, 46(9): 1885–1895.

    MATH  Google Scholar 

  14. Willems J C and Commault C, Disturbance decoupling by measurement feedback with stability or pole placement, SIAM Journal on Control & Optimization, 1981, 19(4): 490–504.

    Article  MathSciNet  Google Scholar 

  15. Basile G and Marro G, Controlled and Conditioned Invariants in Linear System Theory, Prentice Hall, Upper Saddle River, New Jersey, 1992.

    MATH  Google Scholar 

  16. Nijmeijer H and Schaft V A, Nonlinear Dynamical Control Systems, Springer, New York, 175, 1990.

    Book  Google Scholar 

  17. Willems J, Almost invariant subspaces: An approach to high gain feedback design, Part I: Almost controlled invariant subspaces, IEEE Transactions on Automatic Control, 1981, 26(1): 235–252.

    Article  MathSciNet  Google Scholar 

  18. Marino R, Respondek W, and Schaft A, Almost disturbance decoupling for single-input singleoutput nonlinear systems, IEEE Transactions on Automatic Control, 1989, 34(9): 1013–1017.

    Article  MathSciNet  Google Scholar 

  19. Willand S and Willems J C, Almost disturbance decoupling with internal stability, IEEE Transactions on Automatic Control, 1989, 34: 277–286.

    Article  MathSciNet  Google Scholar 

  20. Fradkov A L, Miroshnik L V, and Nikiforov V O, Nonlinear and adaptive control of complex systems, Nonlinear and Adaptive Control of Complex Systems, 1999.

  21. Chen B, Tong S C, and Liu X P, Fuzzy approximate disturbance decoupling of MIMO nonlinear systems by ackstepping approach, Fuzzy Sets and Systems, 2007, 158(10): 1097–1125.

    Article  MathSciNet  Google Scholar 

  22. Tan Q Q, Li T, Song Z Y, et al., Output tracking control for generalized high-order nonlinear system with almost disturbance decoupling, International Journal of Control Automation and Systems, 2007, 15(6): 2570–2578.

    Article  Google Scholar 

  23. Fu Y M, Wu A G, and Duan G R, Almost disturbance decoupling for a class of inherently nonlinear systems, International Journal of Control Automation and Systems, 2009, 7(2): 325–330.

    Article  Google Scholar 

  24. Zhang Z Y, Liu X P, Liu Y, et al., Fixed-time almost disturbance decoupling of nonlinear time-varying systems with multiple disturbances and dead-zone input, Information Sciences: An International Journal, 2018, 450: 267–283.

    Article  MathSciNet  Google Scholar 

  25. Liu X P, Liu Y, Zhou Y C, et al., The finite-time almost disturbance decoupling for nonlinear systems, International Journal of Systems Science, 2018, 49(9–12): 2243–2256.

    Article  MathSciNet  Google Scholar 

  26. Liu X P, Zhao Y J, Wang C Y, et al., Almost disturbance decoupling for a class of fractional-order nonlinear systems with zero dynamics, Complexity, 2020, 1: 1–13.

    Google Scholar 

  27. Chen C C, Chien T L, and Wu C J, Simultaneous tracking and almost disturbance decoupling for nonlinear systems with uncertainties, Journal of the Chinese Institute of Engineers, Series A, 2004, 27(1): 23–24.

    Article  Google Scholar 

  28. Liu X P, Jutan A, and Jutan S, Almost disturbance decoupling of MIMO nonlinear systems and application to chemical processes, Automatica, 2004, 40(3): 465–471.

    Article  MathSciNet  Google Scholar 

  29. Yang L and Jing Y W, Practical finite-time almost disturbance decoupling strategy for uncertain nonlinear systems, Nonlinear Dynamics, 2019, 95(3): 117–128.

    Article  Google Scholar 

  30. Kang S, Nagamune R, Yan H, et al., Almost disturbance decoupling force control for the electrohydraulic load simulator with mechanical backlash, Mechanical Systems and Signal Processing, 2020, 135: 106400.

    Article  Google Scholar 

  31. Chu H Y, Qian C J, Yang J Q, et al., Almost disturbance decoupling for a class of nonlinear systems via sampled-data output feedback control, International Journal of Robust and Nonlinear Control, 2016, 26(10): 2201–2215.

    Article  MathSciNet  Google Scholar 

  32. Yang J, Chen W H, Li S H, et al., Static disturbance-to-output decoupling for nonlinear systems with arbitrary disturbance relative degree, International Journal of Robust & Nonlinear Control, 2013, 23(5), DOI: https://doi.org/10.1002/rnc.1850.

  33. Li C Y and Wang W, Fuzzy almost disturbance decoupling for MIMO nonlinear uncertain systems based on high-gain observer, Neurocomputing, 2013, 111: 104–114.

    Article  Google Scholar 

  34. Zhong Z Z and Wang J C, Looper-tension almost disturbance decoupling control for hot strip finishing mill based on feedback linearization, IEEE Transactions on Industrial Electronics, 2011, 58(8): 3668–3679.

    Article  Google Scholar 

  35. Chu H Y, Qian C J, Yang J Q, et al., Almost disturbance decoupling for a class of nonlinear systems via sampled-data output feedback control, International Journal of Robust and Nonlinear Control, 2016, 16(10): 2201–2215.

    Article  MathSciNet  Google Scholar 

  36. Isidori A and Astolfi A, Disturbance attenuation and H∞-control via measurement feedback in nonlinear systems, IEEE Transactions on Automatic Control, 1992, 37(9): 1283–1293.

    Article  MathSciNet  Google Scholar 

  37. Zhou K M, Doyle J C, and Glover K, Robust and Optimal Control, Prentice-Hall, Inc., Upper Saddle River, New Jersey, 1996.

    MATH  Google Scholar 

  38. Duan G R, Generalized Sylvester Equations: Unified Parametric Solutions, CRC Press, Boca Raton, 2015.

    Book  Google Scholar 

  39. Chen W H, Disturbance observer based control for nonlinear systems, IEEE/ASME Transactions on Mechatronics, 2004, 9(4): 706–710.

    Article  Google Scholar 

Download references

Author information

Author notes

  1. Joint first authors, contributed equally.

Authors and Affiliations

  1. School of Information and Electrical Engineering, Shandong Jianzhu University, Jinan, 250101, China

    Na Wang, Xiaoping Liu, Cungen Liu & Yucheng Zhou

  2. Faculty of Engineering, Lakehead University, Thunder Bay, ON, P7B 5E1, Canada

    Xiaoping Liu

  3. The Department of Mathematics, Bohai University, Jinzhou, 121013, China

    Huanqing Wang

Authors
  1. Na Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xiaoping Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Cungen Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Huanqing Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Yucheng Zhou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Cungen Liu.

Additional information

This research was supported by the Taishan Scholar Project of Shandong Province of China under Grant Nos. 2015162 and tsqn201812093.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, N., Liu, X., Liu, C. et al. Almost Disturbance Decoupling for HOFA Nonlinear Systems with Strict-Feedback Form. J Syst Sci Complex 35, 481–501 (2022). https://doi.org/10.1007/s11424-022-2017-4

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11424-022-2017-4

Keywords

Search

Navigation