Abstract
During the electrical stimulation of a uniform, long, and straight nerve axon, the electric field oriented parallel to the axon has been widely accepted as the major field component that activates the axon. Recent experimental evidence has shown that the electric field oriented transverse to the axon is also sufficient to activate the axon, by inducing a transmembrane potential within the axon. The transverse field can be generated by a time-varying magnetic field via electromagnetic induction. The aim of this study was to investigate the factors that influence the transmembrane potential induced by a transverse field during magnetic stimulation. Using an unmyelinated axon model, we have provided an analytic expression for the transmembrane potential under spatially uniform, time-varying magnetic stimulation. Polarization of the axon was dependent on the properties of the magnetic field (i.e., orientation to the axon, magnitude, and frequency). Polarization of the axon was also dependent on its own geometrical (i.e., radius of the axon and thickness of the membrane) and electrical properties (i.e., conductivities and dielectric permittivities). Therefore, this article provides evidence that aside from optimal coil design, tissue properties may also play an important role in determining the efficacy of axonal activation under magnetic stimulation. The mathematical basis of this conclusion was discussed. The analytic solution can potentially be used to modify the activation function in current cable equations describing magnetic stimulation.






Similar content being viewed by others
References
Abdeen MA, Stuchly MA (1994) Modeling of magnetic field stimulation of bent neurons. IEEE Trans Biomed Eng 41:1092–1095
Amassian VE, Maccabee PJ, Cracco RQ (1989) Focal stimulation of human peripheral nerve with the magnetic coil: a comparison with electrical stimulation. Exp Neurol 103:282–289
Bardou AL, Chesnais JM, Birkui PJ, Govaere MC, Auger PM, Von Euw D, Degonde J (1990) Directional variability of stimulation threshold measurements in isolated guinea pig cardiomyocytes: relationship with orthogonal sequential defibrillating pulses. Pacing Clin Electrophysiol 13:1590–1595
Barker A, Freeston IL, Garnham CW (1990) Measurement of Cortical and peripheral neural membrane time constant in man using nerve stimulation. J Physiol (Lond) 423:66
Basser PJ, Roth BJ (1991) Stimulation of a myelinated nerve axon by electromagnetic induction. Med Biol Eng Comput 29:261–268
Basser PJ, Roth BJ (2000) New currents in electrical stimulation of excitable tissues. Annu Rev Biomed Eng 2:377–397
Basser PJ, Wijesinghe RS, Roth BJ (1992) The activating function for magnetic stimulation derived from a three-dimensional volume conductor model. IEEE Trans Biomed Eng 39:1207–1210
Berthold CH, Rydmark M (1995) Morphology of normal peripheral axon. In: Waxman SG, Kocsis JD, Stys PK (eds) The axon: structure, function, and pathophysiology. Oxford University Press, Oxford
Carbunaru R, Durand DM (1997) Axonal stimulation under MRI magnetic field z gradients: a modeling study. Magn Reson Med 38:750–758
Cros D, Day TJ, Shahani BT (1990) Spatial dispersion of magnetic stimulation in peripheral nerves. Muscle Nerve 13:1076–1082
Di Lazzaro V, Oliviero A, Pilato F, Saturno E, Dileone M, Mazzone P, Insola A, Tonali PA, Rothwell JC (2004) The physiological basis of transcranial motor cortex stimulation in conscious humans. Clin Neurophysiol 115:255–266
Durand DM (2003) Electric field effects in hyperexcitable neural tissue: a review. Radiat Prot Dosim 106:325–331
Esselle KP, Stuchly MA (1994) Quasi-static electric field in a cylindrical volume conductor induced by external coils. IEEE Trans Biomed Eng 41:151–158
Esselle KP, Stuchly MA (1995) Cylindrical tissue model for magnetic field stimulation of neurons: effects of coil geometry. IEEE Trans Biomed Eng 42:934–941
Farkas DL, Korenstein R, Malkin S (1984) Electrophotoluminescence and the electrical properties of the photosynthetic membrane. I. Initial kinetics and the charging capacitance of the membrane. Biophys J 45:363–373
Garnsworthy RK, Gully RL, Kenins P, Westerman RA (1988) Transcutaneous electrical stimulation and the sensation of prickle. J Neurophysiol 59:1116–1127
Ghai RS, Bikson M, Durand DM (2000) Effects of applied electric fields on low-calcium epileptiform activity in the CA1 region of rat hippocampal slices. J Neurophysiol 84:274–280
Gielen FL, Cruts HE, Albers BA, Boon KL, Wallinga-de Jonge W, Boom HB (1986) Model of electrical conductivity of skeletal muscle based on tissue structure. Med Biol Eng Comput 24:34–40
Grumet AE, Wyatt JL Jr, Rizzo JF 3rd (2000) Multi-electrode stimulation and recording in the isolated retina. J Neurosci Methods 101:31–42
Knisley SB, Trayanova N, Aguel F (1999) Roles of electric field and fiber structure in cardiac electric stimulation. Biophys J 77:1404–1417
Konings MK (2007) A new method for spatially selective, non-invasive activation of neurons: concept and computer simulation. Med Biol Eng Comput 45:7–24
Kotnik T, Miklavcic D (2000) Second-order model of membrane electric field induced by alternating external electric fields. IEEE Trans Biomed Eng 47:1074–1081
Kotnik T, Miklavcic D (2006) Theoretical evaluation of voltage inducement on internal membranes of biological cells exposed to electric fields. Biophys J 90:480–491
Kotnik T, Bobanovic F, Miklavcic D (1997) Sensitivity of transmembrane voltage induced by applied electric fields—a theoretical analysis. Bioelectrochem Bioenerg 43:285–291
Kotnik T, Miklavcic D, Slivnik T (1998) Time course of transmembrane voltage induced by time-varying electric field—a method for theoretical analysis and its application. Bioelectrochem Bioenerg 45:3–16
Krasteva VT, Papazov SP, Daskalov IK (2003) Peripheral nerve magnetic stimulation: influence of tissue non-homogeneity. Biomed Eng Online 2:19
Lee DC, Grill WM (2005) Polarization of a spherical cell in a nonuniform extracellular electric field. Ann Biomed Eng 33:603–615
Lontis ER, Nielsen K, Struijk JJ (2009) In vitro magnetic stimulation of pig phrenic nerve with transverse and longitudinal induced electric fields: analysis of the stimulation site. IEEE Trans Biomed Eng 56:500–512
Lu H, Chestek CA, Shaw KM, Chiel HJ (2008) Selective extracellular stimulation of individual neurons in ganglia. J Neural Eng 5:287–309
Maccabee PJ, Amassian VE, Cracco RQ, Eberle LP, Rudell AP (1991) Mechanisms of peripheral nervous system stimulation using the magnetic coil. Electroencephalogr Clin Neurophysiol Suppl 43:344–361
Maccabee PJ, Amassian VE, Eberle LP, Cracco RQ (1993) Magnetic coil stimulation of straight and bent amphibian and mammalian peripheral nerve in vitro: locus of excitation. J Physiol 460:201–219
Mansfield P, Harvey PR (1993) Limits to neural stimulation in echo-planar imaging. Magn Reson Med 29:746–758
McNeal DR (1976) Analysis of a model for excitation of myelinated nerve. IEEE Trans Biomed Eng 23:329–337
Meyerson BA, Linderoth B (2000) Mechanisms of spinal cord stimulation in neuropathic pain. Neurol Res 22:285–292
Miranda PC, Hallett M, Basser PJ (2003) The electric field induced in the brain by magnetic stimulation: a 3-D finite-element analysis of the effect of tissue heterogeneity and anisotropy. IEEE Trans Biomed Eng 50:1074–1085
Nagarajan SS, Durand DM (1995) Analysis of magnetic stimulation of a concentric axon in a nerve bundle. IEEE Trans Biomed Eng 42:926–933
Nagarajan SS, Durand DM (1996) A generalized cable equation for magnetic stimulation of axons. IEEE Trans Biomed Eng 43:304–312
Olney RK, So YT, Goodin DS, Aminoff MJ (1990) A comparison of magnetic and electrical stimulation of peripheral nerves. Muscle Nerve 13:957–963
Polk C, Song JH (1990) Electric fields induced by low frequency magnetic fields in inhomogeneous biological structures that are surrounded by an electric insulator. Bioelectromagnetics 11:235–249
Polson MJ, Barker AT, Freeston IL (1982) Stimulation of nerve trunks with time-varying magnetic fields. Med Biol Eng Comput 20:243–244
Ranck JB Jr (1975) Which elements are excited in electrical stimulation of mammalian central nervous system: a review. Brain Res 98:417–440
Ravazzani P, Ruohonen J, Grandori F, Tognola G (1996) Magnetic stimulation of the nervous system: induced electric field in unbounded, semi-infinite, spherical, and cylindrical media. Ann Biomed Eng 24:606–616
Reilly JP (1989) Peripheral nerve stimulation by induced electric currents: exposure to time-varying magnetic fields. Med Biol Eng Comput 27:101–110
Roth BJ, Basser PJ (1990) A model of the stimulation of a nerve fiber by electromagnetic induction. IEEE Trans Biomed Eng 37:588–597
Roth BJ, Cohen LG, Hallett M, Friauf W, Basser PJ (1990) A theoretical calculation of the electric field induced by magnetic stimulation of a peripheral nerve. Muscle Nerve 13:734–741
Ruohonen J, Panizza M, Nilsson J, Ravazzani P, Grandori F, Tognola G (1996) Transverse-field activation mechanism in magnetic stimulation of peripheral nerves. Electroencephalogr Clin Neurophysiol 101:167–174
Schnabel V, Struijk JJ (1999) Magnetic and electrical stimulation of undulating nerve fibres: a simulation study. Med Biol Eng Comput 37:704–709
Schnabel V, Struijk JJ (2001) Evaluation of the cable model for electrical stimulation of unmyelinated nerve fibers. IEEE Trans Biomed Eng 48:1027–1033
Stratton J (1941) Electromagnetic theory. McGraw-Hill, New York
Struijk JJ, Durand DM (1998) Magnetic peripheral nerve stimulation: axial verses transverse fields. In: Proceedings BMES/EMBS, Atlanta, 469 pp
Struijk JJ, Schnabel V (2001) Difference between electrical and magnetic nerve stimulation: A case for transverse field. Proceedings of the 23rd Annual International Conference of IEEE, pp 885–887
Susil R, Semrov D, Miklavcic D (1998) Electric field induced transmembrane potential depends on cell density and organization. Electro Magnetobiol 17:391–399
Teruel MN, Meyer T (1997) Electroporation-induced formation of individual calcium entry sites in the cell body and processes of adherent cells. Biophys J 73:1785–1796
Tofts PS, Branston NM (1991) The measurement of electric field, and the influence of surface charge, in magnetic stimulation. Electroencephalogr Clin Neurophysiol 81:238–239
Tuday EC, Olree KS, Horch KW (2006) Differential activation of nerve fibers with magnetic stimulation in humans. BMC Neurosci 7:58
Wachner D, Simeonova M, Gimsa J (2002) Estimating the subcellular absorption of electric field energy: equations for an ellipsoidal single shell model. Bioelectrochemistry 56:211–213
While PT, Forbes LK (2004) Electromagnetic fields in the human body due to switched transverse gradient coils in MRI. Phys Med Biol 49:2779–2798
Ye H, Cotic M, Carlen PL (2007) Transmembrane potential induced in a spherical cell model under low-frequency magnetic stimulation. J Neural Eng 4:283–293
Yu H, Zheng C, Wang Y (2005) A new model and improved cable function for representing the activating peripheral nerves by a transverse electric field during magnetic stimulation. Proceedings of the 2nd International IEEE EMBS Conference on Neural Engineering, Arlington, VA
Acknowledgments
This study was supported by CIHR grants to PC and MGF, as a Canadian Heart and Stroke Foundation (HSF) grant to MGF, and a HSF postdoctoral fellowship to Hui Ye. The authors thank Dr Robert Chen and Dr Stiliyan N. Kalitzin for their valuable comments to improve the article.
Author information
Authors and Affiliations
Corresponding author
Appendices
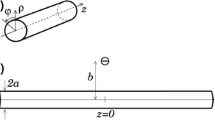
Appendix 1: Expression of vector potential \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {A} \) in cylindrical coordinates (r′, θ′, z′)
In cylindrical coordinates (r′, θ′, z′) centered at O′, we have
The magnetic vector potential\( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {A} \) induced by an external current is
where \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {J} \) is the current density; R is the distance between the current element and the target tissue where \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {A} \) is evaluated; dv is the volume of the element carrying current density \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {J} \); μ is the magnetic conductivity; \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {A} \) is in the direction of -\( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {\theta } ^{\prime} \) and is symmetrical about the O′Z′ axis.
Substituting (15) into (13), we have
Also
where CO is a constant. As the current is symmetrical about the Z′ axis. \( A_{{\theta^{\prime}(r^{\prime} = 0)}} \; = \;0. \) Therefore CO = 0. Thus,
Appendix 2: Coordinate transformation from cylindrical coordinates (r′, θ′, z′) to (r, θ, z) in the computation of the magnetic vector potential \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {A} \)
In cylindrical coordinates (r′, θ′, z′) with origin at o′, from Eq. 19, we know
Here, we have omitted the time factor e jωt to simplify the calculation, which allows us to investigate the “instant” polarization of the axon. The vector potential can be expressed in a Cartesian basis using a matrix transformation
In Fig. 1, since x′ = x − C, y′ = y, z′ = z, the vector potential \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {A} \) can be expressed in (x, y, z) coordinates
Expressed in cylindrical coordinates(r, θ, z)
Appendix 3: Determining unknown coefficients C n , D n in Eq. 11 using boundary conditions (A)–(D)
As V is bounded at r = 0 and r → ∞ and from Eq. 11, we have
Therefore, expressions for the potential distribution in the extracellular media, the membrane, and in the cytoplasm are
We substituted A 0r (Eq. 6) and the \( \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {r} \) components of ∇V in the three regions into (9) to yield the expressions of the normal components of the electric fields in the three regions:
Following boundary condition (A), V is continuous at the extracellular media/membrane (r = R +) and membrane/intracellular cytoplasm interfaces (r = R −),
We then used the boundary condition (B), that the normal components of the current densities are continuous between two different media (Eqs. 1, 2), to obtain the following equations:
We solved (32), (33), (34) and (35) to get the last four unknown coefficients:
Rights and permissions
About this article
Cite this article
Ye, H., Cotic, M., Fehlings, M.G. et al. Transmembrane potential generated by a magnetically induced transverse electric field in a cylindrical axonal model. Med Biol Eng Comput 49, 107–119 (2011). https://doi.org/10.1007/s11517-010-0704-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11517-010-0704-0