Skip to main content
SpringerLink
Log in

Dynamic facility layout problem under competitive environment: a new formulation and some meta-heuristic solution methods

  • Production Management
  • Published:
Production Engineering Aims and scope Submit manuscript

Abstract

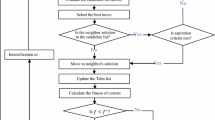
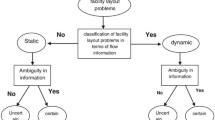
Dynamic facility layout problem (DFLP) considers flow over multiple time periods in an environment where material flow between departments changes over time. These changes render the current facility layout inefficient and could increase material handling costs, which results in a need for dynamic layout models. But a key factor ignored for simplicity in the DFLP literature, is considering the effects of non-cooperating internal and external competitions on the mentioned changes in facility layout over time that sometimes results in main conflict of objectives/goals for these distinct decision makers. To the best our knowledge, the existing special literature did not cover this important assumption. Ignoring this main assumption resulted in the existing monopolistic models for DFLP that cannot satisfy the new needs in the nowadays competitive environments. Therefore, in this paper, a duopoly Bertrand non-cooperative game model is presented to consider the impacts of all conflicting factors on a DFLP for each period and three meta-heuristic algorithms are proposed to obtain Nash equilibrium for this model. The results obtained from solving some numerical examples with these algorithms show that the proposed simulated annealing algorithm is more effective than the others for the competitive dynamic facility layout problem.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19

Similar content being viewed by others

References

  1. Afentakis PA (1989) Loop layout design problem for flexible manufacturing systems. Int J Flex Manuf Syst 1:143–175

    Article  Google Scholar 

  2. Aiello G, Enea M, Galante G (2006) A multi-objective approach on facility layout problem by genetic search algorithm and electre method. Robot Comput Integrat Manuf 22(5–6):447–455

    Article  Google Scholar 

  3. Atashpaz-Gargari E, Lucas C (2007) Imperialist competitive algorithm: an algorithm for optimization inspired by imperialistic competition. In: IEEE congress on evolutionary computation, CEC 2007 (4661–4667)

  4. Balakrishnan J, Jacobs RF, Venkataramanan MA (1992) Solutions for the constrained dynamic facility layout problem. Eur J Oper Res 57:280–286

    Article  MATH  Google Scholar 

  5. Balakrishnan J, Cheng CH, Wong KF (2003) FACOPT: a user friendly FACility layout OPTimization system. Comput Oper Res 30(11):1625–1641

    Article  MATH  Google Scholar 

  6. Balakrishnan J, Cheng CH, Conway DG, Lau CM (2003) A hybrid genetic algorithm for the dynamic plant layout problem. Int J Prod Econ 86(2):107–120

    Article  Google Scholar 

  7. Baykasoglu A, Gindy NNZ (2001) A simulated annealing algorithm for dynamic layout problem. Comput Oper Res 28(14):1403–1426

    Article  MathSciNet  MATH  Google Scholar 

  8. Baykasoglu A, Dereli T, Sabuncu I (2006) An ant colony algorithm for solving budget constrained and unconstrained dynamic facility layout problems. Omega 34(4):385–396

    Article  Google Scholar 

  9. Braglia M, Zanoni S, Zavanella L (2003) Layout design in dynamic environments: strategies and quantitative indices. Int J Prod Res 41(5):995–1016

    Article  MATH  Google Scholar 

  10. Chen GY (2013) A new data structure of solution representation in hybrid ant colony optimization for large dynamic facility layout problems. Int J Prod Econ 142(2):362–371

    Article  Google Scholar 

  11. Emami S, Nookabadi AS (2013) Managing a new multi-objective model for the dynamic facility layout problem. Int J Adv Manuf Technol 68(9–12):2215–2228

    Article  Google Scholar 

  12. Hosseini-Nasab H, Emami L (2013) A hybrid particle swarm optimization for dynamic facility layout problem. Int J Prod Res 51(14):4325

    Article  Google Scholar 

  13. Islier AA (1998) A genetic algorithm approach for multiple criteria facility layout design. Int J Prod Res 36(6):1549–1569

    Article  MATH  Google Scholar 

  14. Kaveh M, Majazi Dalfard V, Amiri S (2014) A new intelligent algorithm for dynamic facility layout problem in state of fuzzy constraints. Neural Comput Appl 24(5):1179–1190

    Article  Google Scholar 

  15. Kheirkhah A, Navidi H, Messi Bidgoli M (2015) Dynamic facility layout problem: a new bilevel formulation and some metaheuristic solution methods. IEEE Trans Eng Manag 62(3):396–410

    Article  Google Scholar 

  16. Lee YH, Lee MH (2002) A shape-based block layout approach to facility layout problems using hybrid genetic algorithm. Comput Ind Eng 42:237–248

    Article  Google Scholar 

  17. McKendall AR, Shang J, Kuppusamy S (2006) Simulated annealing heuristics for the dynamic facility layout problem. Comput Oper Res 33(8):2431–2444

    Article  MathSciNet  MATH  Google Scholar 

  18. Meng G, Heragu SS, Zijm H (2004) Reconfigurable layout problem. Int J Prod Res 42(22):4709–4729

    Article  MATH  Google Scholar 

  19. Navidi H, Bashiri M, MessiBidgoli M (2012) A heuristic approach on the facility layout problem based on game theory. Int J Prod Res 50(6):1512–1527

    Article  Google Scholar 

  20. Page AL (1991) New product development survey: performance and best practices. In: PDMA conference

  21. Pourvaziri H, Naderi B (2014) A hybrid multi-population genetic algorithm for the dynamic facility layout problem’. Appl Soft Comput 24:457–469

    Article  Google Scholar 

  22. Rajabioun R, Atashpaz-Gargari E, Lucas C (2008) Colonial competitive algorithm as a tool for Nash equilibrium point achievement. In: Computational science and its applications–ICCSA, pp 680–695

  23. Ripon KSN, Glette K, Koch D, Hovin M, Torresen J (2011) Genetic algorithm using a modified backward pass heuristic for the dynamic facility layout problem. PALADYN J Behav Robot 2(3):164–174. doi:10.2478/s13230-012-0008-1

    Google Scholar 

  24. Tamashunas V, Labban J, Sly D (1990) Interactive graphics offer an analysis of plant layout and material handling systems. Indust Eng 22(6):38–43

    Google Scholar 

  25. Tompkins JA, White JA, Bozer YA, Frazelle EH, Tanchoco JMA, Trevino J (1996) Facilities planning. Wiley, NewYork, pp 137–285

    Google Scholar 

  26. Tsay AA, Agrawal N (2000) Channel dynamics under price and service competition. Manuf Serv Oper Manag 2:372–391

    Google Scholar 

  27. Uddin MS (2015) Hybrid genetic algorithm and variable neighborhood search for dynamic facility layout problem. Open J Optim 4(4):156–167

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Industrial Engineering Department, Bu-Ali Sina University, Hamedan, Iran

    AmirSaman Kheirkhah & Masume Messi Bidgoli

Authors
  1. AmirSaman Kheirkhah
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Masume Messi Bidgoli
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Masume Messi Bidgoli.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kheirkhah, A., Bidgoli, M.M. Dynamic facility layout problem under competitive environment: a new formulation and some meta-heuristic solution methods. Prod. Eng. Res. Devel. 10, 615–632 (2016). https://doi.org/10.1007/s11740-016-0703-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11740-016-0703-6

Keywords

Search

Navigation