Quantitative analysis of SNR in bilinear time frequency domain

Abstract

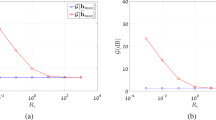
Signal-to-noise ratio (SNR) is an essential concept or quantity on the result of a process or on the output of a filter, which helps us in designing, analyzing or evaluating a system. In this paper, we study SNR for bilinear time–frequency transform (TFT). Firstly, according to the definition of SNR in time domain, we define a proper form for SNR in time–frequency (T–F) plane for bilinear TFT; then, we extract SNR relation in terms of TFT kernel, signals power and noise power in time domain. The extracted relation of SNR for bilinear TFT that can be represented in terms of Wigner–Ville distribution (WVD) shows its dependence on the kernel used in the TFT. Finally, to illustrate the applicability of the proposed SNR, the relations of SNR for several distributions are extracted in the T–F domain, and the variation of SNR versus the noise variance is shown by curves. The results show that the WVD has higher SNR than the Rihaczek, Page, spectrogram and Levin respectively.

Appendix

For a bilinear TFD of a signal as $\rho_{s} \left( {t,f} \right)$, we can write as

$$ \begin{aligned} & \mathop \int \limits_{ - \infty }^{\infty } \langle\rho_{s} \left( {t,f} \right)\rangle {\text{d}}f \\ & = \mathop \int \limits_{ - \infty }^{\infty } \langle W_{s} \left( {t,f} \right)**_{{\left( {t,f} \right)}} \gamma \left( {t,f} \right)\rangle {\text{d}}f \\ & = \mathop {\lim }\limits_{T \to \infty } \frac{1}{T}\mathop \int \limits_{{ - \frac{T}{2}}}^{{\frac{T}{2}}} \mathop \int \limits_{ - \infty }^{\infty } \mathop {\iint }\limits_{ - \infty }^{\infty } W_{s} \left( {t - \tau ,f - \tau^{\prime}} \right)\gamma \left( {\tau ,\tau^{\prime}} \right){\text{d}}\tau {\text{d}}\tau^{{\prime }} {\text{d}}t{\text{d}}f \\ & = \mathop {\iint }\limits_{ - \infty }^{\infty } \gamma \left( {\tau ,\tau^{\prime}} \right)\mathop \int \limits_{ - \infty }^{\infty } \mathop {\lim}\limits_{T \to \infty } \frac{1}{T}\mathop \int \limits_{{ - \frac{T}{2} - \tau }}^{{\frac{T}{2} - \tau }} W_{s} \left( {t,f} \right){\text{d}}t{\text{d}}f{\text{d}}\tau {\text{d}}\tau^{{\prime }} \\ & = \mathop {\iint }\limits_{ - \infty }^{\infty } \gamma \left( {\tau ,\tau^{\prime}} \right)\mathop \int \limits_{ - \infty }^{\infty } W_{s} \left( {t,f} \right){\text{d}}f{\text{d}}\tau {\text{d}}\tau^{{\prime }} \\ & = \mathop \int \limits_{ - \infty }^{\infty } W_{s} \left( {t,f} \right) df \times \mathop {\iint }\limits_{ - \infty }^{\infty } \gamma \left( {\tau ,\tau^{\prime}} \right){\text{d}}\tau {\text{d}}\tau^{{\prime }} . \\ \end{aligned} $$

As $\mathop \int \limits_{ - \infty }^{\infty } W_{s} \left( {t,f} \right) df = \left| {s\left( t \right)} \right|^{2}$

and with changing the variables, we have

$$ \mathop \int \limits_{ - \infty }^{\infty } \langle\rho_{s} \left( {t,f} \right)\rangle df = \langle\left| {s\left( t \right)} \right|^{2}\rangle \mathop {\iint }\limits_{ - \infty }^{\infty } \gamma \left( {t,f} \right){\text{d}}t{\text{d}}f $$