Abstract
Implicational tonoid logics and their relational semantics have been introduced by Yang and Dunn. This paper extends this investigation to implicational partial Galois logics. For this, we first define some implicational partial gaggle logics as special kinds of implicational tonoid logics called “implicational partial Galois logics.” Next, we provide Routley–Meyer-style relational semantics for finitary those logics.
Similar content being viewed by others
Notes
We use “\(\varphi \dashv \vdash \psi \)” as shorthand for \(\varphi \vdash \psi \) and \(\psi \vdash \varphi \).
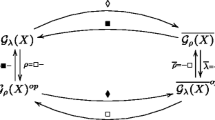
To understand the conditions 1) and 2) in each (1) and (2) using the notations “\(+\),” “−”, and “±,” see the indices of (\(p_{GC^{{\mathcal {A}}}}\)), (\(p_{dGC^{{\mathcal {A}}}}\)), (\(p_{RC^{{\mathcal {A}}}}\)), and (\(p_{dRC^{{\mathcal {A}}}}\)) in Sect. 4.1.
To understand the conditions 1) and 2) using the notations “\(+\),” “−”, and “±,” see the indices of (\(p_{RES^{{\mathcal {A}}}}\)) in Sect. 4.2.
Note that in (\(\sharp ^{n}_{\Diamond +}\)) the index ‘\((\pm )\)’ of \(\sharp \) ambiguously denotes one of isotonicity \(+\) and antitonicity − of all the argument places of \(\sharp \) excepting its i-th one and the notation ‘\(\Vvdash \)’ ambiguously denotes one of their corresponding forcing \(\Vdash \) and non-forcing \(\not \Vdash \). Similarly for (\(\sharp ^{n}_{\Diamond -}\)), (\(\sharp ^{n}_{\Box +}\)), and (\(\sharp ^{n}_{\Box -}\)).
We assume that the canonical relations \(R^{can}\)’s also preserve labeling and tonicity maps, i.e., \(R^{can}_{\Rightarrow }\) and \(R^{can}_{\sharp }\)’s preserve the labels and tonicities of the connectives \(\Rightarrow \) and \(\sharp \)’s given by the labeling and tonicity maps of the logic.
References
Bimbó, K., Dunn, J.M.: Generalized Galois Logics: Relational Semantics of Nonclassical Logical Calculi. CSLI Publications, Stanford (2008)
Cintula, P.: Weakly Implicative (Fuzzy) Logics I: Basic properties. Arch. Math. Log. 45, 673–704 (2006)
Cintula, P., Noguera, C.: A general framework for mathematical fuzzy logic. In: Cintula, P., Hájek, P., Noguera, C. (eds.) Handbook of Mathematical Fuzzy Logic. vol 1, pp. 103–207. College Publications, London (2011)
Dunn, J.M.: Gaggle theory, an abstraction of galois connections and residuation, with applications to negation, implication, and various logical operators. In: Van Eijck, J. (ed.) Logics in AI (JELIA 1990, Amsterdam), Lecture Notes in AI, pp. 31–51. Springer, Berlin (1991)
Dunn, J.M.: Partial gaggles applied to logics with restricted structural rules. In: Schroeder-Heister, P., Do\(\check{s}\)en, K. (eds.) Substructural Logics, pp. 63–108. Clarendon, Oxford (1993)
Dunn, J.M., Hardegree, G.: Algebraic Methods in Philosophical Logic. Oxford University Press, Oxford (2001)
Galatos, N., Jipsen, P., Kowalski, T., Ono, H.: Residuated Lattices: An Algebraic Glimpse at Substructural Logics. Elsevier, Amsterdam (2007)
Yang, E., Dunn, J.M.: Implicational tonoid logics: algebraic and relational semantics. Log. Univ. (2021). https://doi.org/10.1007/s11787-021-00288-z
Acknowledgements
I dedicate this manuscript to the late J. Michael Dunn. Actually, it was completed together with the paper “Implicational tonoid logics.” When we submitted the latter one, we planed to submit the former one to a journal after the acceptance of our latter paper. Since this latter one has been accepted for publication in this journal, I submit the former manuscript to the same journal in honor of Mike and the acceptance in the journal Logica Universalis. We wish to acknowledge the helpful comments we received from Katalin Bimbó and Petr Cintula, which certainly improved our paper. The authors also thank Jeonbuk National University and Indiana University for supporting our joint work during the first author’s sabbatical visit. This work (Yang) was supported by the Ministry of Education of the Republic of Korea and the National Research Foundation of Korea (NRF-2019S1A5A2A01034874).
Author information
Authors and Affiliations
Corresponding author
Additional information
To Hyunjung and Sally.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Deceased: J. Michael Dunn.
Rights and permissions
About this article
Cite this article
Yang, E., Dunn, J.M. Implicational Partial Galois Logics: Relational Semantics. Log. Univers. 15, 457–476 (2021). https://doi.org/10.1007/s11787-021-00290-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11787-021-00290-5