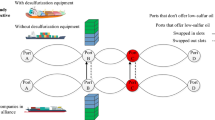
Abstract
The empty equipment repositioning (EER) problem is deemed an inefficiency of the marine industry. However, owing to trade imbalance as the fundamental cause of EER, such problem is inevitable and requires consideration in pricing decisions. Accordingly, this study explores a marine service chain with one carrier and two dominant forwarders providing transportation service between two ports. We built a mathematical model to examine how carrier and forwarders determine pricing decisions with and without EER cost sharing. We find that the smaller one between empty equipment balancing and EER costs determines pricing decisions. We also investigate whether the EER cost-sharing mechanism can coordinate the service chain and subsequently found that the EER cost-sharing mechanism aggravates double marginalization. Then, we design a two-part tariff coordination mechanism to coordinate the service chain. Lastly, we study the effect of EER cost sharing and coordination mechanism on the environment. Interestingly, we find that EER cost-sharing and coordination should be avoided from the perspective of environmental protection.
Similar content being viewed by others
References
Bonnet C, Dubois P (2006) Two-part tariffs versus linear pricing between manufacturers and retailers: empirical tests on differentiated products markets. Working paper 2006, University of Touluse
Bresnahan TF, Reiss PC (1985) Dealer and manufacturer margins. RAND J Econ 16:253–268
Cachon GP, Lariviere MA (2005) Supply chain coordination with revenue sharing: strengths and limitations. Manage Sci 51:30–44
Charnes A, Cooper WW, Kirby MJL, Raike W (1972) Regulatory model’s for pricing and evaluation of transport service. Transp Serv 6:15–31
Chen R, Dong JX, Lee CY (2016) Pricing and competition in a shipping market with waste shipments and empty container repositioning. Transp Res Part B Methodol 43:677–691
Crainic TG, Gendreau M, Dejac P (1993) Dynamic and stochastic-models for the allocation of empty containers. Oper Res 41:102–126
Dejax PJ, Crainic TG (1987) Survey paper-a review of empty flows and fleet management models in freight transportation. Transp Sci 21:227–248
Drewry (2018). Container Market Annual Review and Forecast 2018/19. https://www.drewry.co.uk/maritime-research-products/container-market-annual-review-and-forecast-201819. Accessed 01 Oct 2018
Fransoo JC, Lee CY (2013) The critical role of ocean container transport in global supply chain performance. Prod Oper Manag 22:253–268
Gorman MF (2002) Pricing and market mix optimization in freight transportation. Transp Q 56:135–148
Hueley WJ, Petersen ER (1994) Nonlinear tariffs and freight network equilibrium. Transp Sci 28:236–245
Kuzmicz AK, Pesch E (2019) Approaches to empty container repositioning problems in the context of Eurasian intermodal transportation. Omega 85:194–213
Lariviere MA, Porteus EL (2001) Selling to the newsvendor: an analysis of price-only contracts. Manuf Serv Oper Manag 3:293–305
Li JA, Leung SC, Wu Y (2007) Allocation of empty containers between multi-ports. Eur J Oper Res 182:400–412
Martin MS, Saracho AI (2012) Two part-tariff licensing mechanisms. Working paper 2012, University of the Basque Country NPV/EHU
Padmanabhan V, Png IP (1995) Returns policies: make money by making good. Sloan Manag Rev 37:65–72
Rodrigue JP (2008) The geography of transport systems. Routledge, New York
Song DP, Dong JX (2008) Empty container management in cyclic shipping routes. Marit Econ Logist 10:335–361
Song DP, Dong JX (2013) Long-haul liner service route design with ship deployment and empty container repositioning. Transp Res Part B Methodol 55:188–211
Song DP, Dong JX (2015) Empty container repositioning. In: Lee CY, Meng Q (eds) Handbook of ocean container transport logistics: making global supply chains effective, vol 220. Springer, Heidelberg, pp 163–208
Topaloglu H, Powell W (2007) Incorporating pricing decisions into the stochastic dynamic fleet management problem. Transp Sci 41:281–301
Tsay AA, Agrawal N (2000) Channel dynamics under price and service competition. Manuf Serv Oper Manag 2:372–391
Wang SA (2013) Essential elements in tactical planning models for container liner shipping. Transp Res Part B Methodol 54:84–99
Wu X, Chen H (2015) Supply chain coordination under ramp-type price and effort induced demand considering revenue sharing contract. Int J U-and-E Serv Serv Technol 8:207–218
Xie YY, Liang XY, Ma LJ, Yan HM (2017) Empty container management and coordination in intermodal transport. Eur J Oper Res 257:223–232
Xu L, Govindam K, Bu XZ (2015) Pricing and balancing of the sea–cargo service chain with empty equipment repositioning. Comput Oper Res 54:286–294
Xu XF, Hao J, Deng YR, Wang Y (2017) Design optimization of resource combination for collaborative logistics network under uncertainty. Appl Soft Comput 56:684–691
Xu XF, Wei ZF, Ji Q, Wang CL, Gao GW (2019) Global renewable energy development: Influencing factors, trend predictions and countermeasures. Res Policy 63:101–470
Xu XF, Zheng Y, Yu L (2018) A bi-level optimization model of LRP in collaborative logistics network considered backhaul no-load cost. Soft Comput 22(16):5385–5393
Yu MZ, Fransoo JC, Lee CY (2018) Detention decisions for empty containers in the hinterland transportation system. Transp Res Part B Methodol 110:188–208
Zhang B, Ng CT, Cheng TCE (2014) Multi-period empty container repositioning with stochastic demand and lost sales. J Oper Res Soc 65:302–319
Zhang FQ (2011) Supply chain coordination. Wiley, Hoboken
Zhao Y, Xue QW, Zhang X (2018) Stochastic empty container repositioning problem with CO2 emission considerations for an intermodal transportation system. Sustainability 10:4211
Zheng W, Li B, Song DP (2017) Effects of risk-aversion on competing shipping lines’ pricing strategies with uncertain demands. Transp Res Part B Methodol 104:337–356
Zhou WH, Lee CY (2009) Pricing and competition in a transportation market with empty equipment repositioning. Transp Res Part B Methodol 43:677–691
Funding
This work was supported in part by the National Natural Science Foundation of China [grant numbers 71771185, 71271167, 71832011].
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
Proof of Proposition 1
Due to forwarders lead the Stackelberg game, we first solve decisions problem of the carrier. For Karush–Kuhn–Tucker condition of Eq. (2), we can obtain the carrier’s optimal pricing strategy as follows:
-
1.
when \( {T^C} \leq - {e_A} + \frac{{{\alpha_A}m_A^{FD} - {\alpha_B}m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}} \), we have \( w_A^{FD} = \frac{{{D_A} + {\alpha_A}\left({{c_A} - {e_A}} \right) - {\alpha_A}m_A^{FD}}}{{2{\alpha_A}}} \), \( w_B^{FD} = \frac{{{D_B} + {\alpha_B}\left({{c_B} + {e_A}} \right) - {\alpha_B}m_B^{FD}}}{{2{\alpha_B}}} \), the corresponding realized demands are \( d_A^{FD} = \frac{{{D_A} - {\alpha_A}\left({{c_A} - {e_A}} \right) - {\alpha_A}m_A^{FD}}}{2} \), \( d_B^{FD} = \frac{{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right) - {\alpha_B}m_B^{FD}}}{2} \);
-
2.
when \( - {e_A} + \frac{{{\alpha_A}m_A^{FD} - {\alpha_B}m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}} < {T^C} < {e_B} + \frac{{{\alpha_A}m_A^{FD} - {\alpha_B}m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}} \), we have \( w_A^{FD} = \frac{{{D_A} + {\alpha_A}\left({{c_A} + {T^C}} \right) - {\alpha_A}m_A^{FD} - {\alpha_A}\frac{{{\alpha_A}m_A^{FD} - {\alpha_B}m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}}}}{{2{\alpha_A}}} \), \( w_B^{FD} = \frac{{{D_B} + {\alpha_B}\left({{c_B} - {T^C}} \right) - {\alpha_B}m_B^{FD} + {\alpha_B}\frac{{{\alpha_A}m_A^{FD} - {\alpha_B}m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}}}}{{2{\alpha_B}}} \), the corresponding realized demands are \( d_A^{FD} = \frac{{{D_A} - {\alpha_A}\left({{c_A} + {T^C}} \right) - {\alpha_A}{\alpha_B}\frac{{m_A^{FD} + m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}}}}{2} \), \( d_B^{FD} = \frac{{{D_B} - {\alpha_B}\left({{c_B} - {T^C}} \right) - {\alpha_A}{\alpha_B}\frac{{m_A^{FD} + m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}}}}{2} \);
-
3.
when \( {T^C} \geq {e_B} + \frac{{{\alpha_A}m_A^{FD} - {\alpha_B}m_B^{FD}}}{{{\alpha_A} + {\alpha_B}}} \), we have \( w_A^{FD} = \frac{{{D_A} + {\alpha_A}\left({{c_A} + {e_B}} \right) - {\alpha_A}m_A^{FD}}}{{2{\alpha_A}}} \), \( w_B^{FD} = \frac{{{D_B} + {\alpha_B}\left({{c_B} - {e_B}} \right) - {\alpha_B}m_B^{FD}}}{{2{\alpha_B}}} \), the corresponding realized demands are \( d_A^{FD} = \frac{{{D_A} - {\alpha_A}\left({{c_A} + {e_B}} \right) - {\alpha_A}m_A^{FD}}}{2} \), \( d_B^{FD} = \frac{{{D_B} - {\alpha_B}\left({{c_B} - {e_B}} \right) - {\alpha_B}m_B^{FD}}}{2} \).
Then, substituting the above \( d_A^{FD} \) and \( d_B^{FD} \) into decision problems of the two forwarders respectively. By the first-order condition of Eq. (3), we can obtain \( m_A^{FD*} \) and \( m_B^{FD*} \) in Proposition 1. Substituting \( m_A^{FD *} \) and \( m_B^{FD *} \) of the above carrier’s pricing decision \( w_A^{FD} \) and \( w_B^{FD} \), we have \( w_A^{FD*} \) and \( w_B^{FD*} \) in Proposition 1. Lastly, we substitute \( m_i^{FD*} \) and \( w_i^{FD*} \) (\( i = A,B \))into the realized demand and profit functions which is shown in Table 1 and Table 2.
Proof to Corollary 1
This Corollary can be derived straight forwardly from Proposition 1.
Proof of Proposition 2
Due to forwarders lead the Stackelberg game, we first solve decisions problem of the carrier. By the first-order condition of Eq. (6), we have \( w_A^{FE} = \frac{{{D_A} + {\alpha_A}\left[{{c_A} - \left({1 - {\eta^{FE}}} \right){e_A}} \right] - {\alpha_A}m_A^{FE}}}{{2{\alpha_A}}} \), \( w_B^{FE} = \frac{{{D_B} + {\alpha_B}\left[{{c_B} + \left({1 - {\eta^{FE}}} \right){e_A}} \right] - {\alpha_B}m_B^{FE}}}{{2{\alpha_B}}} \), \( d_A^{FE} = \frac{{{D_A} - {\alpha_A}\left[{{c_A} - \left({1 - {\eta^{FE}}} \right){e_A}} \right] - {\alpha_A}m_A^{FE}}}{2} \), \( d_B^{FE} = \frac{{{D_B} - {\alpha_B}\left[{{c_B} + \left({1 - {\eta^{FE}}} \right){e_A}} \right] - {\alpha_B}m_B^{FE}}}{2} \).
Then, substituting the above \( d_A^{FE} \) and \( d_B^{FE} \) into Eq. (5), by Karush–Kuhn–Tucker condition, we can have
According to the Karush–Kuhn–Tucker condition, four cases are considered as following:
-
1.
when \( \lambda_1^{FE*} > 0 \) and \( \lambda_2^{FE*} > 0 \), no solution;
-
2.
when \( \lambda_1^{FE*} > 0 \) and \( \lambda_2^{FE*} = 0 \), \( {\eta^{FE*}} = 0 \), \( m_A^{FE*} = \frac{{\frac{D_A}{\alpha_A} - \left({{c_A} - {e_A}} \right)}}{2} \), \( m_B^{FE*} = \frac{{\frac{D_B}{\alpha_B} - \left({{c_B} + {e_A}} \right)}}{2} \). Under this case the carrier solely undertakes EER cost, the result is the same to the result of Proposition 1;
-
3.
when \( \lambda_1^{FE*} = 0 \) and \( \lambda_2^{FE*} > 0 \), \( {\eta^{FE*}} = 1 \), \( m_A^{FE*} = \frac{{\frac{D_A}{\alpha_A} - {c_A}}}{2} \), \( m_B^{FE*} = \frac{{\frac{D_B}{\alpha_B} - \left({{c_B} - {e_A}} \right)}}{2} \);
-
4.
when \( \lambda_1^{FE*} = 0 \) and \( \lambda_2^{FE*} = 0 \), \( {\eta^{FE*}} = \frac{{\frac{D_A}{\alpha_A} - \left({{c_A} - {e_A}} \right)}}{{3{e_A}}} \), \( m_A^{FE*} = \frac{{\frac{D_A}{\alpha_A} - \left({{c_A} - {e_A}} \right)}}{3} \), \( m_B^{FE*} = \frac{{\frac{D_B}{\alpha_B} - {c_B}}}{2} + \frac{{\frac{D_A}{\alpha_A} - {c_A}}}{6} + \frac{{\frac{D_A}{\alpha_A} - \left({{c_A} + {e_A}} \right)}}{6} \). Due to \( {\eta^{FE*}} \) is incompatible with \( 0 \leq {\eta^{FE*}} \leq 1 \), this is not the K-T point.
Then, we substitute \( {\eta^{FE*}} = 1 \), \( m_A^{FE*} = \frac{{\frac{D_A}{\alpha_A} - {c_A}}}{2} \) and \( m_B^{FE*} = \frac{{\frac{D_B}{\alpha_B} - \left({{c_B} - {e_A}} \right)}}{2} \) into \( w_A^{FE} \) and \( w_B^{FE} \), we get results shown in Proposition 2. Lastly, we substitute \( m_i^{FD*} \) and \( w_i^{FD*} \) (\( i = A,B \))into the realized demand and profit functions which is shown in Table 1 and Table 2.
Proof to Corollary 2 and 3
These Corollary can be derived straight forwardly from Proposition 2.
Proof of proposition 3
According to Table 1, if the carrier solely undertakes EER cost, when \( {T^C} \leq - {e_A} \) and \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), the optimal profit of the forwarder B is \( \pi_B^{FE*}{|_{{\eta^{FE*}} = 0,d_A^{FE*} < d_B^{FE*}}} = \frac{{{{\left[{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right)} \right]}^2}}}{{8{\alpha_B}}} \) and \( \pi_B^{FE*}{|_{{\eta^{FE*}} = 0,d_A^{FE*} = d_B^{FE*}}} = \frac{{{{\left[{{D_B} - {\alpha_B}\left({{c_B} - {T^C}} \right)} \right]}^2}}}{{8{\alpha_B}}} \) respectively. If the forwarder B undertakes EER cost completely, when \( {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), the optimal profit of the forwarder B is \( \pi_B^{FE*}{|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} = \frac{{{{\left[{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right)} \right]}^2}}}{{8{\alpha_B}}} + \frac{{{D_A} - {\alpha_A}{c_A}}}{4}{e_A} \). Then to compare two feasible EER sharing mechanism, \( {\eta^{FE*}} = 0 \) and \( {\eta^{FE*}} = 1 \), we have to compare optimal profit of forwarder B under both mechanisms in the interval \( {T^C} \leq - {e_A} \) and \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \).
When \( {T^C} \leq - {e_A} \), apparently we have \( \pi_B^{FE*}{|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} > \pi_B^{FE*}{|_{{\eta^{FE*}} = 0,d_A^{FE*} < d_B^{FE*}}} \). When \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), we have
Due to \( \pi_B^{FE*}{|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} - \pi_B^{FE*}{|_{{\eta^{FE*}} = 0,d_A^{FE*} = d_B^{FE*}}} \) is strictly decreasing function of \( {T^C} \) and \( \left({\pi_B^{FE*}{|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} - \pi_B^{FE*}{|_{{\eta^{FE*}} = 0,d_A^{FE*} = d_B^{FE*}}}} \right){|_{{T^C} = - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} > 0 \), we can obtain \( \pi_B^{FE*}{|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} > \pi_B^{FE*}{|_{{\eta^{FE*}} = 0,d_A^{FE*} = d_B^{FE*}}} \). In summary, we get \( \pi_B^{FE*}{|_{{\eta^{FE*}} = 1}} > \pi_B^{FE*}{|_{{\eta^{FE*}} = 0}} \).
Proof of Proposition 4
Similarly to proof of Proposition 3, to investigate the effect of EER cost-sharing mechanism on double marginalization, we compare total optimal profit of the service chain in the interval \( {T^C} \leq - {e_A} \) and \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \).
When \( {T^C} \leq - {e_A} \), we have
When \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), we have
Due to \( \frac{{\partial \left({\left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} - \left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 0,d_A^{FE*} = d_B^{FE*}}}} \right)}}{{\partial {T^C}}} = 0 \) as well as \( \left({\left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} - \left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 0,d_A^{FE*} = d_B^{FE*}}}} \right){|_{{T^C} = - {e_A}}} < 0 \), we obtain \( \left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 1,d_A^{FE*} < d_B^{FE*}}} - \left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 0,d_A^{FE*} = d_B^{FE*}}} < 0 \). In summary, we get \( \left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 1}} < \left({\pi_A^{FE*} + \pi_B^{FE*} + \pi_C^{FE*}} \right){|_{{\eta^{FE*}} = 0}} \).
Proof of Proposition 5
Through solving centralized marine service chain, when \( {T^C} \leq - {e_A} \), the pricing decision is \( p_A^{C*} = \frac{{\frac{D_A}{\alpha_A} + {c_A} - {e_A}}}{2} \) and \( p_B^{C*} = \frac{{\frac{D_B}{\alpha_B} + {c_B} + {e_A}}}{2} \).
For Karush–Kuhn–Tucker condition of Eq. (7), when \( {T^C} \leq - {e_A} \), we have \( \frac{D_A}{\alpha_A} + {c_A} - {e_A} + m_A^{FC} = 2\left({w_A^{FC} + m_A^{FC}} \right) \) and \( \frac{D_B}{\alpha_B} + {c_B} + {e_A} + m_B^{FC} = 2\left({w_B^{FC} + m_B^{FC}} \right) \). The coordination of the service chain requires \( w_A^{FC} + m_A^{FC} = p_A^{C*} \) and \( w_B^{FC} + m_B^{FC} = p_B^{C*} \), which means \( \frac{D_A}{\alpha_A} + {c_A} - {e_A} + m_A^{FC} = \frac{D_A}{\alpha_A} + {c_A} - {e_A} \) and \( \frac{D_B}{\alpha_B} + {c_B} + {e_A} + m_B^{FC} = \frac{D_B}{\alpha_B} + {c_B} + {e_A} \). So we obtain \( m_A^{FC} = 0 \) and \( m_B^{FC} = 0 \).
Similarly, we prove that when \( - {e_A} < {T^C} < {e_B} \) and \( {T^C} \geq {e_B} \), the coordination of the service chain also requires \( m_A^{FC} = 0 \) and \( m_B^{FC} = 0 \).
Proof of Proposition 6
When \( {T^C} \leq - {e_A} \), we substitute \( m_i^{FD*} \) and \( w_i^{FD*} \) (\( i = A,B \))into Eqs. (7) and (8) to solve optimal profit of the carrier and forwarders. We obtain that \( \pi_A^{FC*} = F_A^{FC} \), \( \pi_B^{FC*} = F_B^{FC} \), \( \pi_C^{FC*} = \frac{{{{\left[{{D_A} - {\alpha_A}\left({{c_A} - {e_A}} \right)} \right]}^2}}}{{4{\alpha_A}}} + \frac{{{{\left[{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right)} \right]}^2}}}{{4{\alpha_B}}} - F_A^{FC} - F_B^{FC} \). Then by comparing service chain members’ optimal profit with and without two-part tariff coordination mechanism, we have \( \pi_A^{FC*} - \pi_A^{FD*} = F_A^{FC} - \frac{{{{\left[{{D_A} - {\alpha_A}\left({{c_A} - {e_A}} \right)} \right]}^2}}}{{8{\alpha_A}}} \), \( \pi_B^{FC*} - \pi_B^{FD*} = F_B^{FC} - \frac{{{{\left[{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right)} \right]}^2}}}{{8{\alpha_B}}} \), \( \pi_C^{FC*} - \pi_C^{FD*} = \frac{{3{{\left[{{D_A} - {\alpha_A}\left({{c_A} - {e_A}} \right)} \right]}^2}}}{{16{\alpha_A}}} + \frac{{3{{\left[{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right)} \right]}^2}}}{{16{\alpha_B}}} - F_A^{FC} - F_B^{FC} \).
The feasibility of coordination mechanism requires \( \pi_A^{FC*} - \pi_A^{FD*} \geq 0 \), \( \pi_B^{FC*} - \pi_B^{FD*} \geq 0 \) and \( \pi_C^{FC*} - \pi_C^{FD*} \geq 0 \). According to the above conditions, we have \( F_A^{FC} \geq \frac{{{{\left[{{D_A} - {\alpha_A}\left({{c_A} - {e_A}} \right)} \right]}^2}}}{{8{\alpha_A}}} = \pi_A^{FD*} \), \( F_B^{FC} \geq \frac{{{{\left[{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right)} \right]}^2}}}{{8{\alpha_B}}} = \pi_B^{FD*} \) and \( F_A^{FC} + F_B^{FC} \leq \frac{{3{{\left[{{D_A} - {\alpha_A}\left({{c_A} - {e_A}} \right)} \right]}^2}}}{{16{\alpha_A}}} + \frac{{3{{\left[{{D_B} - {\alpha_B}\left({{c_B} + {e_A}} \right)} \right]}^2}}}{{16{\alpha_B}}} = \frac{3}{2}\left({\pi_A^{FD*} + \pi_B^{FD*}} \right) \).
Similarly, we prove that when \( - {e_A} < {T^C} < {e_B} \) and \( {T^C} \geq {e_B} \), the feasibility of the two-part tariff coordination mechanism requires the same conditions.
Proof of Proposition 7
According to Table 2, if the carrier solely undertakes EER cost, when \( {T^C} \leq - {e_A} \), the EER volume is \( {E^{FD}}{ |_{{T^C} \leq - {e_A}}} = - \frac{{\left({{\alpha_A} + {\alpha_B}} \right)\left({{T^C} + {e_A}} \right)}}{4} \). Meanwhile, if the forwarder B undertakes all EER cost, when \( {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), the EER volume is \( {E^{FE}}{ |_{{T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} = - \frac{{\left({{\alpha_A} + {\alpha_B}} \right)\left({{T^C} + \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}} \right)}}{4} \). According to Proposition 1, when \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), the EER volume is \( {E^{FD}}{ |_{- {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} = 0 \). Through solving centralized marine service chain, when \( {T^C} \leq - {e_A} \) and \( {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), the EER volume is \( {E^{FC}}{ |_{{T^C} \leq - {e_A}}} = - \frac{{\left({{\alpha_A} + {\alpha_B}} \right)\left({{T^C} + {e_A}} \right)}}{2} \) and \( {E^{FC}}{ |_{- {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} = 0 \) respectively.
We first compare the EER volume under scenarios that carrier undertaking EER cost and coordination, apparently we always have \( {E^{FC}} > {E^{FD}} \) when \( {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \). Then we compare the EER volume under scenarios that carrier undertaking EER cost and EER cost sharing, when \( {T^C} \leq - {e_A} \), we obtain \( {E^{FE}}{ |_{{T^C} \leq - {e_A}}} - {E^{FD}}{ |_{{T^C} \leq - {e_A}}} = \frac{{{\alpha_A}{e_A}}}{4} > 0 \). When \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), straight forwardly we get \( {E^{FE}}{ |_{- {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} > {E^{FD}}{ |_{- {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} \). In summary, we get \( {E^{FE}} > {E^{FD}} \).
Lastly, we compare the EER volume under EER sharing and coordination. When \( {T^C} \leq - {e_A} \), we have \( {E^{FC}}{ |_{{T^C} \leq - {e_A}}} - {E^{FE}}{ |_{{T^C} \leq - {e_A}}} = - \frac{{\left({{\alpha_A} + {\alpha_B}} \right)\left({{T^C} + \frac{{2{\alpha_A} + {\alpha_B}}}{{{\alpha_A} + {\alpha_B}}}{e_A}} \right)}}{4} \). Due to \( {E^{FC}}{ |_{{T^C} \leq - {e_A}}} - {E^{FE}}{ |_{{T^C} \leq - {e_A}}} \) is strictly decreasing function of \( {T^C} \), we get \( {E^{FC}}{ |_{{T^C} \leq - {e_A}}} > {E^{FE}}{ |_{{T^C} \leq - {e_A}}} \) if \( {T^C} < - {e_A} - \frac{\alpha_A}{{{\alpha_A} + {\alpha_B}}}{e_A} \). When \( - {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A} \), straight forwardly we get \( {E^{FC}}{ |_{- {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} < {E^{FE}}{ |_{- {e_A} < {T^C} \leq - \frac{\alpha_B}{{{\alpha_A} + {\alpha_B}}}{e_A}}} \). In summary, when \( {T^C} < - {e_A} - \frac{\alpha_A}{{{\alpha_A} + {\alpha_B}}}{e_A} \), we get \( {E^{FC}} > {E^{FE}} \).
Rights and permissions
About this article
Cite this article
Zhang, X., Zhang, X. Pricing and coordination of marine service chain with empty equipment repositioning. Evol. Intel. 16, 1789–1799 (2023). https://doi.org/10.1007/s12065-020-00351-6
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12065-020-00351-6