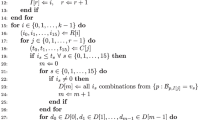Skip to main content

Advertisement

Springer Nature Link
Log in

Secondary constructions of Boolean functions with maximum algebraic immunity

  • Published:
Cryptography and Communications Aims and scope Submit manuscript

Abstract

The algebraic immunity of cryptographic Boolean functions with odd number of variables is studied in this paper. Proper modifications of functions with maximum algebraic immunity are proved that yield new functions whose algebraic immunity is also maximum. Several results are provided for both the multivariate and univariate representation, and their applicability is shown on known classes of Boolean functions. Moreover, new efficient algorithms to produce functions of guaranteed maximum algebraic immunity are developed, which further extend and generalize well-known constructions in this area. It is shown that high nonlinearity as well as good behavior against fast algebraic attacks are also achievable in several cases.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Armknecht, F., Carlet, C., Gaborit, P., Künzli, S., Meier, W., Ruatta, O.: Efficient computation of algebraic immunity for algebraic and fast algebraic attacks. In: Advances in Cryptology - Eurocrypt 2006. Lecture Notes in Computer Science, vol. 4004, pp. 147–164. Springer-Heidelberg (2006)

  2. Braeken, A., Preneel, B.: On the algebraic immunity of symmetric Boolean functions. In: Advances in Cryptology - Indocrypt 2005. Lecture Notes in Computer Science, vol. 3797, pp. 35–48. Springer-Heidelberg (2005)

  3. Canteaut, A.: Open problems related to algebraic attacks on stream ciphers. International Workshop on Coding and Cryptography (WCC), pp. 1–11 (2005)

  4. Carlet, C., Dalai, D.K., Gupta, K.C., Maitra, S.: Algebraic immunity for cryptographically significant Boolean functions: analysis and construction. IEEE Trans. Inf. Theory 52, 3105–3121 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  5. Carlet, C.: Constructing balanced functions with optimum algebraic immunity. In: Proc. IEEE Int. Symp. Inf. Theory (ISIT), pp. 451–455 (2007)

  6. Carlet, C., Feng, K.: An infinite class of balanced functions with optimal algebraic immunity, good immunity to fast algebraic attacks and good nonlinearity. In: Asiacrypt 2008. Lecture Notes in Computer Science, vol. 5350, pp. 425–440. Springer-Heidelberg (2008)

  7. Carlet, C., Gaborit, P.: On the construction of balanced Boolean functions with a good algebraic immunity. In: Proc. IEEE Int. Symp. Inf. Theory (ISIT), pp. 1101–1105 (2005)

  8. Carlet, C., Zeng, X., Li, C., Hu, L.: Further properties of several classes of Boolean functions with optimum algebraic immunity. Designs Codes Cryptogr. 52, 303–338 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  9. Carlet, C.: Comments on constructions of cryptographically significant Boolean functions using primitive polynomials. IEEE Trans. Inf. Theory 57, 4852–4853 (2011)

    Article  MathSciNet  Google Scholar 

  10. Chen, Y., Lu, P.: Two classes of symmetric Boolean functions with optimum algebraic immunity: construction and analysis. IEEE Trans. Inf. Theory 57, 2522–2538 (2011)

    Article  Google Scholar 

  11. Cormen, T.H., Leiserson, C.E., Rivest, R.L.: Introduction to Algorithms. MIT Press (1990)

  12. Courtois, N., Meier, W.: Algebraic attacks on stream ciphers with linear feedback. In: Advances in Cryptology - Eurocrypt 2003. Lecture Notes in Computer Science, vol. 2656, pp. 345–359. Springer-Heidelberg (2003)

  13. Courtois, N.: Fast algebraic attacks on stream ciphers with linear feedback. In: Advances in Cryptology - Crypto 2003. Lecture Notes in Computer Science, vol. 2729, pp. 176–194. Springer-Heidelberg (2003)

  14. Dalai, D.K., Maitra, S., Sarkar, S.: Basic theory in construction of Boolean functions with maximum possible annihilator immunity. Designs Codes Cryptogr. 40, 41–58 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  15. Du, Y., Zhang, F.G.: Finding more Boolean functions with maximum algebraic immunity based on univariate polynomial representation. In: ACISP 2011. Lecture Notes in Computer Science, vol. 6812, pp. 47–60. Springer-Heidelberg (2011)

  16. Du, Y., Zhang F.: On the existence of Boolean functions with optimal resistance against fast algebraic attacks. Cryptology ePrint Archive, Report 2012/210 (2012). Available http://eprint.iacr.org

  17. ECRYPT. D. STVL.9, Ongoing research areas in symmetric cryptography, ECRYPT Deliverable (2008)

  18. Li, N., Qi, W.: Construction and analysis of Boolean functions of 2t + 1 variables with maximum algebraic immunity. In: Advances in Cryptology - Asiacrypt 2006. Lecture Notes in Computer Science, vol. 4284, pp. 84–98. Springer-Heidelberg (2006)

  19. Li, N., Qu, L., Qi, W., Feng, G., Li, C., Xie, D.: On the construction of Boolean functions with optimal algebraic immunity. IEEE Trans. Inf. Theory 54, 1330–1334 (2008)

    Article  MathSciNet  Google Scholar 

  20. Liu, M., Zhang, Y., Lin, D.: Perfect algebraic immune functions. Cryptology ePrint Archive, Report 2012/212 (2012). Available http://eprint.iacr.org

  21. MacWilliams, F.J., Sloane, N.J.A.: The Theory of Error Correcting Codes. North-Holland, Amsterdam (1977)

    MATH  Google Scholar 

  22. Meier, W., Pasalic, E., Carlet, C.: Algebraic attacks and decomposition of Boolean functions. In: Advances in Cryptology - Eurocrypt 2004. Lecture Notes in Computer Science, vol. 3027, pp. 474–491. Springer-Heidelberg (2004)

  23. Pasalic, E.: Almost fully optimized infinitive classes of Boolean functions resistant to (fast) algebraic cryptanalysis. In: ICISC 2008. Lecture Notes in Computer Science, vol. 5461, pp. 399–414. Springer-Heidelberg (2008)

  24. Pasalic, E.: A design of Boolean functions resistant to (fast) algebraic cryptanalysis with efficient implementation. Cryptogr. Commun. 4, 25–45 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  25. Qu, L., Li, C., Feng, K.: A note on symmetric Boolean functions with maximum algebraic immunity in odd number of variables. IEEE Trans. Inf. Theory 53, 2908–2910 (2007)

    Article  MathSciNet  Google Scholar 

  26. Qu, L., Feng, K., Liu, F., Wang, L.: Constructing symmetric Boolean functions with maximum algebraic immunity. IEEE Trans. Inf. Theory 55, 2406–2412 (2009)

    Article  MathSciNet  Google Scholar 

  27. Rizomiliotis, P.: On the resistance of Boolean functions against algebraic attacks using univariate polynomial representation. IEEE Trans. Inf. Theory 56, 4014–4024 (2010)

    Article  MathSciNet  Google Scholar 

  28. Sarkar, S., Maitra, S.: Construction of rotation symmetric Boolean functions on odd number of variables with maximum algebraic immunity. In: AAECC 2007. Lecture Notes in Computer Science, vol. 4851, pp. 271–280. Springer-Heidelberg (2007)

  29. Siegenthaler, T.: Correlation-immunity of nonlinear combining functions for cryptographic applications. IEEE Trans. Inf. Theory 30, 776–780 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  30. Wang, C., Chen, X.: On extended algebraic immunity. Designs Codes Cryptogr. 57, 271–281 (2010)

    Article  MATH  Google Scholar 

  31. Wang, Q., Peng, J., Kan, H.: Constructions of cryptographically significant Boolean functions using primitive polynomials. IEEE Trans. Inf. Theory 56, 3048–3053 (2010)

    Article  MathSciNet  Google Scholar 

  32. Zeng, X., Carlet, C., Shan, J., Hu, L.: More balanced Boolean functions with optimal algebraic immunity and good nonlinearity and resistance to fast algebraic attacks. IEEE Trans. Inf. Theory 57, 6310–6320 (2011)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This research has been co-financed by the European Union (European Social Fund – ESF) and Greek national funds through the Operational Program “Education and Lifelong Learning” of the National Strategic Reference Framework (NSRF) - Research Funding Program THALIS: Secure wireless nonlinear communications at the physical layer.

Author information

Authors and Affiliations

  1. Hellenic Data Protection Authority, Kifissias 1–3, Athens, Greece

    Konstantinos Limniotis

  2. Department of Informatics and Telecommunications, National and Kapodistrian University of Athens, 15784, Athens, Greece

    Konstantinos Limniotis & Nicholas Kalouptsidis

  3. Department of Computer Science and Technology, University of Peloponnese, End of Karaiskaki Str., 22100, Tripolis, Greece

    Nicholas Kolokotronis

Authors
  1. Konstantinos Limniotis
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Nicholas Kolokotronis
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Nicholas Kalouptsidis
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Konstantinos Limniotis.

Additional information

Part of this work has been presented at the IEEE Int’l Symp. Inform. Theory (ISIT), St. Petersburg, Russia, July 31–August 6, 2011. A new section with constructions of functions based on their univariate representation has been added, whereas the results based on the multivariate representation have been extended. Moreover, the behavior of the constructions against fast algebraic attacks is also discussed in a new section.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Limniotis, K., Kolokotronis, N. & Kalouptsidis, N. Secondary constructions of Boolean functions with maximum algebraic immunity. Cryptogr. Commun. 5, 179–199 (2013). https://doi.org/10.1007/s12095-013-0081-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12095-013-0081-2

Keywords

Mathematics Subject Classifications (2000)