Skip to main content
SpringerLink
Log in

Updating reducts in fuzzy \(\beta \)-covering via matrix approaches while coarsening and refining a covering element

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

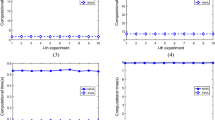
In a dynamic environment, knowledge reduction of information systems with variations of object sets, attribute sets and attribute values is an important topic, and related covering reduction of dynamic fuzzy \(\beta \)-covering approximation space when coarsening and refining a covering element has attracted little attention. How to update the reducts based on the original information is an important problem since it can help to improve the efficiency of knowledge discovery. This paper provides the definitions of the coarsening and refinement of a covering element in the dynamic fuzzy \(\beta \)-covering approximation space. Then approaches for updating the reducts by the relation character matrix are discussed when coarsening and refining a covering element. And the corresponding algorithms for computing new reducts are derived. Finally, several examples were given to illustrate the validity of the proposed approaches.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Zakowski, W.: Approximations in the space (u, \(\pi \)). Demonstr. Math. 16(3), 761 (1983)

    MATH  Google Scholar 

  2. Pawlak, Z.: Rough sets. Int. J. Comput. Inf. Sci. 11(5), 341 (1982)

    Article  MATH  Google Scholar 

  3. Wang, C., Chen, D., Hu, Q.: Fuzzy information systems and their homomorphisms. Fuzzy Sets Syst. 249, 128 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  4. Feng, T., Zhang, S.P., Mi, J.S.: The reduction and fusion of fuzzy covering systems based on the evidence theory. Int. J. Approx. Reason. 53(1), 87 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  5. Wu, M.F., Han, H.H., Si, Y.F., In: 2012 International Conference on Machine Learning and Cybernetics, vol. 1, pp. 184–189. IEEE (2012)

  6. Li, T.J., Leung, Y., Zhang, W.X.: Generalized fuzzy rough approximation operators based on fuzzy coverings. Int. J. Approx. Reason. 48(3), 836 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  7. Deng, T., Chen, Y., Xu, W., Dai, Q.: A novel approach to fuzzy rough sets based on a fuzzy covering. Inf. Sci. 177(11), 2308 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  8. Zhan, J., Zhang, X., Yao, Y.: Covering based multigranulation fuzzy rough sets and corresponding applications. Artif. Intell. Rev. 53(2), 1093 (2020)

    Article  Google Scholar 

  9. Zhang, K., Zhan, J., Wu, W.Z.: Novel fuzzy rough set models and corresponding applica- tions to multi-criteria decision-making. Fuzzy Sets Syst. 383, 92 (2020)

    Article  Google Scholar 

  10. Zhan, J., Sun, B., Zhang, X.: Pf-topsis method based on cpfrs models: an application to unconventional emergency events. Comput. Ind. Eng. 139, 106192 (2020)

    Article  Google Scholar 

  11. Zhang, L., Zhan, J., Yao, Y., et al.: Intuitionistic fuzzy TOPSIS method based on CVPIFRS models: an application to biomedical problems. Inf. Sci. 517, 315–339 (2020)

    Article  MathSciNet  Google Scholar 

  12. Ma, L.: Two fuzzy covering rough set models and their generalizations over fuzzy lattices. Fuzzy Sets Syst. 294, 1 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  13. Zhang, L., Zhan, J.: Fuzzy soft \(\beta \)-covering based fuzzy rough sets and corresponding decision-making applications. Int. J. Mach. Learn. Cybern. 10(6), 1487 (2019)

    Article  Google Scholar 

  14. Zhang, L., Zhan, J., Alcantud, J.C.R.: Novel classes of fuzzy soft \(\beta \)-coverings-based fuzzy rough sets with applications to multi-criteria fuzzy group decision making. Soft Comput. 23(14), 5327 (2019)

    Article  MATH  Google Scholar 

  15. Yang, B., Hu, B.Q.: A fuzzy covering-based rough set model and its generalization over fuzzy lattice. Inf. Sci. 367, 463 (2016)

    Article  MATH  Google Scholar 

  16. Yang, B., Hu, B.Q.: On some types of fuzzy covering-based rough sets. Fuzzy Sets Syst. 312, 36 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  17. D’eer, L., Restrepo, M., Cornelis, C., Gomez, J.: Neighborhood operators for covering-based rough sets. Inf. Sci. 336, 21 (2016)

    Article  MATH  Google Scholar 

  18. D’eer, L., Cornelis, C., Godo, L.: Fuzzy neighborhood operators based on fuzzy coverings. Fuzzy Sets Syst. 312, 17 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  19. Lang, G., Li, Q., Cai, M., Yang, T.: Characteristic matrixes-based knowledge reduction in dynamic covering decision information systems. Knowl.-Based Syst. 85, 1 (2015)

    Article  Google Scholar 

  20. Li, S., Li, T., Liu, D.: Dynamic maintenance of approximations in dominance-based rough set approach under the variation of the object set. Int. J. Intell. Syst. 28(8), 729 (2013)

    Article  MathSciNet  Google Scholar 

  21. Jing, Y., Li, T., Luo, C., Horng, S.J., Wang, G., Yu, Z.: An incremental approach for attribute reduction based on knowledge granularity. Knowl.-Based Syst. 104, 24 (2016)

    Article  Google Scholar 

  22. Hu, J., Li, T., Luo, C., Fujita, H., Li, S.: Incremental fuzzy probabilistic rough sets over two universes. Int. J. Approx. Reason. 81, 28 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  23. Hu, C., Liu, S., Liu, G.: Matrix-based approaches for dynamic updating approximations in multigranulation rough sets. Knowl.-Based Syst. 122, 51 (2017)

    Article  Google Scholar 

  24. Jing, Y., Li, T., Huang, J., Zhang, Y.: An incremental attribute reduction approach based on knowledge granularity under the attribute generalization. Int. J. Approx. Reason. 76, 80 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  25. Li, T., Ruan, D., Geert, W., Song, J., Xu, Y.: A rough sets based characteristic relation approach for dynamic attribute generalization in data mining. Knowl.-Based Syst. 20(5), 485 (2007)

    Article  Google Scholar 

  26. Huang, Y., Li, T., Luo, C., Fujita, H., Horng, S.J.: Dynamic variable precision rough set approach for probabilistic set-valued information systems. Knowl.-Based Syst. 122, 131 (2017)

    Article  Google Scholar 

  27. Li, S., Li, T.: Incremental update of approximations in dominance-based rough sets approach under the variation of attribute values. Inf. Sci. 294, 348 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  28. Luo, C., Li, T., Chen, H., Lu, L.: Fast algorithms for computing rough approximations in set-valued decision systems while updating criteria values. Inf. Sci. 299, 221 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  29. Zeng, A., Li, T., Hu, J., Chen, H., Luo, C.: Dynamical updating fuzzy rough approximations for hybrid data under the variation of attribute values. Inf. Sci. 378, 363 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  30. Cai, M., Li, Q., Ma, J.: Knowledge reduction of dynamic covering decision information systems caused by variations of attribute values. Int. J. Mach. Learn. Cybern. 8(4), 1131 (2017)

    Article  Google Scholar 

  31. Lang, G., Miao, D., Yang, T., Cai, M.: Knowledge reduction of dynamic covering decision information systems when varying covering cardinalities. Inf. Sci. 346, 236 (2016)

    Article  MATH  Google Scholar 

  32. Yang, Y., Chen, D., Wang, H., Tsang, E.C., Zhang, D.: Fuzzy rough set based incremental attribute reduction from dynamic data with sample arriving. Fuzzy Sets Syst. 312, 66 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  33. Qian, J., Dang, C., Yue, X., Zhang, N.: Attribute reduction for sequential three-way decisions under dynamic granulation. Int. J. Approx. Reason. 85, 196 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  34. Huang, J., Yu, P., Li, W.: Updating the reduct in fuzzy \(\beta \)-covering via matrix approaches while adding and deleting some objects of the universe. Information 11(1), 3 (2020)

    Article  Google Scholar 

Download references

Acknowledgements

This work is supported by National Natural Science Foundation of China (Nos. 11871259, 61379021, 11701258). Natural Science Foundation of Fujian Province, China (Nos. 2019J01748, 2017J01507).

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Minnan Normal University, Zhangzhou, 363000, Fujian, China

    Liting Xu, Jinjin Li & Peiqiu Yu

Authors
  1. Liting Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jinjin Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Peiqiu Yu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jinjin Li.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Xu, L., Li, J. & Yu, P. Updating reducts in fuzzy \(\beta \)-covering via matrix approaches while coarsening and refining a covering element. J. Appl. Math. Comput. 63, 717–737 (2020). https://doi.org/10.1007/s12190-020-01336-5

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-020-01336-5

Keywords

Mathematics Subject Classification

Search

Navigation