Skip to main content
SpringerLink
Log in

A qualitative study and numerical simulations for a time-delayed dispersive equation

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

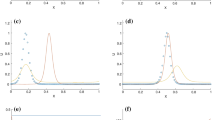
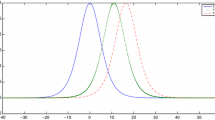
This paper deals with the stability analysis of a nonlinear time-delayed dispersive equation of order four. First, we prove the well-posedness of the system and give some regularity results. Then, we show that the zero solution of the system exponentially converges to zero when the time tends to infinity provided that the time-delay is small and the damping term satisfies reasonable conditions. Lastly, an intensive numerical study is put forward and numerical illustrations of the stability result are provided.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Ait Benhassi, E.M., Ammari, K., Boulite, S., Maniar, L.: Feedback stabilization of a class of evolution equations with delay. J. Evol. Equ. 9, 103–121 (2009)

    MathSciNet  MATH  Google Scholar 

  2. Al-Khaled, K., Haynes, N., Schiesser, W., Usman, M.: Eventual periodicity of the forced oscillations for a Korteweg–de Vries type equation on a bounded domain using a sinc collocation method. J. Comput. Appl. Math. 330, 417–428 (2018)

    MathSciNet  MATH  Google Scholar 

  3. Al-Musallam, F., Ammari, K., Chentouf, B.: Asymptotic analysis of a 2D overhead crane with input delays in the boundary control. Zeitschrift fur Angewandte Mathematik und Mechanik 98, 1103–1122 (2018)

    Google Scholar 

  4. Ammari, K., Chentouf, B.: Further results on the long-time behavior of a 2D overhead crane with a boundary delay: exponential convergence. Appl. Math. Comput. (2020). https://doi.org/10.1016/j.amc.2019.124698

    Article  MathSciNet  MATH  Google Scholar 

  5. Ammari, K., Chentouf, B.: On the exponential and polynomial convergence for a delayed wave equation without displacement. Appl. Math. Lett. 86, 126–133 (2018)

    MathSciNet  MATH  Google Scholar 

  6. Ammari, K., Crépeau, E.: Feedback stabilization and boundary controllability of the Korteweg–de Vries equation on a star-shaped network. SIAM J. Control Optim. 56, 1620–1639 (2018)

    MathSciNet  MATH  Google Scholar 

  7. Ammari, K., Crépeau, E.: Well-posedness and stabilization of the Benjamin–Bona–Mahony equation on star-shaped networks. Syst. Control Lett. 127, 39–43 (2019)

    MathSciNet  MATH  Google Scholar 

  8. Ammari, K., Nicaise, S.: Stabilization of Elastic Systems by Collocated Feedback. Lecture Notes in Mathematics, vol. 2124. Springer, Cham (2015)

    MATH  Google Scholar 

  9. Ammari, K., Nicaise, S., Pignotti, C.: Stability of an abstract-wave equation with delay and a Kelvin–Voigt damping. Asymptot. Anal. 95, 21–38 (2015)

    MathSciNet  MATH  Google Scholar 

  10. Ammari, K., Nicaise, S., Pignotti, C.: Stabilization by switching time-delay. Asymptot. Anal. 83, 263–283 (2013)

    MathSciNet  MATH  Google Scholar 

  11. Ammari, K., Nicaise, S., Pignotti, C.: Feedback boundary stabilization of wave equations with interior delay. Syst. Control Lett. 59, 623–628 (2010)

    MathSciNet  MATH  Google Scholar 

  12. Ascher, U.M., Petzold, L.R.: Computer Methods for Ordinary Differential Equations and Differential-Algebraic Equations. SIAM, Philadelphia (1998). ISBN 0-89871-412-5

    MATH  Google Scholar 

  13. Balogh, A., Gilliam, D.S., Shubov, V.I.: Stationary solutions for a boundary controlled Burgers’ equation. Math. Comput. Model. 33, 21–37 (2001)

    MathSciNet  MATH  Google Scholar 

  14. Balogh, A., Krstic, M.: Global boundary stabilization and regularization of Burgers’ equation. In: Proceedings of the American Control Conference, San Diego, California, pp. 1712–1716 (1999)

  15. Balogh, A., Krstic, M.: Burgers’ equation with nonlinear boundary feedback: stability, well-posedness and simulation. Math. Probl. Eng. 6, 189–200 (2000)

    MathSciNet  MATH  Google Scholar 

  16. Biler, P.: Asymptotic behavior in time of solutions to some equations generalizing the Korteweg–de Vries–Burgers equation. Bull. Pol. Acad. Sci. Math. 32, 275–282 (1984)

    MATH  Google Scholar 

  17. Biler, P.: Large-time behavior of periodic solutions to dissipative equations of Korteweg–de Vries–Burgers type. Bull. Pol. Acad. Sci. Math. 32, 401–405 (1984)

    MATH  Google Scholar 

  18. Bona, J.L., Dougalis, V.A., Karakashian, O.A., McKinney, W.R.: Computations of blow-up and decay for periodic solutions of the generalized Korteweg–de Vries Burgers equation. Appl. Numer. Math. 10, 335–355 (1992)

    MathSciNet  MATH  Google Scholar 

  19. Bona, J.L., Luo, L.: Decay of solutions to nonlinear, dispersive wave equations. Differ. Integr. Equ. 6, 961–980 (1993)

    MathSciNet  MATH  Google Scholar 

  20. Bona, J.L., Luo, L.: More results on the decay of solutions to nonlinear dispersive wave equations. Discrete Contin. Dyn. Syst. 1, 151–193 (1995)

    MathSciNet  MATH  Google Scholar 

  21. Bona, J.L., Dougalis, V.A., Karakashian, A., McKinney, W.R.: The effect of dissipation on solutions of the generalized Korteweg–de Vries equation. J. Comput. Appl. Math. 74, 127–154 (1996)

    MathSciNet  MATH  Google Scholar 

  22. Boussinesq, J.: Essai sur la théorie des eaux courantes, Mémoires Présentés par Divers Savants à l’Acad. des Sci. Inst. Nat. Fr. 23, 1–680 (1877)

    Google Scholar 

  23. Brezis, H.: Functional Analysis, Sobolev Spaces and Partial Differential Equations, Universitex. Springer, Berlin (2011)

    MATH  Google Scholar 

  24. Capistrano-Filho, R.A., Zhang, B.Y.: Initial boundary value problem for Korteweg–de Vries equation: a review and open problems. São Paulo J. Math. Sci. 13, 402–417 (2019)

    MathSciNet  MATH  Google Scholar 

  25. Cerpa, E.: Control of a Korteweg–de Vries equation: a tutorial. Math. Control Relat. Fields 4, 45–99 (2014)

    MathSciNet  MATH  Google Scholar 

  26. Cerpa, E., Crépeau, E.: Boundary controllability for the nonlinear Korteweg–de Vries equation on any critical domain. Annales de l’Institut Henri Poincare (C) Non Linear Analysis 26, 457–475 (2009)

    MathSciNet  MATH  Google Scholar 

  27. Chang, H.C.: Nonlinear waves on liquid film surfaces-II. Flooding in a vertical tube. Chem. Eng. Sci. 41, 2463–2476 (1986)

    Google Scholar 

  28. Chentouf, B.: Compensation of the interior delay effect for a rotating disk-beam system. IMA J. Math. Control Inf. 33(4), 963–978 (2016)

    MathSciNet  MATH  Google Scholar 

  29. Chentouf, B., Smaoui, N., Alalabi, A.: Nonlinear adaptive boundary control of the modified generalized Korteweg–de Vries–Burgers equation. Complexity 2020, Article ID 4574257, 1–18 (2020)

  30. Cohen, B.I., Krommes, J.A., Tang, W.M., Rosenbluth, M.N.: Nonlinear saturation of the dissipative trapped-ion mode by mode coupling. Nucl. Fusion 16, 971–992 (1976)

    Google Scholar 

  31. Coron, J.M., Crépeau, E.: Exact boundary controllability of a nonlinear KdV equation with critical lengths. J. Eur. Math. Soc. 6, 367–398 (2004)

    MathSciNet  MATH  Google Scholar 

  32. Crépeau, E.: Exact boundary controllability of the Korteweg–de Vries equation with a piecewise constant main coefficient. Syst. Control Lett. 97, 157–162 (2016)

    MathSciNet  MATH  Google Scholar 

  33. Erdoğan, M.B., Tzirakis, N.: Dispersive Partial Differential Equations. Cambridge University Press, Cambridge (2016)

    MATH  Google Scholar 

  34. Ferhat, M., Hakem, A.: Asymptotic behavior for a weak viscoelastic wave equations with a dynamic boundary and time varying delay term. J. Appl. Math. Comput. 51, 509–526 (2016)

    MathSciNet  MATH  Google Scholar 

  35. Hardy, G.H., Littlewood, J.E., Pólya, G.: Inequalities, 2nd edn. Cambridge University Press, Cambridge (1988)

    MATH  Google Scholar 

  36. Hussein, A.J., Kashkool, H.A.: Weak Galerkin finite element method for solving one-dimensional coupled Burgers’ equations. J. Appl. Math. Comput. 63, 265–293 (2020)

    MathSciNet  Google Scholar 

  37. Jeffrey, A., Kakutani, T.: Weak nonlinear dispersive waves: a discussion centered around the Korteweg–De Vries equation. SIAM Rev. 14, 582–643 (1972)

    MathSciNet  MATH  Google Scholar 

  38. Korteweg, D.J., de Vries, G.: On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves. Philos. Mag. 39, 422–443 (1895)

    MathSciNet  MATH  Google Scholar 

  39. Krstic, M.: On global stabilization of Burgers’ equation by boundary control. Syst. Control Lett. 37, 123–142 (1999)

    MathSciNet  MATH  Google Scholar 

  40. Kuramoto, Y., Tsuzuki, T.: On the formation of dissipative structures in reaction–diffusion systems. Progr. Theor. Phys. 54, 687–699 (1975)

    Google Scholar 

  41. Lighthill, M.J.: On waves generated in dispersive systems to travelling effects, with applications to the dynamics of rotating fluids. J. Fluid Mech. 27, 725–752 (1967)

    MATH  Google Scholar 

  42. Linares, F., Pazoto, A.F.: On the exponential decay of the critical generalized Korteweg–de Vries with localized damping. Proc. Am. Math. Soc. 135, 1515–1522 (2007)

    MathSciNet  MATH  Google Scholar 

  43. Linares, F., Ponce, G.: Introduction to Nonlinear Dispersive Equations. Springer-Verlag, New York (2009)

    MATH  Google Scholar 

  44. Liu, W.J., Krstic, M.: Adaptive control of Burgers’ equation with unknown viscosity. Int. J. Adapt. Control Signal Process. 15, 745–766 (2001)

    MATH  Google Scholar 

  45. Liu, W.J.: Asymptotic behavior of solutions of time-delayed Burgers equation. Discrete Contin. Dyn. Syst.-B 2, 47–56 (2002)

    MathSciNet  MATH  Google Scholar 

  46. Nicaise, S., Pignotti, C.: Stability and instability results of the wave equation with a delay term in the boundary or internal feedbacks. SIAM J. Control Optim. 45, 1561–1585 (2006)

    MathSciNet  MATH  Google Scholar 

  47. Pazoto, A.F.: Unique continuation and decay for the Korteweg–de Vries equation with localized damping. ESAIM: Control Optim. Calc. Var. 11, 473–486 (2005)

    MathSciNet  MATH  Google Scholar 

  48. Perla Menzala, G., Vasconcelos, C.F., Zuazua, E.: Stabilization of the Korteweg–de Vries equation with localized damping. Q. Appl. Math. 60, 111–129 (2002)

    MathSciNet  MATH  Google Scholar 

  49. Rosier, L.: Exact boundary controllability of the Korteweg–de Vries equation on a bounded domain. ESAIM: COCV 2, 33–55 (1997)

    MathSciNet  MATH  Google Scholar 

  50. Rosier, L., Zhang, B.Y.: Global stabilization of the generalized Korteweg–de Vries equation posed on a finite domain. SIAM J. Control Optim. 45, 927–956 (2006)

    MathSciNet  MATH  Google Scholar 

  51. Rosier, L., Zhang, B.Y.: Control and stabilization of the Korteweg–de Vries equation: recent progresses. J. Syst. Sci. Complex. 22, 647–682 (2009)

    MathSciNet  MATH  Google Scholar 

  52. Sivashinsky, G.: Nonlinear analysis for hydrodynamic instability in Laminar flames. Derivation of basic equations. Acta Astronaut. 4, 1177–1206 (1977)

    MathSciNet  MATH  Google Scholar 

  53. Smaoui, N.: Controlling the dynamics of Burgers equation with a high-order nonlinearity. Int. J. Math. Math. Sci. 62, 3321–3332 (2004)

    MathSciNet  MATH  Google Scholar 

  54. Smaoui, N.: Nonlinear boundary control of the generalized Burgers equation. Nonlinear Dyn. 37, 75–86 (2004)

    MathSciNet  MATH  Google Scholar 

  55. Smaoui, N., Al-Jamal, R.: A nonlinear boundary control for the dynamics of the generalized Korteweg–de Vries–Burgers equation. Kuwait J. Sci. Eng. 34, 57–76 (2007)

    MathSciNet  MATH  Google Scholar 

  56. Smaoui, N., Al-Jamal, R.: Boundary control of the generalized Korteweg–de Vries–Burgers equation. Nonlinear Dyn. 51, 439–446 (2008)

    MathSciNet  MATH  Google Scholar 

  57. Smaoui, N., El-Kadri, A., Zribi, M.: Adaptive boundary control of the forced generalized Korteweg–de Vries–Burgers equation. Eur. J. Control 16, 72–84 (2010)

    MathSciNet  MATH  Google Scholar 

  58. Smaoui, N., El-Kadri, A., Zribi, M.: Nonlinear boundary control of the unforced generalized Korteweg–de Vries–Burgers equation. Nonlinear Dyn. 60, 561–574 (2010)

    MathSciNet  MATH  Google Scholar 

  59. Smaoui, N., Chentouf, B., Alalabi, A.: Boundary linear stabilization of the modified generalized Korteweg–de Vries–Burgers equation. Adv. Differ. Equ. 2019, Article number: 457, 17 pages (2019)

  60. Smaoui, N., Mekkaoui, M.: The generalized Burgers equation with and without a time-delay. J. Appl. Math. Stoch. Anal. 1, 73–96 (2004)

    MathSciNet  MATH  Google Scholar 

  61. Smaoui, N., Zribi, M.: A finite dimensional control of the dynamics of the generalized Korteweg–de Vries Burgers equation. Appl. Math. Inf. Sci.-Int. J. 3, 207–221 (2009)

    MathSciNet  MATH  Google Scholar 

  62. Tang, Y., Wang, M.: A remark on exponential stability of time-delayed Burgers equation. Discrete Contin. Dyn. Syst. Ser. B. 12, 219–225 (2009)

    MathSciNet  MATH  Google Scholar 

  63. Tunç, C.: On the stability of solutions of certain fourth-order delay differential equations. Appl. Math. Mech. (Engl. Edn.) 27, 1141–1148 (2006)

    MathSciNet  MATH  Google Scholar 

  64. Tunç, C.: On the stability of solutions to a certain fourth-order delay differential equation. Nonlinear Dyn. 51, 71–81 (2008)

    MathSciNet  MATH  Google Scholar 

  65. Whiham, G.B.: Non-linear dispersive waves. Proc. R. Soc. Ser. A 283, 238–261 (1965)

    MathSciNet  Google Scholar 

  66. Whitham, G.B.: Linear and Nonlinear Waves, Pure and Applied Mathematics. Wiley, New York (1974)

    MATH  Google Scholar 

  67. Zabusky, N.J., Kruskal, M.D.: Interaction of “solitons” in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 15, 240–243 (1965)

    MATH  Google Scholar 

Download references

Acknowledgements

This work was supported and funded by Kuwait University, Research Grant No. SM05/18. The valuable corrections, suggestions, and comments from the editor and the anonymous referees are greatly appreciated.

Author information

Authors and Affiliations

  1. UR Analysis and Control of PDEs, UR 13ES64, Department of Mathematics, Faculty of Sciences of Monastir, University of Monastir, Monastir, Tunisia

    Kaïs Ammari

  2. Department of Mathematics, Faculty of Science, Kuwait University, 13060, Safat, Kuwait

    Boumediène Chentouf & Nejib Smaoui

Authors
  1. Kaïs Ammari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Boumediène Chentouf
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Nejib Smaoui
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Boumediène Chentouf.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ammari, K., Chentouf, B. & Smaoui, N. A qualitative study and numerical simulations for a time-delayed dispersive equation. J. Appl. Math. Comput. 66, 465–491 (2021). https://doi.org/10.1007/s12190-020-01446-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-020-01446-0

Keywords

Mathematics Subject Classification

Search

Navigation