Skip to main content
Springer Nature Link
Log in

Stochastic predator–prey Lévy jump model with Crowley–Martin functional response and stage structure

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

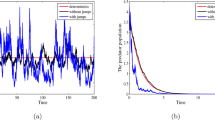
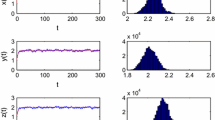
This paper investigates the dynamics of the stochastic predator–prey model with a Crowley–Martin functional response function driven by Lévy noise. First, the existence, uniqueness and boundedness of a global positive solution is proven. Next, the persfor an optimal conditions. Finally, the theoretical results are reinforced by some numerical simulations. We illustrated the sufficient conditions to classify the dynamics of the stochastic predator–prey model.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Tripathi, J.P., Tyagi, S., Abbas, S.: Global analysis of a delayed density dependent predator–prey model with Crowley–Martin functional response. Commun. Nonlinear Sci. Numer. Simul. 30, 45–69 (2016)

    Article  MathSciNet  Google Scholar 

  2. Meng, X.Y., Huo, H.F., Xiang, H., Yin, Q.Y.: Stability in a predator–prey model with Crowley–Martin function and stage structure for prey. Appl. Math. Comput. 232, 810–819 (2014)

    MathSciNet  MATH  Google Scholar 

  3. Liu, Q., Jiang, D.Q., Hayat, T., Alsaedi, A.: Dynamics of a stochastic predator-prey model with stage structure for predator and Holling type II functional response. J. Nonlinear Sci. 28, 1151–1187 (2018)

    Article  MathSciNet  Google Scholar 

  4. Lotka, A.: Elements of Mathematical Biology. Dover Publications, New York (1956)

    MATH  Google Scholar 

  5. Fay, T.H., Greeff, J.C.: Lion, wildebeest and zebra: a predator–prey model. Ecol. Modell. 196(1–2), 237–244 (2006)

    Article  Google Scholar 

  6. Spencer, P.D., Collie, J.S.: A simple predator–prey model of exploited marine fish populations incorporating alternative prey. ICES J. Mar. Sci. 53(3), 615–628 (1996)

    Article  Google Scholar 

  7. Mukherjee, D.: Persistence and bifurcation analysis on a predator–prey system of Holling type. Esaim Math. Model. Numer. Anal. 37, 339–344 (2003)

    Article  MathSciNet  Google Scholar 

  8. Liu, Z.J., Zhong, S.M.: Permanence and extinction analysis for a delayed periodic predator-prey system with Holling type II response function and diffusion. Appl. Math. Comput. 216, 3002–3015 (2010)

    MathSciNet  MATH  Google Scholar 

  9. Zhang, Z., Yang, H., Fu, M.: Hopf bifurcation in a predator-prey system with Holling type III functional response and time delays. J. Appl. Math. Comput. 44, 337–356 (2014)

    Article  MathSciNet  Google Scholar 

  10. Upadhyay, R.K., Naji, R.K.: Dynamics of a three species food chain model with Crowley–Martin type functional response. Chaos Solitons Fractals 42, 1337–1346 (2009)

    Article  MathSciNet  Google Scholar 

  11. Cui, J., Takeuchi, Y.: Permanence, extinction and periodic solution of predator-prey system with Beddington–DeAngelis functional response. J. Math. Anal. Appl. 317, 464–474 (2006)

    Article  MathSciNet  Google Scholar 

  12. Tripathi, J.P., Abbas, S., Thakur, M.: Dynamical analysis of a prey-predator model with Beddington–DeAngelis type function response incorporating a prey refuge. Nonlinear Dyn. 80, 177–196 (2015)

    Article  MathSciNet  Google Scholar 

  13. Wang, K., Zhu, Y.L.: Permanence and global asymptotic stability of a delayed predator-prey model with Hassell-Varley type functional response. Bull. Iran. Math. Soc. 94, 1–14 (2013)

    Google Scholar 

  14. Wang, W., Chen, L.: A predator–prey system with stage-structure for predator. Comput. Math. Appl. 33, 83–91 (1997)

    Article  MathSciNet  Google Scholar 

  15. Chen, Y., Song, C.: Stability and Hopf bifurcation analysis in a prey–predator system with stage-structure for prey and time delay. Chaos Solitons Fractals 38, 1104–1114 (2008)

    Article  MathSciNet  Google Scholar 

  16. Yang, W., Li, X., Bai, Z.: Permanence of periodic Holling type-IV predator–prey system with stage structure for prey. Math. Comput. Model. 48, 677–684 (2008)

    Article  MathSciNet  Google Scholar 

  17. Huang, C.Y., Zhao, M., Zhao, L.C.: Permanence of periodic predator–prey system with two predators and stage structure for prey. Nonlinear Anal. Real World Appl. 11(1), 503–514 (2010)

    Article  MathSciNet  Google Scholar 

  18. Meng, X.Y., Huo, H.F., Xiang, H., Yin, Q.Y.: Stability in a predator–prey model with Crowley–Martin function and stage structure for prey. Appl. Math. Comput. 232, 810–819 (2014)

    MathSciNet  MATH  Google Scholar 

  19. Sun, X.K., Huo, H.F., Xiang, H.: Bifurcation and stability analysis in predator–prey model with a stage-structure for predator. Nonlinear Dyn. 58, 497–513 (2009)

    Article  MathSciNet  Google Scholar 

  20. Xu, R.: Global dynamics of a predator–prey model with time delay and stage structure for the prey. Nonlinear Anal. Real World Appl. 12, 2151–2162 (2011)

    Article  MathSciNet  Google Scholar 

  21. Xu, C., Ren, G., Yu, Y.: Extinction analysis of stochastic predator–prey system with stage structure and Crowley–Martin functional response. Entropy 21(3), 252 (2019)

    Article  MathSciNet  Google Scholar 

  22. Liu, Q., Jiang, D., Hayat, T., Alsaedi, A.: Dynamics of a stochastic predator–prey model with stage structure for predator and Holling type II functional response. J. Nonlinear Sci. 28(3), 1151–1187 (2018)

    Article  MathSciNet  Google Scholar 

  23. Choo, S., Kim, Y.H.: Global stability in stochastic difference equations for predator–prey models. J. Comput. Anal. Appl. 23(1) (2017)

  24. Yang, L., Zhong, S.: Global stability of a stage-structured predator-prey model with stochastic perturbation. Discrete Dyn. Nat. Soc. (2014)

  25. Zhao, S., Song, M.: A stochastic predator–prey system with stage structure for predator. Abstr. Appl. Anal. (2014)

  26. Zhang, X., Li, W., Liu, M., Wang, K.: Dynamics of a stochastic Holling II one-predator two-prey system with jumps. Phys. A Stat. Mech. Appl. 421, 571–582 (2015)

    Article  MathSciNet  Google Scholar 

  27. Liu, Q., Chen, Q.M.: Analysis of a stochastic delay predator–prey system with jumps in a polluted environment. Appl. Math. Comput. 242, 90–100 (2014)

    MathSciNet  MATH  Google Scholar 

  28. Liu, M., Bai, C., Deng, M., Du, B.: Analysis of stochastic two-prey one-predator model with Lévy jumps. Phys. A Stat. Mech. Appl. 445, 176–188 (2016)

    Article  Google Scholar 

  29. Zhao, Y., Yuan, S.: Stability in distribution of a stochastic hybrid competitive Lotka–Volterra model with Lévy jumps. Chaos Solitons Fractals 85, 98–109 (2016)

    Article  MathSciNet  Google Scholar 

  30. Wu, J.: Stability of a three-species stochastic delay predator–prey system with Lévy noise. Phys. A Stat. Mech. Appl. 502, 492–505 (2018)

    Article  Google Scholar 

  31. Zou, X., Wang, K.: Numerical simulations and modeling for stochastic biological systems with jumps. Commun. Nonlinear Sci. Numer. Simulat 19, 1557–1568 (2014)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Laboratory of Mathematics and Applications, Faculty of Sciences and Techniques Mohammedia, University Hassan II-Casablanca, PO Box 146, Mohammedia, Morocco

    Jaouad Danane

  2. Department of Mathematics and Informatics, National School of Applied Sciences of Berrechid, University Hassan First of Settat, Settat, Morocco

    Jaouad Danane

Authors
  1. Jaouad Danane
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jaouad Danane.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Danane, J. Stochastic predator–prey Lévy jump model with Crowley–Martin functional response and stage structure. J. Appl. Math. Comput. 67, 41–67 (2021). https://doi.org/10.1007/s12190-020-01490-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-020-01490-w

Keywords