Skip to main content
Springer Nature Link
Log in

A minimal search method for solving fractional integro-differential equations based on modified Legendre multiwavelets

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

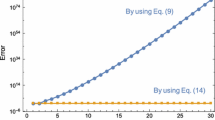
This paper improves the modified multiwavelets bases of minimal search method for the fractional integro-differential equation. First, it shows the unique solvability of the equation. And then the Legendre multiwavelets are improved and the modified multiwavelets in reproducing kernel space are obtained. Subsequently, it is established a strict theory for obtaining the \(\varepsilon \)-approximate solution with minimal search method. Finally, some examples show that the modified continuous multiwavelets method is more effective and stable than the Legendre multiwavelets and other methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Li, X., Wu, B.: A new reproducing kernel method for variable order fractional boundary value problems for functional differential equations. J. Comput. Appl. Math. 311, 387–393 (2017)

    Article  MathSciNet  Google Scholar 

  2. Geng, F., Cui, M.: A reproducing kernel method for solving nonlocal fractional boundary value problems. Appl. Math. Let. 25(5), 818–823 (2012)

    Article  MathSciNet  Google Scholar 

  3. Li, X., Wu, B.: Reproducing kernel functions-based meshless method for variable order fractional advection-diffusion-reaction equations. Alex. Eng. J. 59(5), 3181–3186 (2020)

    Article  Google Scholar 

  4. Mary, S., Tamilselvan, A.: Numerical method for a non-local boundary value problem with Caputo fractional order. J. Appl. Math. Comput. (2021). https://doi.org/10.1007/s12190-021-01501-4

    Article  MathSciNet  Google Scholar 

  5. Diethelm, K.: The Analysis of fractional differential equations. Lecture Notes in Mathematics (2004)

  6. Baillie, R.T.: Long memory processes and fractional integration in econometrics. J. Econometrics 73, 5–59 (1996)

    Article  MathSciNet  Google Scholar 

  7. Rossikhin, Y.A., Shitikova, M.V.: Applications of fractional calculus to dynamic problems of linear and nonlinear hereditary mechanics of solids. ASME Appl. Mech. Rev. 50, 15–67 (1997)

    Article  Google Scholar 

  8. Kilbas, A.A. Srivastava, H.M. and Trujillo, J.J. (2006) Theory and Applications of Fractional Differential Equations, in: North-Holland Mathematics Studies, vol. 204, Elsevier, Amsterdam

  9. Tarasov, V.E.: Fractional integro-differential equations for electromagnetic waves in dielectric media. Theoret. Math. Phys. 158, 355–359 (2009)

    Article  MathSciNet  Google Scholar 

  10. Du, H., Chen, Z., Yang, T.: A stable least residue method in reproducing kernel space for solving a nonlinear fractional integro-differential equation with a weakly singular kernel. Appl. Numer. Math. 157, 210–222 (2020)

    Article  MathSciNet  Google Scholar 

  11. Saadatmandi, A., Dehghan, M.: A Legendre collocation method for fractional integro-differential equations. J. Vib. Control 17, 2050–2058 (2011)

    Article  MathSciNet  Google Scholar 

  12. Rawashdeh, R.A.: Numerical solution of fractional integro-differential equations by collocation method. Appl. Math. Comput. 176, 1–6 (2006)

    MathSciNet  MATH  Google Scholar 

  13. Ma, X.H., Huang, C.M.: Spectral collocation method for linear fractional integro-differential equations. Appl. Math. Model. 38, 1434–1448 (2014)

    Article  MathSciNet  Google Scholar 

  14. Jiang, W., Li, H.: A space-time spectral collocation method for the two-dimensional variable-order fractional percolation equations. Comput. Math. Appl. 75, 3508–3520 (2018)

    Article  MathSciNet  Google Scholar 

  15. Zhang, X.G., Du, H.: A generalized collocation method in reproducing kernel space for solving a weakly singular Fredholm integro-differential equations. Appl. Numer. Math. 156, 158–173 (2020)

    Article  MathSciNet  Google Scholar 

  16. Xu, M.Q., Niu, J., Lin, Y.Z.: An efficient method for fractional nonlinear differential equations by quasi-Newton’s method and simplified reproducing kernel method. Math. Meth. Appl. Sci. 41(1), 5–14 (2018)

    Article  MathSciNet  Google Scholar 

  17. Omar, A.A., Banan, M.: Fitted fractional reproducing kernel algorithm for the numerical solutions of ABC-Fractional Volterra integro-differential equations. Chaos Soli. Fract. 126, 394–402 (2019)

    Article  MathSciNet  Google Scholar 

  18. Du, H., Chen, Z.: A new reproducing kernel method with higher convergence order for solving a Volterra-Fredholm integral equation. Appl. Math. Lett. 102, 106117 (2020)

    Article  MathSciNet  Google Scholar 

  19. Niu, J., Xu, M., Yao, G.: An efficient reproducing kernel method for solving the Allen-Cahn equation. Appl. Math. Lett. 89, 78–84 (2019)

    Article  MathSciNet  Google Scholar 

  20. Niu, J., Sun, L., Xu, M., Hou, J.: A reproducing kernel method for solving heat conduction equations with delay. Appl. Math. Lett. 100, 106036 (2020)

    Article  MathSciNet  Google Scholar 

  21. Xu, M., Zhang, L., Tohidi, E.: A fourth-order least-squares based reproducing kernel method for one-dimensional elliptic interface problems. Appl. Numer. Math. 162, 124–136 (2021)

    Article  MathSciNet  Google Scholar 

  22. Meyer, Y., Coifman, R.: Wavelets: Calderon-Zygmund and Multilinear Operator. Cambridge University Press, Cambridge (1997)

    MATH  Google Scholar 

  23. Cohen, A.: Numerical Analysis of Wavelet Methods. Elsevier, Amsterdam (2003)

    MATH  Google Scholar 

  24. Pan, G.W.: Wavelets in Electromagnetics and Device Modeling. Wiley-Interscience, London (2003)

    Book  Google Scholar 

  25. Yang, S.Z., Cheng, Z.X., Wang, H.Y.: Construction of biorthogonal multiwavelets. J. Math. Anal. Appl. 276, 1–12 (2002)

    Article  MathSciNet  Google Scholar 

  26. Dahmen, W., Kunoth, A., Urban, K.: Biorthogonal spline wavelets on the interval stability and moment conditions. Appl. Comput. Harmon. Anal. 6, 132–196 (1999)

    Article  MathSciNet  Google Scholar 

  27. Safar, I.P., Mehdi, D., Somayeh, A.M., Mehrdad, L.: Numerical solution for a class of fractional convectionCdiffusion equations using the flatlet oblique multiwavelets. J. Vib. Control 20, 913–924 (2014)

    Article  MathSciNet  Google Scholar 

  28. Rick, A., George, F., William, S.: Adaptive discontinuous Galerkin methods in multiwavelets bases. Appl. Numer. Math. 61, 879–890 (2011)

    Article  MathSciNet  Google Scholar 

  29. Chen, Z., Wu, L., Lin, Y.: Exact solution of a class of fractional integro-differential equations with the weakly singular kernel based on a new fractional reproducing kernel space. Math. Meth. Appl. Sci. 41, 3841–3855 (2018)

    Article  MathSciNet  Google Scholar 

  30. Brezis, H.: Functional analysis. Sobolev Spaces and Partial Differential Equations. Springer, New York (2010)

    Google Scholar 

  31. Alpert, B., Beylkin, G., Gines, D., Vozovoi, L.: Adaptive solution of partial differential equations in multiwavelet bases. J. Comput. Phys. 182, 149–190 (2002)

    Article  MathSciNet  Google Scholar 

  32. Lakestani, M., Saray, B.N., Dehghan, M.: Numerical solution for the weakly singular Fredholm integro-differential equations using Legendre multiwavelets. J. Comput. Appl. Math. 235, 3291–3303 (2011)

    Article  MathSciNet  Google Scholar 

  33. Cui, M.G., Wu, B.Y.: Numerical Analysis in Reproducing Kernel Space. Scientific Press, Peking (2004)

    Google Scholar 

  34. Yan, Q.J. :Numerical Analysis, 2nd ed., Beihang University Press, 2001

  35. Wu, L.B., Cheng, Z., Ding, X.H.: A stable minimal search method for solving multi-order fractional differential equations based on reproducing kernel space. Numer. Algor. (2020). https://doi.org/10.1007/s11075-020-01026-0

    Article  Google Scholar 

  36. Kumar, K., Pandey, R.K., Sharma, S.: Comparative study of three numerical schemes for fractional integro-differential equations. J. Comput. Appl. 315, 287–302 (2017)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work is supported by the Natural Science Foundation of Shandong Province of China (No. ZR2020MA050). The authors would like to express their appreciation to the anonymous referees for their many valuable suggestions and for carefully correcting the preliminary version of the manuscript.

Author information

Authors and Affiliations

  1. Department of Mathematics, Harbin Institute of Technology at Weihai, Weihai, Shandong, People’s Republic of China

    Longbin Wu, Zhong Chen & Xiaohua Ding

Authors
  1. Longbin Wu
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Zhong Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Xiaohua Ding
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Xiaohua Ding.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wu, L., Chen, Z. & Ding, X. A minimal search method for solving fractional integro-differential equations based on modified Legendre multiwavelets. J. Appl. Math. Comput. 68, 1467–1483 (2022). https://doi.org/10.1007/s12190-021-01573-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-021-01573-2

Keywords

Mathematics Subject Classification