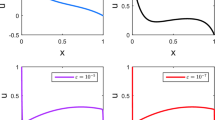
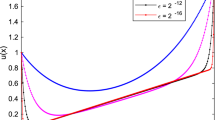
Abstract
In this paper, a linear singularly perturbed Fredholm integro-differential initial value problem with integral condition is being considered. On a Shishkin-type mesh, a fitted finite difference approach is applied using a composite trapezoidal rule in both; in the integral part of equation and in the initial condition. The proposed technique acquires a uniform second-order convergence in respect to perturbation parameter. Further provided the numerical results to support the theoretical estimates.


Similar content being viewed by others
References
Abdulghani, M., Hamoud, A., Ghandle, K.: The effective modification of some analytical techniques for Fredholm integro-differential equations. Bulletin of the International Mathematical Virtual Institute 9, 345–353 (2019)
Abdullah, J.T.: Numerical solution for linear Fredholm integro-differential equation using Touchard polynomials. Baghdad Sci. J. 18(2), 330–337 (2021)
Amiraliyev, G.M., Durmaz, M.E., Kudu, M.: Uniform convergence results for singularly perturbed Fredholm integro-differential equation. J. Math. Anal. 9(6), 55–64 (2018)
Amiraliyev, G.M., Durmaz, M.E., Kudu, M.: Fitted second order numerical method for a singularly perturbed Fredholm integro-differential equation Bull. Belg. Math. Soc. - Simon Stevin 27(1), 71–88 (2020)
Amiraliyev, G.M., Durmaz, M.E., Kudu, M.: A numerical method for a second order singularly perturbed Fredholm integro-differential equation. Miskolc Math. Notes 22(1), 37–48 (2021)
Amiraliyev, G.M., Mamedov, Y.D.: Difference schemes on the uniform mesh for singularly perturbed pseudo-parabolic equations. Turkish J. Math. 19, 207–222 (1995)
Arqub, O.A., Al-Smadi, M., Shawagfeh, N.: Solving Fredholm integro-differential equations using reproducing kernel Hilbert space method. Appl. Math. Comput. 219(17), 8938–8948 (2013)
Brunner, H.: Contemporary Computational Mathematics-A Celebration of the 80th Birthday of Ian Sloan. In: Dick, J., et al. (eds.) Numerical Analysis and Computational Solution of Integro-Differential Equations, pp. 205–231. Springer, Cham (2018)
Cakir, M.: A numerical study on the difference solution of singularly perturbed semilinear problem with integral boundary condition. Math. Model. Anal. 21(5), 644–658 (2016)
Cakir, M., Arslan, D.: A new numerical approach for a singularly perturbed problem with two integral boundary conditions. Comput. Appl. Math. 40, 189 (2021)
Cannon, J.R.: The solution of the heat equation subject to the specification of energy. Q. Appl. Math. 21(2), 155–160 (1963)
Chen, J., He, M., Huang, Y.: A fast multiscale Galerkin method for solving second order linear Fredholm integro-differential equation with Dirichlet boundary conditions. J. Comput. Appl. Math. 364, 112352 (2020)
Cimen, E., Cakir, M.: A uniform numerical method for solving singularly perturbed Fredholm integro-differential problem. Comput. Appl. Math. 40, 42 (2021)
Dag, H.G., Bicer, K.E.: Boole collocation method based on residual correction for solving linear Fredholm integro-differential equation. Journal of Science and Arts 3(52), 597–610 (2020)
Durmaz, M.E., Amiraliyev, G.M.: A robust numerical method for a singularly perturbed Fredholm integro-differential equation. Mediterr. J. Math. 18, 1–17 (2021)
Durmaz, M.E., Amiraliyev, G.M., Kudu, M.: Numerical solution of a singularly perturbed Fredholm integro differential equation with Robin boundary condition. Turk. J. Math. 46(1), 207–224 (2022)
Dzhumabaev, D.S., Nazarova, K.Z., Uteshova, R.E.: A modification of the parameterization method for a linear boundary value problem for a Fredholm integro-differential equation. Lobachevskii J. Math. 41, 1791–1800 (2020)
Farrell, P.A., Hegarty, A.F., Miller, J.J.H., O’Riordan, E., Shishkin, G.I.: Robust Computational Techniques for Boundary Layers. Chapman Hall/CRC, New York (2000)
Hamoud, A.A., Ghadle, K.P.: Usage of the variational iteration technique for solving Fredholm integro-differential equations. J. Comput. Appl. Mech. 50(2), 303–307 (2019)
Hosseini, S.M., Shahmorad, S.: Tau numerical solution of Fredholm integro-differential equations with arbitrary polynomial bases. Appl. Math. Model. 27(2), 145–154 (2003)
Ionkin, N.I.: Solution of a boundary value problem in heat conduction theory with nonlocal boundary conditions. Differ. Equ. 13, 294–304 (1977)
Kadalbajoo, M.K., Gupta, V.: A brief survey on numerical methods for solving singularly perturbed problems. Appl. Math. Comput. 217, 3641–3716 (2010)
Keimer, A., Pflug, L.: Modeling infectious diseases using integro-differential equations: Optimal control strategies for policy decisions and Applications in COVID-19, (2020), https://doi.org/10.13140/RG.2.2.10845.44000
Kudu, M., Amirali, I., Amiraliyev, G.M.: A finite-difference method for a singularly perturbed delay integro-differential equation. J. Comput. Appl. Math. 308, 379–390 (2016)
Kudu, M.: A parameter uniform difference scheme for the parameterized singularly perturbed problem with integral boundary condition, Adv. Differ. Equ., 170 (2018)
Miller, J.J.H., O’Riordan, E., Shishkin, G.I.: Fitted Numerical Methods for Singular Perturbation Problems: Error Estimates in the Maximum Norm for Linear Problems in One and Two Dimensions, Rev World Scientific, Singapore (2012)
Nayfeh, A.H.: Introduction to Perturbation Techniques. Wiley, New York (1993)
Nicoud, F., Schönfeld, T.: Integral boundary conditions for unsteady biomedical CFD applications. Int. J. Numer. Methods Fluids 40, 457–465 (2002)
O’Malley, R.E.: Singular Perturbations Methods for Ordinary Differential Equations. Springer, New York (2013)
Panda, A., Mohapatra, J., Amirali, I.: A second-order post-processing technique for singularly perturbed Volterra integro-differential equations. Mediterr. J. Math. 18, 231 (2021)
Panda, A., Mohapatra, J., Reddy, N.R.: A comparative study on the numerical solution for singularly perturbed Volterra integro-differential equations. Comput. Math. Model. 32, 364–375 (2021)
Pour-Mahmoud, J., Rahimi-Ardabili, M.Y., Shahmorad, S.: Numerical solution of the system of Fredholm integro-differential equations by the Tau method. Appl. Math. Comput. 168(1), 465–478 (2005)
Roos, H.G., Stynes, M., Tobiska, L.: Robust Numerical Methods for Singularly Perturbed Differential Equations. Springer-Verlag, Berlin Heidelberg (2008)
Samarskii, A.A.: The Theory of Difference Schemes. Marcell Dekker, Inc., New York (2001)
Shakti, D., Mohapatra, J.: A second order numerical method for a class of parameterized singular perturbation problems on adaptive grid. Nonlinear Eng. 6(3), 221–228 (2017)
Shakti, D., Mohapatra, J.: A uniformly convergent numerical scheme for singularly perturbed differential equation with integral boundary condition arising in neural network. Int. J. Computing Science and Mathematics 10(4), 340–350 (2019)
Tair, B., Guebbai, H., Segni, S., Ghiat, M.: An approximation solution of linear Fredholm integro-differential equation using Collocation and Kantorovich methods. J. Appl. Math, Comput (2021)
Tair, B., Guebbai, H., Segni, S., Ghiat, M.: Solving linear Fredholm integro-differential equation by Nyström method. J. Appl. Math. Comput. Mech. 20(3), 53–64 (2021)
Thieme, H.R.: A model for the spatial spread of an epidemic. J. Math. Biol. 4, 337–351 (1977)
Turkyilmazoglu, M.: Analytic approximate solutions of parameterized unperturbed and singularly perturbed boundary value problems. Appl. Math. Model. 35(8), 3879–3886 (2011)
Turkyilmazoglu, M.: An effective approach for numerical solutions of high-order Fredholm integro-differential equations. Appl. Math. Comput. 227, 384–398 (2014)
Turkyilmazoglu, M.: High-order nonlinear Volterra - Fredholm - Hammerstein integro-differential equations and their effective computation. Appl. Math. Comput. 247, 410–416 (2014)
Turkyilmazoglu, M.: Effective computation of exact and analytic approximate solutions to singular nonlinear equations of Lane-Emden-Fowler type. Appl. Math. Model. 37, 7539–7548 (2013)
Yalcinbas, S., Sezer, M., Sorkun, H.H.: Legendre polynomial solutions of high-order linear Fredholm integro-differential equations. Appl. Math. Comput. 210(2), 334–349 (2009)
Yapman, Ö., Amiraliyev, G.M.: Convergence analysis of the homogeneous second order difference method for a singularly perturbed Volterra delay-integro-differential equation. Chaos Solit. Fractals 150, 111100 (2021)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Durmaz, M.E., Amirali, I. & Amiraliyev, G.M. An efficient numerical method for a singularly perturbed Fredholm integro-differential equation with integral boundary condition. J. Appl. Math. Comput. 69, 505–528 (2023). https://doi.org/10.1007/s12190-022-01757-4
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12190-022-01757-4