Skip to main content

Advertisement

Springer Nature Link
Log in

Uniformly convergent finite difference methods for singularly perturbed parabolic partial differential equations with mixed shifts

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

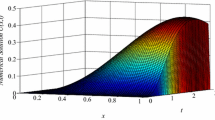
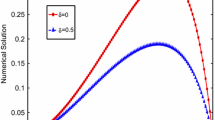
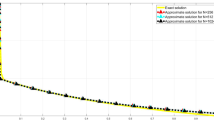
In this paper, exponentially fitted finite difference methods are presented for singularly perturbed one-dimensional parabolic partial differential equation with mixed shifts in the spatial variable. Such boundary value problems are ubiquitous in control theory, namely, the processing of metal sheets and the first exist time problems in modelling of activation of neuronal variability. The methods presented here are based upon the size of the shift parameter. When the shift parameters are smaller to the perturbation parameter, the shift terms are approximated by their Taylor series expansions, while for the shift parameters larger to the perturbation parameter, a special type of mesh is constructed so that the shifts lie on the nodal points after discretization. The proposed methods are checked for convergence. Also two test problems are solved with the proposed methods to demonstrate the applicability. Graphs are plotted to illustrate the effect of the shifts on the solution of the problems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Wu, J.: Theory and Applications of Partial Functional Differential Equations. Springer, NewYork (1996)

    Book  MATH  Google Scholar 

  2. Tuckwell, H.C.: Introduction to Theoretical Neurobiology, vol. 1. Cambridge University Press, Cambridge (1988)

    Book  MATH  Google Scholar 

  3. Tuckwell, H.C.: Introduction to Theoretical Neurobiology, vol. 2. Cambridge University Press, Cambridge (1988)

    Book  MATH  Google Scholar 

  4. Kadalbajoo, M.K., Patidar, K.C.: Singularly perturbed problems in partial differential equations: a survey. Appl. Math. Comput. 134, 371–429 (2003)

    MathSciNet  MATH  Google Scholar 

  5. Kumar, D., Kadalbajoo, M.K.: A parameter-uniform numerical method for time-dependent singularly perturbed differential difference equations. Appl. Math. Model. 35, 2805–2819 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  6. Kaushik, A., Sharma, K.K., Sharma, M.: A parameter uniform difference scheme for parabolic partial differential equation with a retarded argument. Appl. Math. Model. 34, 4232–4242 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  7. Kaushik, A., Sharma, M.: A robust numerical approach for singularly perturbed time delayed parabolic partial differential equations. Comput. Math. Model. 23(1), 96–106 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  8. Das, A., Natesan, S.: Uniformly convergent hybrid numerical scheme for singularly perturbed delay parabolic convection–diffusion problems on Shishkin mesh. Appl. Math. Comput. 271(15), 168–186 (2015)

    MathSciNet  MATH  Google Scholar 

  9. Gowrisankar, S., Natesan, S.: \(\epsilon \)-Uniformly convergent numerical scheme for singularly perturbed delay parabolic partial differential equations. Int. J. Comput. Math. 94(5), 902–921 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  10. Chakravarthy, P.P., Kumar, K.: An adaptive mesh method for time dependent singularly perturbed differential-difference equations. Nonlinear Eng. 8(1), 328–339 (2019)

    Article  Google Scholar 

  11. Kumar, P.M.M., Ravi Kanth, A.S.V.: Computational study for a class of time-dependent singularly perturbed parabolic partial differential equation through tension spline. Comput. Appl. Math. 39(3), 1–19 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  12. Gürbüz, B.: A computational technique for solving singularly perturbed delay partial differential equations. Found. Comput. Decis. Sci. 46(3), 221–233 (2021)

    Article  Google Scholar 

  13. Parthiban, S., Valarmathi, S., Franklin, V.: A numerical method to solve singularly perturbed linear parabolic second order delay differential equation of reaction–diffusion type. Malaya J. Mat. 2, 412–420 (2015)

    MATH  Google Scholar 

  14. Yadav, S., Rai, P., Sharma, K.K.: A higher order uniformly convergent method for singularly perturbed parabolic turning point problems. Numer. Methods Partial Differ. Equ. 36(2), 342–368 (2020)

    Article  MathSciNet  Google Scholar 

  15. Izadi, M., Yüzbaşi, Ş: A hybrid approximation scheme for 1-D singularly perturbed parabolic convection–diffusion problems. Math. Commun. 27(1), 47–62 (2022)

    MathSciNet  MATH  Google Scholar 

  16. Gobena, W.T., Duressa, G.F.: Parameter uniform numerical methods for singularly perturbed delay parabolic differential equations with non-local boundary condition. Int. J. Eng. Sci. Technol. 13(2), 57–71 (2021)

    Article  MATH  Google Scholar 

  17. Daba, I.T., Duressa, G.F.: An efficient computational method for singularly perturbed delay parabolic partial differential equations. Int. J. Math. Models Methods Appl. Sci. 15, 105–117 (2021)

    MATH  Google Scholar 

  18. Daba, I.T., Duressa, G.F.: Hybrid algorithm for singularly perturbed delay parabolic partial differential equations. Appl. Appl. Math. 16(1), 397–416 (2021)

    MathSciNet  MATH  Google Scholar 

  19. Duressa, G.F., Woldaregay, M.M.: Fitted numerical scheme for solving singularly perturbed parabolic delay partial differential equations. Tamkang J. Math. 53 (2022)

  20. Woldaregay, M.M., Duressa, G.F.: Uniformly convergent numerical scheme for singularly perturbed parabolic delay differential equations. ITM Web Conf. 34, 02011 (2020)

    Article  Google Scholar 

  21. Woldaregay, M.M., Duressa, G.F.: Uniformly convergent numerical method for singularly perturbed delay parabolic differential equations arising in computational neuroscience. Kragujevac J. Math. 46(1), 65–84 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  22. Woldaregay, M.M., Duressa, G.F.: Parameter uniform numerical method for singularly perturbed parabolic differential difference equations. J. Niger. Math. Soc. 38(2), 223–245 (2019)

    MathSciNet  MATH  Google Scholar 

  23. Woldaregay, M.M., Duressa, G.F.: Accurate numerical scheme for singularly perturbed parabolic delay differential equation. BMC. Res. Notes 14, 358 (2021)

    Article  MATH  Google Scholar 

  24. Nagero, N., Duressa, G.F.: An efficient numerical approach for singularly perturbed parabolic convection–diffusion problems with large time-lag. J. Math. Model. 10(2), 173–190 (2022)

    MathSciNet  Google Scholar 

  25. Kumar, D.: A parameter-uniform scheme for the parabolic singularly perturbed problem with a delay in time. Numer. Methods Partial Differ. Equ. 37, 626–642 (2020)

    Article  MathSciNet  Google Scholar 

  26. Shivhare, M., Chakravarthy, P.P., Kumar, D.: A uniformly convergent quadratic B-spline collocation method for singularly perturbed parabolic partial differential equations with two small parameters. J. Math. Chem. 59, 186–215 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  27. Govindarao, L., Mohapatra, J.: A second order numerical method for singularly perturbed delay parabolic partial differential equation. Eng. Comput. 36(2), 420–444 (2019)

    Article  Google Scholar 

  28. Kumar, D.: An implicit scheme for singularly perturbed parabolic problem with retarded terms arising in computational neuroscience. Numer. Methods Partial Differ. Equ. 34, 1933–1952 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  29. Farrell, P.A., Hegarty, A.F., Miller, J.J.H., O’Riordan, E., Shishkin, G.I.: Robust Computational Techniques for Boundary Layers. Chapman & Hall, London (2000)

    Book  MATH  Google Scholar 

  30. Doolan, E.P., Miller, J.J.H., Schilderr, W.H.A.: Uniform Numerical Methods for Problems with Initial and Boundary Layers. Boole Press, Dublin (1980)

    Google Scholar 

  31. O’Malley, R.E.: Introduction to Singular Perturbations. Academic Press, New York (1974)

    MATH  Google Scholar 

  32. Chawla, M.M.: A fourth-order tridiagonal finite difference method for general non-linear two-point boundary value problems with mixed boundary conditions. J. Inst. Math. Appl. 21, 83–93 (1978)

    Article  MathSciNet  MATH  Google Scholar 

  33. Varga, R.S.: Matrix Iterative Analysis. Prentice-Hall Inc, Englewood Cliffs, NJ (1962)

    MATH  Google Scholar 

  34. Young, D.M.: Iterative Solution of Large Linear Systems. Academic Press, New York (1971)

    MATH  Google Scholar 

Download references

Acknowledgements

Authors are grateful to the anonymous referees for their valuable suggestions and comments that improved the quality of this paper.

Funding

The authors wish to thank the National Board for Higher Mathematics, Department of Atomic Energy, Government of India, for their financial support under the project No. 02011/8/2021 NBHM(R.P)/R &D II/7224, dated 24.06.2021.

Author information

Authors and Affiliations

  1. Department of Mathematics, School of Advanced Sciences, Vellore Institute of Technology, Vellore, Tamil Nadu, 632 014, India

    T. Prathap & R. Nageshwar Rao

Authors
  1. T. Prathap
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. R. Nageshwar Rao
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to R. Nageshwar Rao.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest, relevant to the content of this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Prathap, T., Rao, R.N. Uniformly convergent finite difference methods for singularly perturbed parabolic partial differential equations with mixed shifts. J. Appl. Math. Comput. 69, 1679–1704 (2023). https://doi.org/10.1007/s12190-022-01802-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-022-01802-2

Keywords

Mathematics Subject Classification