Channel characterization of time reversal UWB communication systems

Abstract

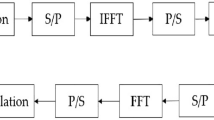
An ultra wideband (UWB) communications system that applies time reversal to transmit the desired signal is investigated. Exact expressions for the first- and second-order moments, cross-correlation, intersymbol interference metric, and correlation coefficient of time reversal (TR) UWB equivalent channel are derived in terms of the physical channel parameters such as delay spread and mean excess delay. These expressions are verified by simulated and experimental results. It is shown that TR-UWB excess delay is very smaller than UWB and its delay spread decreases as signaling bandwidth increases. Semi-analytical results show that the time reversal UWB delay spread is approximately the same as UWB. Furthermore, an ISI metric is derived for TR-UWB channel based on transmitted signal and UWB channel parameters. Moreover, correlation coefficient of two TR-UWB received signals with different power delay profile is computed analytically. Simulation and analytical results show that for τ > 0.3T _w correlation coefficient is below 0.25 and for τ > T _w correlation coefficient is zero, where T _w is the transmitted pulse width. Finally, theoretical performance of a receiver with one tap matched filter is computed and compared with measured and simulated result.

Appendices

Appendix 1—autocorrelation

Aim of this appendix is the derivation of the autocorrelation of the received pulse in TR-UWB defined as: $R\left( {{t_1},{t_2}} \right) = E\left( {{h_{\rm{eq}}}({t_1})h_{\rm{eq}}^{*}({t_2})} \right)$. Since E{α _i α _j } = 0, and $E\left\{ {\alpha_i^3{\alpha_j}} \right\} = 0$, $E\left\{ {\alpha_i^2\alpha_j^2} \right\} = E\left\{ {\alpha_i^2} \right\}E\left\{ {\alpha_j^2} \right\}$ for i ≠ j, similar to [3], autocorrelation is

$$ {R_I}({t_1},{t_2}) = E\left( {{h_{\rm{eq}}}({t_1})h_{\rm{eq}}^{*}({t_2})} \right)\,) = E\left\{ {\int\limits_A {\int\limits_A {h(} } t)h(\mu )h(t + {t_1})h(\mu + {t_2})dtd\mu } \right\} $$

(53)

Using uncorrelated scattering properties

$$ {R_I}({t_1},{t_2}) = E\left\{ {\int\limits_A {\int\limits_A {\sum\limits_i {\sum\limits_j {\sum\limits_k {\sum\limits_e {{\alpha_i}{\alpha_j}{\alpha_k}{\alpha_e}w(t - {t_i})w(\mu - {t_j}) \times w(t + {t_1} - {t_k})w(t + {t_2} - {t_e})dt\,d\mu } } } } } } } \right\}\, $$

(54)

$$ \begin{gathered} {R_I}({t_1},t_2) = E\left\{ {\int\limits_A {\int\limits_A {\sum {\alpha_i^2} } } } \right.w(t - {t_i}) \times \, \hfill \\\left[ {w(t + {t_1} - {t_i})\sum\limits_k {\alpha_k^2w(\mu - {t_i})w(\mu + {t_2} - {t_k})} } \right. \hfill \\+ w(\mu - {t_i})\sum\limits_{g,j \ne i} {\alpha_g^2w(t + {t_1} - {t_j})w(\mu + {t_2} - {t_j})} \hfill \\\left. {\left. { + w(\mu + {t_2} - {t_i})\sum\limits_{j,j \ne i} {\alpha_j^2} w(t + {t_1} - {t_j})w(\mu - {t_i})} \right]dt\,d\mu } \right\} \hfill \\\end{gathered} $$

(55)

We study the three terms in the above equation, and we denote each one with the corresponding roman number.

The first term can be rewritten as follows

$$ \begin{gathered} I = E\left\{ {\sum\limits_i {\alpha_i^2} } \right.\int\limits_A {w(t - {t_i}} )w(t + {t_1} - {t_i})dt\sum\limits_k {\alpha_k^2} \int\limits_A {w(\mu - {t_k}} )w(\mu + {t_2} - {t_k})d\mu \hfill \\= E\left\{ {\sum\limits_i {\alpha_i^2} } \right.\left. {\sum\limits_k {a_k^2} } \right\}{\varphi_w}({t_1}){\varphi_w}({t_2}) \hfill \\\end{gathered} $$

(56)

where ${\varphi_w}(t) = \int\limits_A {w(\tau } )w(t + \tau )d\tau \,$.

Expectation and variance of $\sum {\alpha_i^2}$ is [3]

$$ \begin{gathered} E\left\{ {\sum {\alpha_i^2} } \right\} = \int\limits_A {{P_g}(t)dt} \, \hfill \\{\hbox{Var}}\left\{ {\sum {\alpha_i^2} } \right\} = \int\limits_A {{R_g}(t)dt} \hfill \\\end{gathered} $$

(57)

Using (57), E {Σ α _i }² is [3]

$$ E{\left\{ {\sum {{\alpha_i}} } \right\}^2} = {\hbox{Var}}\left\{ {\sum {\alpha_i^2} } \right\} + {\left( {E\left\{ {\sum {\alpha_i^2} } \right\}} \right)^2} = \int\limits_A {{R_g}} (t)dt + \int\limits_A {\int\limits_A {{P_g}} (t){P_g}(\mu )dtd\mu } $$

(58)

Using (58), (56) can be written as

$$ I = {\varphi_w}({t_1}){\varphi_w}({t_2})\left\{ {\int\limits_A {{R_g}} (t)dt + \int\limits_A {\int\limits_A {{P_g}} (t){P_g}(\mu )dtd\mu } } \right\} $$

(59)

The second term in (55) is

$$ II = \int\limits_A {\int\limits_A {E\left\{ {\sum\limits_1 {\alpha_i^2w(t - {t_i})w(\mu - {t_i})} } \right\}} } E\left\{ {\sum\limits_{J,J \ne i} {\alpha_j^2w(t + {t_1} - {t_j})w(\mu + {t_2} - {t_j})dtd\mu } } \right\} $$

(60)

As it is known

$$ E\left\{ {\sum\limits_i {\alpha_i^2w(t - {t_i})w(\mu - {t_i})} } \right\} = E\left\{ {g(t)g(\mu )} \right\}\, $$

(61)

$$ E\left\{ {\sum\limits_{I,J} {\alpha_i^2w(t + {t_1} - {t_i})w(\mu + {t_2} - {t_1})} } \right\} = E\left\{ {g(t + {t_1})g(\mu - {t_2})} \right\} $$

(62)

By supposing $\mu = t + \xi$, it is shown that $E\left\{ {(g(t)g(t + \xi } \right\} = {p_g}(t){\phi_w}(\xi )$, so

$$ \begin{gathered} II = \int\limits_A {\int\limits_{A\prime} {E\left\{ {g(t)g(\mu )} \right\}E\left\{ {g(t + {t_1})g(\mu + {t_2})} \right\}} } dt\,d\mu \, \hfill \\= \int\limits_A {\int\limits_{A\prime} {{P_g}(t){\phi_w}(\mu - t){P_g}(t + {t_1}){\varphi_w}(t + {t_1} - \mu - {t_2})dtd\mu } } \hfill \\= \int\limits_A {{P_g}} (t){P_g}(t + {t_1})dt\int\limits_{A\prime} {{\phi_w}} (\xi ){\phi_w}(\xi + {t_1} - {t_2})d\xi \hfill \\= {c_1}{\phi_{{\phi_W}}}({t_2} - {t_1})\int\limits_A {{P_g}(t){P_g}(t + {t_1})dt} \hfill \\\end{gathered} $$

(63)

where $A\prime = \left[ {a - t,a - t + {\tau_I}} \right]\,$.

The third term, the following expression is

$$ III = E\left\{ {\int\limits_A {\int\limits_A {\sum\limits_i {\alpha_i^2w(t - {t_i})w(\mu + {t_2} - {t_i})} } } } \right.\left. {\sum\limits_{j,j \ne i} {w(t + {t_2} - {t_j})w(\mu - {t_j})dtd\mu } } \right\} $$

(64)

$$ \begin{gathered} III \approx \int\limits_A {\int\limits_{A\prime} {E\left( {g(t)g(t + {t_1} + \varepsilon )} \right)E\left( {g(t + {t_2})g(t + \varepsilon )} \right)dtd\varepsilon } } \hfill \\= \int\limits_A {\int\limits_{A\prime} {{p_g}(t){\phi_w}({t_1} + \varepsilon ){p_g}(t + {t_2}){\phi_w}(\varepsilon - {t_2})dtd\varepsilon } } \, \hfill \\= \int\limits_A {{p_g}} (t){p_g}(t + {t_2})dt\int\limits_{A\prime} {{\phi_w}({t_1} + \varepsilon ){\phi_w}(\varepsilon - {t_2})d\varepsilon } \hfill \\= {c_1}{\phi_{{\phi_W}}}({t_1} + {t_2})\int\limits_A {{P_g}} (t){P_g}(t + {t_2})dt \hfill \\\end{gathered} $$

(65)

And obtain (13) from (59), (63), and (65).

Appendix 2—means excess delay and delay spread

Aim of this appendix is the derivation of the mean excess delay in TR-UWB systems based on UWB mean excess delay and UWB PDP. In observation interval of A = [0,∞], by substituting (27) in (26) mean excess delay is

$$ {\bar{\tau }_{\rm{TR}}} = \frac{1}{{\int\limits_{ - \infty }^\infty {pdp(\tau )d\tau } }}\int\limits_{ - \infty }^\infty {\tau \,pdp(\tau )d\tau } \approx \frac{{{c_1}}}{{\int\limits_{ - \infty }^\infty {pdp(\tau )d\tau } }}\int\limits_{{T_w}}^\infty {\tau \int_A {P_g} (t){P_g}(t + \tau )dt\,d\tau } $$

(66)

$$ {\bar{\tau }_{\rm{TR}}}\int\limits_{ - \infty }^\infty {pdp(\tau )d\tau } \approx {c_1}\int_A {{P_g}} (t)\left( {\int\limits_{{T_w}}^\infty \tau {P_g}(t + \tau )d\tau } \right)dt $$

(67)

Supposing that $t + \tau = \beta$ and substituting (28) in (67)

$$ \begin{gathered} \int\limits_{{T_w}}^\infty \tau {P_g}(t + \tau )d\tau = \int\limits_{{T_w}}^\infty {\left( {\beta - t} \right)} {P_g}(\beta )d\beta = \int\limits_{{T_w}}^\infty \beta {P_g}(\beta )d\beta - t\int\limits_{{T_w}}^\infty {{P_g}(\beta )d\beta } \hfill \\= {E_h}\left( {T + {T_w} - t} \right){e^{ - \frac{{{T_w}}}{T}}} \hfill \\\end{gathered} $$

(68)

By supposing ${c_1}{\bar{\tau }_{\phi_{_w}^2}} = \int\limits_{ - \infty }^\infty \tau \phi_w^2(\tau )d\tau$

$$ {\bar{\tau }_{\rm{TR}}}\int\limits_{ - \infty }^\infty {pdp(\tau )d\tau } = {c_1}{E_h}{e^{ - \frac{{{T_w}}}{T}}}\int_A {\left( {T + {T_w} - t} \right){P_g}} (t)dt\, $$

(69)

In observation interval of A = [0,∞]

$$ \begin{gathered} {{\bar{\tau }}_{\rm{TR}}}\int\limits_{ - \infty }^\infty {pdp(\tau )d\tau } = {c_1}{E_h}{e^{ - \frac{{{T_w}}}{T}}}\int\limits_0^\infty {\left( {T + {T_w} - t} \right)} {P_g}(t)dt \hfill \\= {c_1}E_h^2{e^{ - \frac{{{T_w}}}{T}}}\left( {T + {T_w} - \int\limits_0^\infty {\frac{t}{T}{e^{ - \frac{t}{T}}}} dt\,} \right)\,\, \hfill \\= {c_1}E_h^2\left( {T - T + {T_w}} \right){e^{ - \frac{{{T_w}}}{T}}} = {c_1}E_h^2{T_w}{e^{ - \frac{{{T_w}}}{T}}} \hfill \\\end{gathered} $$

(70)

And the TR-UWB PDP is

$$ pdp(\tau ) = pd{p_{\text{TR}}}(\tau )\, \approx \,E_h^2\left\{ {\begin{array}{*{20}{c}} {\frac{{{c_1} + {c_2}}}{T}} & {\,\tau = 0} \\ {\frac{{{c_1}}}{{2T}}{e^{ - \frac{\tau }{T}}}} & {\tau \geqslant {T_w}} \\ \end{array} } \right. $$

(71)

Therefore,

$$ {\bar{\tau }_{\rm{TR}}} \approx \frac{{E_h^2{c_1}{T_w}{e^{ - \frac{{{T_w}}}{T}}}}}{{\int\limits_{ - \infty }^\infty {pdp(\tau )d\tau } }} = \frac{{E_h^2{c_1}{T_w}{e^{ - \frac{{{T_w}}}{T}}}}}{{E_h^2\left( {\frac{{{c_1} + {c_2}}}{T}{T_w} + \frac{{{c_1}}}{2}{e^{ - \frac{{{T_w}}}{T}}}} \right)}}\, \approx \frac{{{c_1}{T_w}{e^{ - \frac{{{T_w}}}{T}}}}}{{\left( {\frac{{{c_1} + {c_2}}}{T}{T_w} + \frac{{{c_1}}}{2}{e^{ - \frac{{{T_w}}}{T}}}} \right)}} $$

(72)

It is obvious that $\frac{{{c_1} + {c_2}}}{T}{T_w} < < \frac{{{c_1}}}{2}{e^{ - \frac{{{T_w}}}{T}}}$, so

$$ {\bar{\tau }_{\rm{TR}}} \approx \frac{{{c_1}{T_w}{e^{ - \frac{{{T_w}}}{T}}}}}{{\frac{{{c_1}}}{2}{e^{ - \frac{{{T_w}}}{T}}}}} = 2{T_w} $$

(73)

As it is seen from (73), ${\bar{\tau }_{\rm{TR}}}$is mostly near zero. Because of symmetric property of equivalent channel impulse response of TR-UWB in observation interval of $A = [ - \infty \,,\,\infty ]$, its mean excess delay is zero.

TR-UWB delay spread (RMS delay) in observation interval of $A = [ - \infty \,,\,\infty ]$ is

$$ \begin{gathered} \tau_{{\rm{RMS - TRUWB}}}^2 = \frac{1}{{\int\limits_{ - \infty }^\infty {pd{p_{\rm{TR}}}(\tau )d\tau } }}\int\limits_{ - \infty }^\infty {{{(\tau - \bar{\tau })}^2}pd{p_{\rm{TR}}}(\tau )d\tau } \, \hfill \\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \approx \frac{1}{{\int\limits_{ - \infty }^\infty {pd{p_{\rm{TR}}}(\tau )d\tau } }}\int\limits_{ - \infty }^\infty {{\tau^2}pd{p_{\rm{TR}}}(\tau )d\tau } = \frac{{2\int\limits_0^\infty {{\tau^2}pd{p_{\rm{TR}}}(\tau )d\tau } }}{{2\int\limits_{ - \infty }^\infty {pd{p_{\rm{TR}}}(\tau )d\tau } }}\, \hfill \\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \approx \frac{{\frac{{{c_1}}}{{2T}}\int\limits_{{T_w}}^\infty {{\tau^2}{e^{ - \frac{\tau }{T}}}d\tau } \,}}{{\frac{{{c_1} + {c_2}}}{T}{T_w} + \frac{{{c_1}}}{2}{e^{ - \frac{{{T_w}}}{T}}}}} \hfill \\\end{gathered} $$

(74)

Using $\frac{{{c_1} + {c_2}}}{T}{T_w} < < \frac{{{c_1}{e^{ - \frac{{{T_w}}}{T}}}}}{2}$ and

$$ \frac{1}{T}\int\limits_{{T_w}}^\infty {{\tau^2}{e^{ - \frac{\tau }{T}}}d\tau } \approx \frac{1}{T}\int\limits_0^\infty {{\tau^2}{e^{ - \frac{\tau }{T}}}d\tau } = \tau_{{\rm{RMS - UWB}}}^2 $$

(75)

Replacing (76) in (75), TR-UWB delay spread is

$$ \begin{gathered} \tau_{{\rm{RMS - TRUWB}}}^2 \approx \frac{{\frac{{{c_1}}}{2}\tau_{{\rm{RMS - UWB}}}^2}}{{\frac{{{c_1}{e^{ - \frac{{{T_W}}}{T}}}}}{2}}} = {e^{\frac{{{T_w}}}{T}}}\tau_{{\rm{RMS - UWB}}}^2 \hfill \\{\tau_{{\rm{RMS - TRUWB}}}} \approx {e^{\frac{{{T_w}}}{{2T}}}}{\tau_{{\rm{RMS - UWB}}}} \hfill \\\end{gathered} $$

(76)