Abstract
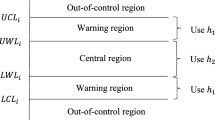
The theoretical results about variable sample size and sampling intervals (VSSI) Shewhart control chart are concerned with the case where the mean and standard deviation of the given process are known. However, in practice the mean and standard deviation are unknown. In this case, the common treatment is to estimate the unknown parameters using historical data. After determining the parameters we treat the control limits as though fixed. Up to now we have no idea about the average time to signal (ATS) of VSSI \({\bar{X}}\) charts when the control limits are estimated. This paper will calculate the mean of the ATS for VSSI \({\bar{X}}\) charts when the control limits are estimated in three different ways. The results show that parameter estimation has significant effect on the in-control ATS, but has little effect on the out-of-control ATS. These results could show us some good reference idea for choosing adequate number of sample groups m and the sample size n of historical data. Finally, this paper obtains the optimal design of the maximum sample size \(n_2\) when other parameters are fixed.
Similar content being viewed by others
References
Chen G (1997) The mean and standard deviation of the run length destribution of \({\bar{X}}\) charts when control limits are estimated. Stat Sin 7:789–798
Costa AFB (1994) \({\bar{X}}\) charts with variable sample size. J Qual Technol 26:155–163
Costa AFB (1997) \({\bar{X}}\) charts with variable sample size and sampling intervals. J Qual Technol 29:197–204
Costa AFB (1999) \({\bar{X}}\) charts with variable parameters. J Qual Technol 31:408–416
Hillier FS (1969) \({\bar{X}}\) and \(R\) chart control limits based on a small number of subgroups. J Qual Technol 1:17–26
Montgomety DC (1996) Introduction to statistical quality control, 3rd edn. Wiley, New York
Patnaik PB (1950) The use of mean range as an estimator of variance in statistical tests. Biometrika 37:78–87
Prabhu SS, Montgomery DC, Runger GC (1994) A combined adaptive sample size and sampling interval \({\bar{X}}\) control scheme. J Qual Technol 26:164–176
Prabhu SS, Runger GC, Keats JB (1993) An adaptive sample size \({\bar{X}}\) chart. Int J Prod Res 31:2895–2909
Proschan F, Savage IR (1960) Starting a control chart. Ind Qual Control 17:12–13
Quesenberry CP (1993) The effect of sample size on estimated limits for \({\bar{X}}\) and \(X\) control charts. J Qual Technol 25:237–247
Reynolds MR Jr, Amin RW, Arnold JC, Nachlas JA (1988) \({\bar{X}}\) charts with variable sampling intervals. Technometrics 30:181–192
Acknowledgments
This paper is supported by the NNSF of China Grants (11401573), the CAUC Tianjin Key Lab for Advanced Signal Processing Open Funds (2015ASP01).
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The author declares that there is no conflict of interests regarding the publication of this article.
Rights and permissions
About this article
Cite this article
Zhou, M. Variable sample size and variable sampling interval Shewhart control chart with estimated parameters. Oper Res Int J 17, 17–37 (2017). https://doi.org/10.1007/s12351-015-0214-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12351-015-0214-9