Analyzing an inventory model with two-level trade credit period including the effect of customers’ credit on the demand function using q-fuzzy number

Abstract

In this paper, an EOQ model for deteriorating items has been developed in infinite time horizon including two-level delay in payment in which one delay in payment (M) is offered to the retailer by the supplier and the another delay in payment (N) is offered by the retailer to all customers. Since the real business world is full of uncertainties and the supplier has to face different problems with the retailer, hence there may exist uncertainties in the credit period which is offered by the supplier to the retailer. Again, uncertainties may be linear or non-linear type. Till now, there is no standard fuzzy number by which linearity and non-linearity can be explored simultaneously. In this respect, a new type of fuzzy number known as q-fuzzy number has been introduced to consider linearity and non-linearity together and this is the novelty of the paper. On the other hand, the retailer intends to offer a credit period to all customers to give rise the demand of the items. So, here demand function depends on credit period and duration of offering the credit period. The aim of the retailer is that how much credit period be benefited to get maximum profit. Therefore the purpose of this model is to determine the optimal credit length for the customers and optimal replenishment cycle length. Also, the model has been discussed considering the situation when the retailer offers no credit to the customers. Then some theoretical results and an algorithm for defuzzification have been developed. Finally, some numerical examples have been carried out to interpret the model and a sensitivity analysis of the optimal solution has been provided with respect to some parameters.

Appendices

Appendix 1: Preliminaries

Here, we state some basic concepts which are eventual for the paper.

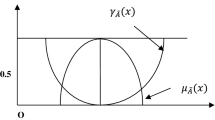
Definition 2.1

Let X be domain set. If $\tilde{A}$ is a fuzzy subset of X, for any $\chi \in X$

$\mu _{\tilde{A}}:X\rightarrow [0,1]$, $\chi \rightarrow \mu _{A}(\chi )$

$\mu _{\tilde{A}}$ is called a membership function of $\chi$ with respect to $\tilde{A}$, $\mu _{A}(\chi )$ denotes the grade to each point in X with a real number in the interval [0, 1] that represents the grade of membership of $\chi$ in A. $\tilde{A}$ is called a fuzzy set and describe as follows

$\tilde{A}=\{(\chi ,\mu _{A}(\chi ))|\chi \in X\}$.

Definition 2.2

A fuzzy number $\tilde{M}$ is a convex normalized fuzzy set $\tilde{M}$ of the real line $\mathfrak {R}$ such that

• (i) It exists exactly one $x_0\in \mathfrak {R}$ with $\mu _{\tilde{M}}(x_0)=1$ ( $x_0$ is called the mean value of $\tilde{M}$).

• (ii) $\mu _{\tilde{M}}(x)$ is piece wise continuous.

Definition 2.3

Let X and Y be the universes and $\tilde{P}(Y)$ be the set of all fuzzy sets in Y (power set), $\tilde{f}:X\rightarrow \tilde{P}(Y)$ is a mapping. Then $\tilde{f}$ is a fuzzy function iff

$$\begin{aligned} \mu _{\tilde{f}(x)}(y)=\mu _{\tilde{R}}(x,y),\qquad \forall (x,y)\in X \times Y \end{aligned}$$

where, $\mu _{\tilde{R}}(x,y)$ is the membership function of the fuzzy relation.

Definition 2.4

Let X be a cartesian product of universes $X= X_1, X_2, \ldots , X_r$ and $\tilde{A_1},\tilde{A_2},\ldots ,\tilde{A_r}$ be fuzzy sets in $X= X_1, X_2, \ldots , X_r$ respectively. Assume that f is a mapping from X to a universe Y, $y=f(x_1,x_2,\ldots ,x_r)$. Then the extension principle allows us to define a fuzzy set B in Y by

$$\begin{aligned} \tilde{B}=(y,\mu _{\tilde{B}}(y)): y=f(x_1,x_2,\ldots ,x_r), (x_1,x_2,\ldots ,x_r)\in X \end{aligned}$$

where,

$$\begin{aligned} \mu _{\tilde{B}}(y) =\left\{ \begin{array}{ll} \sup \limits _{x\in f^{-1}(y)}\mu _{\tilde{A}}(x); &{} \text{ if }\,f^{-1}(y)\ne \phi \\ 0; &{} \text{ otherwise}.\\ \end{array}\right. \end{aligned}$$

Appendix 2

$$\begin{aligned} \frac{dI(t)}{dt}=-D(t)-\theta {I(t)}\\ \frac{dI(t)}{dt}+\theta {I(t)}=-D(t). \end{aligned}$$

Integrating both side when $0\le t\le M-N$, we have

$$\begin{aligned} \int _{0}^{t}d(e^{\theta t}I(t)) &= -D_0\int _{0}^{t}e^{(b_1N(M-N)+\theta )t}dt\\ \text{ or, } e^{\theta t}I(t)-Q &= -\frac{D_0}{b_1N(M-N)+\theta }[e^{(b_1N(M-N)+\theta )t}-1]\\ \text{ or, }I(t) &= Qe^{-\theta t}-\frac{D_0}{b_{1}N(M-N)+\theta }[e^{b_1N(M-N)t}-e^{\theta t}] \end{aligned}$$

Again, integrating both side when $M-N\le t\le T$, we have

$$\begin{aligned} \int _{t}^{T}d(e^{\theta t}I(t)) &= -D_0 e^{b_2N(M-N)^2}\int _{t}^{T}e^{\theta t}dt\\ \text{ or, } -e^{\theta t}I(t) &= -\frac{D_0}{\theta } e^{b_2N(M-N)^2}[e^{\theta T}-e^{\theta t}]\\ \text{ or, } I(t) &= \frac{D_0}{\theta } e^{b_2N(M-N)^2}[e^{\theta (T-t)}-1]. \end{aligned}$$

Appendix 3

$$\begin{aligned} \int _{-\infty }^{\infty }x\mu _{\tilde{M}}(x)dx &= \int _{M_1}^{M_2} x\left(\frac{x-M_1}{M_2-M_1}\right)^qdx+\int _{M_2}^{M_3} x\left(\frac{M_3-x}{M_3-M_2}\right)^q dx\\ &= \frac{1}{(M_2-M_1)^q}\int _{0}^{M_2-M_1}(z_1+M_1)z_1^qdz_1\\&\quad +\frac{1}{(M_3-M_2)^q}\int _{0}^{M_3-M_2}(M_3-z_2)z_2^qdz_2\text{, }\text{ put } x-M_1=z_1\text{, } M_3-x=z_2\\ &= \frac{1}{(M_2-M_1)^q}\bigg [\frac{(M_2-M_1)^{q+2}}{q+2}+\frac{M_1(M_2-M_1)^{q+1}}{q+1}\bigg ]\\&\quad +\frac{1}{(M_3-M_2)^q}\bigg [\frac{M_3(M_3-M_2)^{q+1}}{q+1} -\frac{(M_3-M_2)^{q+2}}{q+2}\bigg ]\\ &= (M_2-M_1)\bigg [\frac{M_2-M_1}{q+2}+\frac{M_1}{q+1}\bigg ]+(M_3-M_2) \bigg [\frac{M_3}{q+1}-\frac{M_3-M_2}{q+2}\\ &= \frac{1}{q+1}(M_3-M_1)(M_3+M_1-M_2)+\frac{1}{q+2}(M_3-M_1)(2M_2-M_1-M_3)\\ \int _{-\infty }^{\infty }\mu _{\tilde{M}}(x)dx &= \int _{M_1}^{M_2}\left(\frac{x-M_1}{M_2-M_1}\right)^qdx+\int _{M_2}^{M_3}\left(\frac{M_3-x}{M_3-M_2}\right)^qdx\\ &= \frac{1}{(M_2-M_1)^q}{\bigg [\frac{(x-M_1)^{q+1}}{q+1} \bigg ]_{M_1}}^{M_2}-\frac{1}{(M_3-M_2)^q}{\bigg [ \frac{(M_3-x)^{q+1}}{q+1}\bigg ]_{M_2}}^{M_3}\\ &= \frac{1}{q+1}(M_2-M_1+M_3-M_2)\\ &= \frac{1}{q+1}(M_3-M_1). \end{aligned}$$

Appendix 4

The first order derivatives of the Eq. (14) with respect to T is given by

$$\begin{aligned} \frac{dTP2(T)}{dT}=\frac{sI_eD_0}{2}-\frac{pD_0\theta }{2} -\frac{pI_cD_0}{2}\left(1-\frac{M^2}{T^2}\right)-hD_0+\frac{A}{T^2} \end{aligned}$$

The second order derivatives of the Eq. (14) with respect to T is given by

$$\begin{aligned} \frac{d^2TP2(T)}{dT^2}=-\frac{pI_cD_0}{T^3}-\frac{2A}{T^3}. \end{aligned}$$