Credit financing and channel encroachment: analysis of distribution choice in a dual-channel supply chain

Abstract

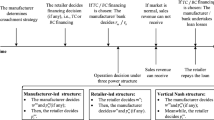
Supply chain finance plays a significant role in alleviating capital shortage, which optimizes supply chain performance. In this paper, we discuss the interaction of credit financing and channel encroachment in a dual-channel supply chain structure consisting of a supplier and a retailer. Under the Stackelberg structure, we observe the interaction between credit financing and channel encroachment is heavily dependent on the substitution degree, potential online market, and production cost. Intuitively, the supplier is more likely to choose trade credit financing, except in the case where both the potential online market, substitution degree, and production cost are small; under these conditions, bank credit financing may be an equilibrium strategy. As long as the production cost is below a certain threshold, the supplier will choose the trade credit financing strategy.

Appendix

1.1 Proof of Proposition 1

Under the scenario without capital constraint for supplier, we have

$$\begin{aligned} \pi_{r}^{NN} = & \frac{{\left[ {1 - a - \left( {1 - \delta } \right)c} \right]^{2} }}{16} \\ \pi_{r}^{NE} = & \pi_{r}^{NN} + \frac{a}{64}\left\{ {8\left( {1 + c\delta } \right) + a\left[ { - 58 + \left( {35 - 4\delta } \right)\delta } \right]} \right\} \\ & - \frac{1}{16}\left\{ {3(2 - \delta ) + \left[ {\delta + c\left( { - 2 + \delta + \delta^{2} } \right)} \right]^{2} } \right\} - \frac{a}{4}\left[ {\frac{1 + 2c}{{4(2 - \delta )}} + \frac{3}{2 + \delta }} \right] \\ \pi_{s}^{NN} = & \frac{{1 + \delta + \left( {1 - \delta } \right)\left( {1 - 2a} \right)^{2} }}{{8\left( {1 - \delta^{2} } \right)}} \\ & + \frac{1}{8}\left\{ { - 2c\left[ {1 + \delta + \left( {1 - \delta } \right)a} \right] + c^{2} \left( {1 - \delta } \right)\left( {3 + \delta } \right) - \left( {1 - a} \right)^{2} } \right\} \\ \end{aligned}$$

$$\begin{aligned} \pi_{s}^{NN} = & \frac{{1 + \delta + \left( {1 - \delta } \right)\left( {1 - 2a} \right)^{2} }}{{8\left( {1 - \delta^{2} } \right)}} \\ & + \frac{1}{8}\left\{ { - 2c\left[ {1 + \delta + \left( {1 - \delta } \right)a} \right] + c^{2} \left( {1 - \delta } \right)\left( {3 + \delta } \right) - \left( {1 - a} \right)^{2} } \right\} \\ \end{aligned}$$

$$\begin{aligned} \pi_{s}^{NE} = & \pi_{S}^{NN} + \frac{{\left( {2 - 3a} \right)^{2} }}{{48\left( {2 + \delta } \right)}} - \frac{{\left[ {2\left( {1 + 2c} \right) - a} \right]^{2} }}{{32\left( {2 - \delta } \right)}} \\ & + \frac{1}{8}\left\{ {\left( {1 - a} \right)\left( {1 - a + 2c\delta } \right) + c\left[ {2 + c\left( {3 + \delta^{2} } \right)} \right] - \frac{1}{{3\left( {1 - \delta } \right)}} - \frac{{\left( {1 - 2a} \right)^{2} }}{1 + \delta }} \right\} \\ \end{aligned}$$

Under BCF scenario and retailer does not encroach the online market. In this case, the retailer’s profit is as $\pi_{r} = \left( {p - w} \right)D_{r}^{{\text{N}}}$. In third stage, the retailer decides on her retail price. With $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {p^{BN} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{r}}} /\partial p^{BN} = 0$, we can derive expression of the optimal retail price in relation to the supplier’s direct price and wholesale price is $p^{BN} = \frac{1 - a + w + d\delta }{2}$. In second stage, the supplier decides on his direct price and wholesale price. With $\partial^{2} {\uppi }_{{\text{s}}} /\partial (d^{BN} )^{2} < 0$ and $\partial^{2} {\uppi }_{{\text{s}}} /\partial (w^{BN} )^{2} < 0$, $\partial {\uppi }_{{\text{s}}} /\partial d^{BN} = 0$ and $\partial {\uppi }_{{\text{s}}} /\partial w^{BN} = 0$, we can derive expressions of the optimal direct price and wholesale price in relation to the creditor’s interest rate are $w^{BN} = \frac{ - 1 + a - a\delta }{{2\left( { - 1 + \delta^{2} } \right)}} + \frac{{c\left( {1 + I_{BN} } \right)}}{2},d^{BN} = \frac{{a\left( { - 1 + \delta } \right) - \delta }}{{2\left( { - 1 + \delta^{2} } \right)}} + \frac{{c\left( {1 + I_{BN} } \right)}}{2}$. In first stage, the creditor sets interest rate. With $\partial^{2} {\uppi }_{{\text{b}}} /\partial \left( {I_{BN} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{b}}} /\partial I_{BN} = 0$, we solve the optimal interest rate $I_{BN} = \frac{{1 + \delta + a\left( {1 - \delta } \right)}}{{2c\left( {1 - \delta } \right)\left( {3 + \delta } \right)}} - \frac{1}{2}$. Then taking $I_{BN}$ to $w^{BN}$ and $d^{BN}$, we can get the optimal wholesale price $w^{BN} = - \frac{{7 + 4\delta + \delta^{2} + a\left( { - 5 + 4\delta + \delta^{2} } \right)}}{{4\left( {3 + \delta } \right)\left( { - 1 + \delta^{2} } \right)}} + \frac{c}{4}$ and direct price $d^{BN} = \frac{{ - 1 - 8\delta - 3\delta^{2} + a\left( { - 7 + 4\delta + 3\delta^{2} } \right)}}{{4\left( {3 + \delta } \right)\left( { - 1 + \delta^{2} } \right)}} + \frac{c}{4}$. Next, we take $w^{BN}$ and $d^{BN}$ to $p^{BN}$, we can get the optimal retail price $p^{BN} = \frac{{ - 19 - 9\delta + 3\delta^{2} + \delta^{3} - a\left( { - 17 + 7\delta + 9\delta^{2} + \delta^{3} } \right)}}{{8\left( {3 + \delta } \right)\left( { - 1 + \delta^{2} } \right)}} + \frac{{c\left( {1 + \delta } \right)}}{8}$. Finally, we can derive out the participates’ profits as $\pi_{b}^{{{\text{BN}}}} = \frac{{c^{2} \left( {1 - \delta } \right)\left( {3 + \delta } \right)}}{4}I_{{{\text{BN}}}}^{2}$, $\pi_{s}^{{{\text{BN}}}} = \pi_{s}^{{{\text{NN}}}} - \frac{{3c^{2} \left( {1 - \delta } \right)\left( {3 + \delta } \right)}}{8}I_{{{\text{BN}}}}^{2}$ and $\pi_{r}^{{{\text{BN}}}} = \frac{{\left[ {1 - a - c\left( {1 - \delta } \right)\left( {1 + I_{{{\text{BN}}}} } \right)} \right]^{2} }}{16}$.

1.2 Proof of Proposition 2

Under BCF scenario and retailer encroaches the online market. In this case, the retailer’s profit is as $\pi_{r} = \left( {p - w} \right)D_{r}^{{\text{E}}} + \left( {b - w} \right)Q_{r}^{{\text{E}}}$. In third stage, the retailer decides on her retail price and booking price. With $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {p^{BE} } \right)^{2} < 0$, $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {b^{BE} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{r}}} /\partial p^{BE} = 0$, $\partial {\uppi }_{{\text{r}}} /\partial b^{BE} = 0$, we can derive expression of the optimal retail price $p^{BE} = \frac{{4 + a\left( { - 4 + \delta } \right) + 2d\delta + d\delta^{2} }}{{8 - 2\delta^{2} }} + \frac{w}{2}$ and booking price $b^{BE} = \frac{{2a\left( { - 1 + \delta } \right) - \delta \left( {2 + d\left( {2 + \delta } \right)} \right)}}{{2\left( { - 4 + \delta^{2} } \right)}} + \frac{w}{2}$ in relation to the supplier’s direct price and wholesale price. In second stage, the supplier decides on his direct price and wholesale price. With $\partial^{2} {\uppi }_{{\text{s}}} /\partial (d^{BE} )^{2} < 0$ and $\partial^{2} {\uppi }_{{\text{s}}} /\partial (w^{BE} )^{2} < 0$, $\partial {\uppi }_{{\text{s}}} /\partial d^{BE} = 0$ and $\partial {\uppi }_{{\text{s}}} /\partial w^{BE} = 0$, we can derive expressions of the optimal direct price and wholesale price in relation to the creditor’s interest rate are $d^{BE} = \frac{{a\left( { - 1 + \delta } \right) - \delta }}{{2\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{{c\left( {1 + I_{BE} } \right)}}{2},w^{BE} = \frac{ - 2 + a - a\delta }{{4\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{{c\left( {1 + I_{BE} } \right)}}{2}$. In first stage, the creditor sets interest rate. With $\partial^{2} {\uppi }_{{\text{b}}} /\partial \left( {I_{BE} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{b}}} /\partial I_{BE} = 0$, we solve the optimal interest rate $I_{{{\text{BE}}}} = \frac{{a\left( {1 - \delta } \right) + 2}}{{4c\left( {1 - \delta } \right)\left( {4 - \delta } \right)}} - \frac{1}{2}$. Then taking $I_{BE}$ to $w^{BE}$ and $d^{BE}$, we can get the optimal wholesale price $w^{BE} = \frac{{20 - 2\delta - 3a\left( {2 - 3\delta + \delta^{2} } \right)}}{{8\left( { - 4 + \delta } \right)\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{c}{4}$ and direct price $d^{BE} = \frac{{4 + 18\delta - 4\delta^{2} + 3a\left( {6 - 7\delta + \delta^{2} } \right)}}{{8\left( { - 4 + \delta } \right)\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{c}{4}$. Next, we take $w^{BE}$ and $d^{BE}$ to $p^{BE}$, we can get the optimal retail price $p^{BE} = \frac{{ - 84 + 90\delta - 26\delta^{2} + 2\delta^{3} + a\left( {70 - 117\delta + 54\delta^{2} - 7\delta^{3} } \right)}}{{8\left( { - 4 + \delta } \right)\left( { - 1 + \delta } \right)\left( { - 4 + \delta^{2} } \right)}} + \frac{c}{{4\left( {2 - \delta } \right)}}$. Finally, we derive the participates’ profits as $\pi_{b}^{{{\text{BE}}}} = \frac{{c^{2} \left( {1 - \delta } \right)\left( {4 - \delta } \right)}}{{2\left( {2 - \delta } \right)}}I_{{{\text{BE}}}}^{2}$,${ }\pi_{s}^{{{\text{BE}}}} = \pi_{s}^{{{\text{NE}}}} - \frac{3}{2}\pi_{b}^{{{\text{BE}}}}$ and.

1.3 Proof of Lemma 1

When ${ }c \in \left[ {0,} \right.\left. {\overline{c}} \right)$,$a \in \left[ {0,} \right.\left. {\overline{a}} \right)$, the retailer will encroach the online market. $\begin{gathered} { }\pi_{r}^{BE} - \pi_{r}^{BN} = \frac{1}{{\left( { - 4 + \delta } \right)^{2} \left( {3 + \delta } \right)^{2} \left( { - 4 + \delta } \right)^{2} }}\left[ { - c^{2} \left( { - 4 + \delta } \right)^{2} \left( { - 1 + \delta } \right)^{2} \left( {2 + \delta } \right)^{2} \left( {3 + \delta } \right)^{2} } \right. \hfill \\ + 2c\left( { - 4 + \delta } \right)\left( { - 1 + \delta } \right)\left( {2 + \delta } \right)\left( {3 + \delta } \right)\left( { - 4 + 26a + 22\delta - 32a\delta - 3\delta^{2} + 5a\delta^{2} - \delta^{3} + a\delta^{3} } \right) \hfill \\ - 3656 + 10424a - 7682a^{2} + 1052\delta - 3436a\delta + 2619a^{2} \delta + 708\delta^{2} - 2180a\delta^{2} \hfill \\ + 1696a^{2} \delta^{2} - 184\delta^{3} + 580a\delta^{3} - 441a^{2} \delta^{3} - 29\delta^{4} + 114a\delta^{4} - 105a^{2} \delta^{4} \hfill \\ \left. { + 10\delta^{5} - 32a\delta^{5} + 26a^{2} \delta^{5} - \delta^{6} + 2a\delta^{6} - a^{2} \delta^{6} } \right]. \hfill \\ \hfill \\ \end{gathered}$.

We simplify its numerator to a quadratic function with $c$ as its independent variable, then we write the numerator as ${\text{f}}\left( {\text{c}} \right)$. We can obtain that $\Delta < 0$ and the quadratic coefficient $- \left( { - 4 + \delta } \right)^{2} \left( { - 1 + \delta } \right)^{2} \left( {2 + \delta } \right)^{2} \left( {3 + \delta } \right)^{2} < 0$. According to the discriminant of the root of quadratic equation with one variable and the opening direction of function, we can know that $f\left( c \right)$ is always smaller than 0. But $\pi_{r}^{BE} - \pi_{r}^{BN} = \frac{1}{{\left( { - 4 + \delta } \right)^{2} \left( {3 + \delta } \right)^{2} \left( { - 4 + \delta^{2} } \right)}}f\left( c \right)$ and $\frac{1}{{\left( { - 4 + \delta } \right)^{2} \left( {3 + \delta } \right)^{2} \left( { - 4 + \delta^{2} } \right)}} < 0$. Therefore, $\pi_{r}^{BE} > \pi_{r}^{BN}$.

1.4 Proof of Proposition 3

Under TCF scenario and retailer does not encroach the online market. In this case, the retailer’s profit is as $\pi_{r} = \left( {p - w} \right)D_{r}^{{\text{N}}} + cI_{TN} \left( {D_{s}^{{\text{N}}} + D_{r}^{{\text{N}}} } \right)$. In third stage, the retailer decides on her retail price. With $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {p^{TN} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{r}}} /\partial p^{TN} = 0$, we can derive expression of the optimal retail price in relation to the supplier’s direct price and wholesale price and interest rate is $p^{TN} = \frac{{\left[ {1 - a + w + cI_{TN} \left( { - 1 + \delta } \right) + d\delta } \right]}}{2}$. In second stage, the supplier decides on his direct price and wholesale price. With $\partial^{2} {\uppi }_{{\text{s}}} /\partial (d^{TN} )^{2} < 0$ and $\partial^{2} {\uppi }_{{\text{s}}} /\partial (w^{TN} )^{2} < 0$, $\partial {\uppi }_{{\text{s}}} /\partial d^{TN} = 0$ and $\partial {\uppi }_{{\text{s}}} /\partial w^{TN} = 0$, we can derive expressions of the optimal direct price and wholesale price in relation to the creditor’s interest rate are $w^{TN} = \frac{{1 + a\left( { - 1 + \delta } \right)}}{{2\left( {1 - \delta^{2} } \right)}} - \frac{{c\left[ { - 1 + I_{TN} \left( { - 2 + \delta } \right)} \right]}}{2},d^{TN} = \frac{{a\left( { - 1 + \delta } \right) - \delta }}{{2\left( { - 1 + \delta^{2} } \right)}} + \frac{{c\left( {1 + I_{TN} } \right)}}{2}$. In first stage, the retailer as a creditor sets interest rate. With $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {I_{TN} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{r}}} /\partial I_{TN} = 0$, we solve the optimal interest rate $I_{TN} = \frac{{\delta + a\left( {1 - \delta } \right)}}{{2c\left( {1 - \delta } \right)\left( {1 + \delta } \right)}} - \frac{1}{2}$. Then taking $I_{TN}$ to $w^{TN}$ and $d^{TN}$, we can get the optimal wholesale price $w^{TN} = \frac{{2 + \left( {2 - a} \right)\delta - \left( {1 - a} \right)\delta^{2} }}{{4\left( {1 - \delta^{2} } \right)}} + \frac{c\delta }{4}$ and direct price $d^{TN} = \frac{{3\left[ {a\left( {1 - \delta } \right) + \delta } \right]}}{{4\left( {1 - \delta^{2} } \right)}} + \frac{c}{4}$. Next, we take $w^{TN}$, $d^{TN}$ and $I_{TN}$ to $p^{TN}$, we can get the optimal retail price $p^{TN} = \frac{{3\left[ {1 - a\left( {1 - \delta } \right)} \right]}}{{4\left( {1 - \delta^{2} } \right)}} + \frac{c}{4}$. Finally, we derive the participates’ profits as $\pi_{s}^{TN} = \pi_{s}^{NN} - \frac{{3c^{2} \left( {1 - \delta^{2} } \right)}}{4}I_{TN}^{2}$ and $\pi_{r}^{TN} = \pi_{r}^{NN} + \frac{{c^{2} \left( {1 - \delta^{2} } \right)}}{2}I_{TN}^{2}$.

1.5 Proof of Proposition 4

Under TCF scenario and retailer encroaches the online market. In this case, the retailer’s profit is as $\pi_{r} = \left( {p - w} \right)D_{r}^{{\text{E}}} + \left( {b - w} \right)Q_{r}^{{\text{E}}} + cI_{{{\text{TE}}}} \left( {D_{s}^{{\text{E}}} + D_{r}^{{\text{E}}} + Q_{r}^{{\text{E}}} } \right)$. In third stage, the retailer decides on her retail price and booking price. With $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {p^{TE} } \right)^{2} < 0$, $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {b^{TE} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{r}}} /\partial p^{TE} = 0$, $\partial {\uppi }_{{\text{r}}} /\partial b^{TE} = 0$. We can derive expression of the optimal retail price $p^{TE} = \frac{{4\left( {1 + w} \right) + a\left( { - 4 + \delta } \right) + 2d\delta + d\delta^{2} - w\delta^{2} + 2cI_{TE} \left( { - 2 + \delta + \delta^{2} } \right)}}{{2\left( {4 - \delta^{2} } \right)}}$ and booking price $b^{TE} = \frac{{4w - 2a\left( { - 1 + \delta } \right) + 2\delta + 2d\delta + d\delta^{2} - w\delta^{2} + 2cI_{TE} \left( { - 2 + \delta + \delta^{2} } \right)}}{{2\left( {4 - \delta^{2} } \right)}}$ in relation to the supplier’s direct price, wholesale price and interest rate. In second stage, the supplier decides on his direct price and wholesale price. With $\partial^{2} {\uppi }_{{\text{s}}} /\partial (d^{TE} )^{2} < 0$ and $\partial^{2} {\uppi }_{{\text{s}}} /\partial (w^{TE} )^{2} < 0$, $\partial {\uppi }_{{\text{s}}} /\partial d^{TE} = 0$ and $\partial {\uppi }_{{\text{s}}} /\partial w^{TE} = 0$, we can derive expressions of the optimal direct price and wholesale price in relation to the creditor’s interest rate are $w^{TE} = \frac{{4 - 2\delta - a\left( {2 - 3\delta + \delta^{2} } \right)}}{{4\left( { - 2 + \delta } \right)\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{{cI_{TE} \left( {4 - 3\delta } \right)}}{{2\left( {2 - \delta } \right)}} + \frac{c}{2},d^{TE} = \frac{{a\left( { - 1 + \delta } \right) - \delta }}{{2\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{{c\left( {1 + I_{TE} } \right)}}{2}$. In first stage, the retailer as a creditor sets interest rate. With $\partial^{2} {\uppi }_{{\text{r}}} /\partial \left( {I_{TE} } \right)^{2} < 0$ and $\partial {\uppi }_{{\text{r}}} /\partial I_{TE} = 0$, we solve the optimal interest rate $I_{TE} = \frac{{\delta + a\left( {1 - \delta } \right)}}{{2c\left( {1 - \delta } \right)\left( {2 + \delta } \right)}} - \frac{1}{2}$. Then taking $I_{TE}$ to $w^{TE}$ and $d^{TE}$, we can get the optimal wholesale price $w^{TE} = \frac{{4 + 2a\left( { - 1 + \delta } \right)^{2} + 2\left( {1 + c} \right)\delta - \left( {3 + c} \right)\delta^{2} - c\delta^{3} }}{{4\left( { - 2 + \delta } \right)\left( { - 2 + \delta + \delta^{2} } \right)}}$ and direct price $d^{TE} = \frac{{3\left[ {a\left( { - 1 + \delta } \right) - \delta } \right]}}{{4\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{c}{4}$. Next, we take $w^{TE}$, $d^{TE}$ and $I_{TE}$ to $p^{TE}$, we get the optimal retail price $p^{TE} = \frac{{ - 10 - 9a\left( { - 1 + \delta } \right) + 4\delta }}{{8\left( { - 2 + \delta + \delta^{2} } \right)}} + \frac{c}{4}$. Finally, we derive the participates’ profits as $\pi_{s}^{{{\text{TE}}}} = \pi_{s}^{{{\text{NE}}}} - \frac{{3c^{2} \left( {1 - \delta } \right)\left( {2 + \delta } \right)}}{{4\left( {2 - \delta } \right)}}I_{{{\text{TE}}}}^{2}$ and $\pi_{r}^{{{\text{TE}}}} = \pi_{r}^{{{\text{NE}}}} + \frac{{c^{2} \left( {1 - \delta } \right)\left( {2 + \delta } \right)}}{{2\left( {2 - \delta } \right)}}I_{{{\text{TE}}}}^{2}$.

1.6 Proof of Lemma 2

When $\pi_{r}^{TE} - \pi_{r}^{TN} > 0$, the retailer will encroach the online market, otherwise, she doesn’t encroach. $\pi_{r}^{TE} - \pi_{r}^{TN} = \frac{1}{{64\left[ {\left( {2 -\delta } \right)\left( {1 - \delta } \right)\left( {1 + \delta }\right)\left( {2 + \delta } \right)} \right]}}\left\{ {24 - 72a -12\delta - } \right\}$$4a\delta - 44\delta^{2} + 128a\delta^{2} + 20\delta^{3} - 44a\delta^{3} + 4\delta^{4} - 8a\delta^{4} + 4c^{2} \left( { - 1 + \delta } \right)^{2} \left( {4 + 4\delta + \delta^{2} + 2\delta^{3} + \delta^{4} } \right) + a^{2} \left( {34 + 21\delta - 86\delta^{2} + 27\delta^{3} + 4\delta^{4} } \right)$$+ c\left[ { - 8a\left( { - 1 + \delta } \right)^{3}\left( {2 + 3\delta + \delta^{2} } \right) + 8\left( { - 1 + \delta } \right)^{2} \delta \left( {2 + 3\delta + \delta^{2} } \right)}\right].$ As evidenced by Lemma 1, the equilibrium strategy is as summarized in the text.

1.7 Proof of Lemma 3

According to the retailer behavior equilibrium, we discuss how the supplier considers different credit financing strategies. Through the similar steps as before, we divide the range of $\delta ,c$ and $a$ into the following intervals

• 1. consider the substitution degree $\delta \in \left[ {0,} \right.\left. {0.319} \right)$, (i) if the production cost $c \in \left[ 0 \right.,\left. {c_{3} } \right)$ and potential online market $a \in \left[ 0 \right.,\left. {\overline{a}} \right)$ or production cost $c \in \left[ {c_{3} ,} \right.c_{1} )$ and potential online market $a \in \left[ {a_{1} } \right.,\left. {\overline{a}} \right)$ or $a \in \left[ {a_{2} } \right.,\left. {\overline{a}} \right)$ or $c \in \left[ {c_{1} ,} \right.\overline{c})$ and $a \in \left[ {a_{2} } \right.,\left. {\overline{a}} \right)$, the supplier will choose trade credit financing strategy; (ii) if the production cost $c \in \left[ {c_{3} ,} \right.\left. {c_{1} } \right)$ and potential online market $a \in {\text{min}}\left\{ {a_{1} ,a_{2} } \right\}$ or $c \in \left[ {c_{1} ,} \right.\overline{c})$ and $a \in {\text{min}}\left\{ {\overline{a},a_{2} } \right\}$ the supplier will choose bank credit financing strategy.

• 2. consider the substitution degree $\delta \in [\left. {0.319,0.505} \right)$, (i) if the production cost $c \in \left[ 0 \right.,\left. {c_{3} } \right)$ and potential online market $a \in \left[ 0 \right.,\left. {\overline{a}} \right)$, the supplier will choose trade credit financing strategy; (ii) if $c \in \left[ {c_{3} ,} \right.\overline{c})$ and potential online market $a \in {\text{min}}\left\{ {a_{1} ,a_{2} } \right\}$, the supplier will choose bank credit financing strategy, otherwise, he will choose trade credit financing strategy.

• 3. consider the substitution degree $\delta \in [\left. {0.505,1} \right)$, if $c \in \left[ {0,} \right.\overline{c})$ and $a \in \left[ 0 \right.,\left. {\overline{a}} \right)$, the supplier will choose trade credit financing.