Skip to main content

Advertisement

Springer Nature Link
Log in

Hopf Bifurcation of a Modified Leslie--Gower Predator--Prey System

  • Published:
Cognitive Computation Aims and scope Submit manuscript

Abstract

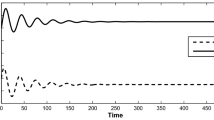
In this paper, Hopf bifurcation is studied for a modified Leslie--Gower predator--prey system with harvesting. Our modified system is a biological economic cognitive system. An important feature of the biological economic cognitive system is that the harvest effort on the predator--prey system is investigated from an economic perspective. By using the local parameterization method and Hopf bifurcation theory, we obtain some new criteria for the Hopf bifurcation. Lastly, a numerical example is provided to demonstrate the effectiveness of our results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Cai LM, Song XY. Permanence and stability of a predator-prey system with stage structure for predator. J Comput Appl Math. 2007;201(2):356–66.

    Article  Google Scholar 

  2. Chen BS, Liao XX, Liu YQ. Normal forms and bifurcations for the differential-algebraic systems. Acta Math Appl Sin. 2000;23:429–43 (in Chinese).

    Google Scholar 

  3. Gordon HS. Economic theory of a common property resource: the fishery. J Polit Econ. 1954; 62(2):124–42.

    Article  Google Scholar 

  4. Gurney KN. Reverse engineering the vertebrate brain: methodological principles for a biologically grounded programme of cognitive modelling. Cogn Comput. 2009;1:29–41.

    Article  Google Scholar 

  5. Huang XC. Stability of a general predator-prey model. J Franklin Inst. 1990;327:751–69.

    Article  Google Scholar 

  6. Kar TK, Pahari UK. Non-selective harvesting in prey-predator models with delay. Commun Nonlinear Sci Numer Simulat. 2006;11(4):499–509.

    Article  Google Scholar 

  7. Li ZH, Zhao KH, Li YK. Multiple positive periodic solutions for a non-autonomous stage-structured predatory-prey system with harvesting terms. Commun Nonlinear Sci Numer Simulat. 2010;15(8):2140–48.

    Article  Google Scholar 

  8. Liu C, Zhang QL, Duan XD. Dynamical behavior in a harvested differential-algebraic prey-predator model with discrete time delay and stage structure. J Franklin Inst. 2009;346:1038–59.

    Article  Google Scholar 

  9. Liu C, Zhang QL, Zhang Y, Duan XD (2008) Bifurcation and control in a differential-algebraic harvested prey-predator model with stage structure for predator. Internat J Bifur Chaos. 18(10): 3159–168.

    Article  Google Scholar 

  10. Lucas WF (1983) Modules in applied mathematics: differential equation models. Springer, New York.

    Google Scholar 

  11. Qu Y, Wei JJ. Bifurcation analysis in a predator-prey system with stage-structure and harvesting. J Franklin Inst. 2010;347:1097–113.

    Article  Google Scholar 

  12. Tao YD, Wang X, Song XY (2011) Effect of prey refuge on a harvested predator-prey model with generalized functional response. Commun Nonlinear Sci Numer Simulat. 2009;16(2):1052–59.

    Article  Google Scholar 

  13. Zhang X, Zhang QL. Bifurcation analysis and control of a class of hybrid biological economic models. Nonlinear Anal HS. 2009;3:578–87.

    Article  Google Scholar 

  14. Zhang X, Zhang QL, Zhang Y. Bifurcations of a class of singular biological economic models. Chaos Solitons Fractals. 2009;40:1309–318.

    Article  Google Scholar 

  15. Zhang Y, Zhang QL, Zhao LC. Bifurcations and control in singular biological economical model with stage structure. J Syst Eng. 2007;22(3):232–38.

    Google Scholar 

Download references

Acknowledgments

The authors are greatly indebted to the anonymous reviewers and the editor for their insightful comments. The work is supported by Key Science Foundation of Educational Department of Hubei Province under Grant D20082201.

Author information

Authors and Affiliations

  1. School of Mathematics and Computer Science, Xinyu University, Xinyu, 338004, People’s Republic of China

    Wei Liu

  2. College of Mathematics and Statistics, Hubei Normal University, Huangshi, 435002, People’s Republic of China

    Chaojin Fu

Authors
  1. Wei Liu
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Chaojin Fu
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding authors

Correspondence to Wei Liu or Chaojin Fu.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Liu, W., Fu, C. Hopf Bifurcation of a Modified Leslie--Gower Predator--Prey System. Cogn Comput 5, 40–47 (2013). https://doi.org/10.1007/s12559-012-9162-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12559-012-9162-3

Keywords