Skip to main content
SpringerLink
Log in

Bifurcation−Driven Tipping in A Novel Bicyclic Crossed Neural Network with Multiple Time Delays

  • Published:
Cognitive Computation Aims and scope Submit manuscript

Abstract

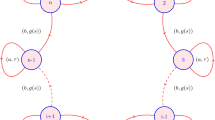
Anatomical experiments have proved that a large number of ring structures exist in neural networks. Therefore, many scholars have focused on modeling and dynamic analysis of multi-ring neural networks. However, most current research regarding multi-ring neural networks considers the case that rings share only one neuron with each other. This type of connection among rings fails to adequately capture the complex structure of actual neural networks. In this paper, we consider two sharing neurons between rings and propose a bicyclic crossed neural network model with multiple time delays. Then, the stability condition of the proposed neural network model is given without time delays. By choosing the sum of time delays as the bifurcation parameter, the occurrence conditions of tipping due to Hopf bifurcation are derived, and the tipping point (bifurcation threshold) is accurately determined. In addition, the explicit formulae for ascertaining the Hopf bifurcation properties are given by utilizing the center manifold theory, which further reveals the evolutionary tipping mechanism. Finally, through numerical simulations, the validity of the theoretical analysis is verified. The results show that with the increase in time delays, the network will gradually lose stability, and the tipping driven by Hopf bifurcation will occur. Moreover, the magnitude of time delays emerges as a significant determinant of both the amplitude and the oscillation period of unstable neurons.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data Availability

The data that support the findings of this study are available from the corresponding author, [MX], upon reasonable request.

References

  1. Briot JP. From artificial neural networks to deep learning for music generation: history, concepts and trends. Neural Comput & Appl. 2021;33:39–65.

    Article  Google Scholar 

  2. Bao H, Chen Z, Cai J, et al. Memristive cyclic three-neuron-based neural network with chaos and global coexisting attractors. Sci China Tech Sci. 2022;65:2582–92.

    Article  Google Scholar 

  3. Nath S. Minimal model for oscillatory dynamics of a nonlinear chemical reaction network. Physica D. 2022;441: 133503.

    Article  MathSciNet  Google Scholar 

  4. Hou Q, Wang Z, Tan F, et al. RISTDnet: Robust infrared small target detection network. IEEE Geosci Remote S. 2021;19:1–5.

    Article  Google Scholar 

  5. Lin H, Wu C, Masdari M. A comprehensive survey of network traffic anomalies and DDoS attacks detection schemes using fuzzy techniques. Comput Electr Eng. 2022;104: 108466.

    Article  Google Scholar 

  6. Abdou MA. Literature review: Efficient deep neural networks techniques for medical image analysis. Neural Comput & Appl. 2022;34:5791–812.

    Article  Google Scholar 

  7. Dabbu M, Karuppusamy L, Pulugu D, et al. Water atom search algorithm-based deep recurrent neural network for the big data classification based on spark architecture. Int J Mach Learn & Cyber. 2022;13:2297–312.

    Article  Google Scholar 

  8. Liu N, Ya Z, Tong X, et al. Meshless surface wind speed field reconstruction based on machine learning. Adv Atmos Sci. 2022;39:1721–33.

    Article  Google Scholar 

  9. Zheng Q, Shen J. Turing instability induced by random network in FitzHugh-Nagumo model. Applied Mathematics and Computation. 2020;381: 125304.

    Article  MathSciNet  Google Scholar 

  10. Zhou W, Fu B, Wang G. Time-delay memristive recurrent neural network and its complex dynamics. Int J Bifurc Chaos. 2022;32:2250150.

    Article  MathSciNet  Google Scholar 

  11. Zhang T, Zhou J, Liao Y. Exponentially stable periodic oscillation and Mittag-Leffler stabilization for fractional-order impulsive control neural networks with piecewise Caputo derivatives. IEEE Trans Cybern. 2021;52:9670–83.

    Article  Google Scholar 

  12. Guo Y, Ge SS, Arbi A. Stability of traveling waves solutions for nonlinear cellular neural networks with distributed delays. J Syst Sci Complex. 2022;35:18–31.

    Article  MathSciNet  Google Scholar 

  13. Xu C, Mu D, Liu Z, et al. New insight into bifurcation of fractional-order 4D neural networks incorporating two different time delays. Commun Nonlinear Sci Numer Simul. 2023;118: 107043.

    Article  MathSciNet  Google Scholar 

  14. Lu L, Yang L, Zhan X, et al. Cluster synchronization and firing rate oscillation induced by time delay in random network of adaptive exponential integrate-and-fire neural system. Eur Phys J B. 2020;93:1–9.

    Article  Google Scholar 

  15. Wei JJ, Ruan SH. Stability and bifurcation in a neural network model with two delays. Physica D. 1999;130:255–72.

    Article  MathSciNet  Google Scholar 

  16. Yu W, Cao J. Stability and Hopf bifurcation analysis on a four-neuron BAM neural network with time delays. Phys Lett A. 2006;351:64–78.

    Article  Google Scholar 

  17. Lin H, Wang C, Tan Y. Hidden extreme multistability with hyperchaos and transient chaos in a Hopfield neural network affected by electromagnetic radiation. Nonlinear Dyn. 2020;99:2369–86.

    Article  Google Scholar 

  18. Tabekoueng NZ, Kengne J, Fotsin HB. Coexistence of multiple stable states and bursting oscillations in a 4D Hopfield neural network. Circuits Syst Signal Process. 2020;39:3424–44.

    Article  Google Scholar 

  19. Fields RD, Stevens-Graham B. New insights into neuron-glia communication. Science. 2002;298:556–62.

    Article  Google Scholar 

  20. Yu D, Zhou X, Wang G, et al. Effects of chaotic activity and time delay on signal transmission in FitzHugh-Nagumo neuronal system. Cogn Neurodyn. 2022;16:887–97.

    Article  Google Scholar 

  21. Lu Y, Xiao M, He J, et al. Stability and bifurcation exploration of delayed neural networks with radial-ring configuration and bidirectional coupling. IEEE Trans Neural Netw Learn Syst. 2023.

  22. Alimi AM, Aouiti C, Assali EA. Finite-time and fixed-time synchronization of a class of inertial neural networks with multi-proportional delays and its application to secure communication. Neurocomputing. 2019;332:29–43.

    Article  Google Scholar 

  23. Vaishwar A, Yadav BK. Stability and Hopf-bifurcation analysis of four dimensional minimal neural network model with multiple time delays. Chinese J Phys. 2022;77:300–18.

    Article  MathSciNet  Google Scholar 

  24. Zhang H, Cheng J, Zhang H, et al. Quasi-uniform synchronization of Caputo type fractional neural networks with leakage and discrete delays. Chaos Solitons Fractals. 2021;152: 111432.

    Article  MathSciNet  Google Scholar 

  25. Chen S. Traveling wave solutions of diffusive Hindmarsh-Rose-type equations with recurrent neural feedback. J Math Anal Appl. 2021;493: 124513.

    Article  MathSciNet  Google Scholar 

  26. Xu C, Liu Z, Liao M, et al. Fractional-order bidirectional associate memory (BAM) neural networks with multiple delays: The case of Hopf bifurcation. Math Comput Simulat. 2021;182:471–94.

    Article  MathSciNet  Google Scholar 

  27. Wang T, Wang Y, Cheng Z. Stability and Hopf Bifurcation Analysis of a General Tri-diagonal BAM Neural Network with Delays. Neural Process Lett. 2021;53:4571–92.

    Article  Google Scholar 

  28. Zhou S, Xiao M, Wang L, et al. Bifurcation and oscillations of a multi-ring coupling neural network with discrete delays. Cogn Comput. 2021;13:1233–45.

    Article  Google Scholar 

  29. Misir S, Wu N, Yang BB. Specific expression and functions of circular RNAs. Cell Death Differ. 2022;29:481–91.

    Article  Google Scholar 

  30. Herranz M, Pardos F, Boyle MJ. Comparative morphology of serotonergic-like immunoreactive elements in the central nervous system of kinorhynchs kinorhyncha, cyclorhagida. J Morphol. 2013;274:258–74.

    Article  Google Scholar 

  31. Hu H, Huang L. Stability and Hopf bifurcation analysis on a ring of four neurons with delays. Appl Math Comput. 2009;213:587–99.

    MathSciNet  Google Scholar 

  32. Tao B, Xiao M, Zheng WX, et al. Dynamics analysis and design for a bidirectional super-ring-shaped neural network with n neurons and multiple delays. IEEE Trans Neural Netw Learn Syst. 2021;32:2978–92.

    Article  MathSciNet  Google Scholar 

  33. Xing R, Xiao M, Zhang Y, et al. Stability and Hopf bifurcation analysis of an (n+m)-neuron double-ring neural network model with multiple time delays. J Syst Sci Complex. 2022;35:159–78.

    Article  MathSciNet  Google Scholar 

  34. Cheng Z, Xie K, Wang T, et al. Stability and Hopf bifurcation of three-triangle neural networks with delays. Neurocomputing. 2018;322:206–15.

    Article  Google Scholar 

  35. Zhang Y, Xiao M, Cao J, et al. Dynamical bifurcation of large-scale-delayed fractional-order neural networks with hub structure and multiple rings. IEEE Trans Syst Man Cybern Syst. 2022;52:1731–43.

    Article  Google Scholar 

  36. Gao Y, Chen Y, Xie Y, et al. A class of topological nodal rings and its realization in carbon networks. Phys Rev B. 2018;97: 121108.

    Article  Google Scholar 

  37. Zhang X, Tu H, Guo J, et al. Braess paradox and double-loop optimization method to enhance power grid resilience. Reliab Eng Syst Saf. 2021;215: 107913.

    Article  Google Scholar 

  38. Kaszs B, Feudel U, Tl T. Tipping phenomena in typical dynamical systems subjected to parameter drift. Sci Rep. 2019;9:1–12.

    Google Scholar 

  39. Ehret S, Constantino SM, Weber EU, et al. Group identities can undermine social tipping after intervention. Nat Hum Behav. 2022;6:1669–79.

    Article  Google Scholar 

  40. Liu T, Chen D, Yang L, et al. Teleconnections among tipping elements in the Earth system. Nat Clim Chang. 2023;13:67–74.

    Article  Google Scholar 

  41. Carrier B, Pascal L, Nozais C, et al. Tipping points and multiple drivers in changing aquatic ecosystems: A review of experimental studies. Limnol Oceanogr. 2022;67:312–30.

    Google Scholar 

  42. Jules S, Sylvain B, Patrice G, et al. Can the threat of economic sanctions ensure the sustainability of international fisheries an experiment of a dynamic non-cooperative CPR game with uncertain tipping point. Environ Resource Econ. 2020;76:153–76.

    Article  Google Scholar 

  43. Zhang X, Xu Y, Liu Q, et al. Rate-dependent tipping-delay phenomenon in a thermoacoustic system with colored noise. Sci China Technol Sci. 2020;63:2315–27.

    Article  Google Scholar 

  44. Xiao M, Chen S, Zheng WX, et al. Tipping point prediction and mechanism analysis of malware spreading in cyber-physical systems. Commun Nonlinear Sci Numer Simul. 2023;122: 107247.

    Article  MathSciNet  Google Scholar 

  45. Lu Y, Xiao M, Huang C, et al. Early Warning of Tipping in a Chemical Model with Cross-Diffusion via Spatiotemporal Pattern Formation and Transition. Chaos. 2023;33: 073120.

    Article  MathSciNet  Google Scholar 

  46. He H, Xiao M, Lu Y, et al. Control of tipping in a small-world network model via a novel dynamic delayed feedback scheme. Chaos, Solitons and Fractals. 2023;168: 113171.

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work is supported in part by the National Natural Science Foundation of China under Grant 62073172 and the Natural Science Foundation of Jiangsu Province of China under Grant BK20221329.

Author information

Authors and Affiliations

  1. College of Automation and College of Artificial Intelligence, Nanjing University of Posts and Telecommunications, Nanjing, 210023, China

    Xiangyu Du, Min Xiao, Jie Ding & Jiajin He

  2. School of Mathematical Sciences, Nanjing Normal University, Nanjing, 210023, China

    Yi Yao

  3. School of Mathematics, Southeast University, Nanjing, 210096, China

    Jinde Cao

  4. Yonsei Frontier Lab, Yonsei University, Seoul, 03722, South Korea

    Jinde Cao

Authors
  1. Xiangyu Du
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Min Xiao
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jie Ding
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jiajin He
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Yi Yao
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Jinde Cao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Min Xiao.

Ethics declarations

Ethical Approval

This article does not contain any experiments with human or animal participants performed by any of the authors.

Informed Consent

Informed consent was obtained from all individual participants included in the study.

Conflict of Interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Du, X., Xiao, M., Ding, J. et al. Bifurcation−Driven Tipping in A Novel Bicyclic Crossed Neural Network with Multiple Time Delays. Cogn Comput 16, 278–292 (2024). https://doi.org/10.1007/s12559-023-10199-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12559-023-10199-4

Keywords

Search

Navigation