Skip to main content

Advertisement

Springer Nature Link
Log in

Optimal robust disturbance observer based sliding mode controller using multi-objective grasshopper optimization algorithm to enhance power system stability

  • Original Research
  • Published:
Journal of Ambient Intelligence and Humanized Computing Aims and scope Submit manuscript

Abstract

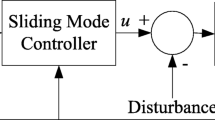
Power system stabilizer (PSS) has operated as an auxiliary controller for the synchronous generator excitation system to suppress the power system low frequency oscillations. This paper aims to design an optimal robust disturbance observer based sliding mode controller (RDO-SMC) acting as PSS to enhance the power system dynamic stability through the nonlinear control of excitation system. Indeed, the sliding disturbance observer has expeditiously estimated the accumulated consequence of unknown nonlinearities, parameter uncertainties, non-ideal dynamic models and exterior time-varying interferences in support of sliding mode controller. The sliding surface trajectory has been mathematically derived using Lyapunov theory. RDO-SMC has investigated the low frequency suppression of both the terminal voltage and angular speed. Due to multi-objective nature of the designed control problem, multi-objective grasshopper optimization algorithm (MOGOA) has been implemented to optimally tune the parameters of RDO-SMC and other understudy controllers. To verify and validate the performance of proposed controller, the analyses have been performed in single-machine infinite-bus and multi-machine power systems under different disturbance conditions. At last, the comprehensive simulation results have certainly confirmed the dynamic capability of the optimal RPO-SMC.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

Explore related subjects

Discover the latest articles and news from researchers in related subjects, suggested using machine learning.

References

  • Ali Khan Z, Zafar A, Javaid S, Aslam S, Rahim MH, Javaid N (2019) Hybrid meta-heuristic optimization based home energy management system in smart grid. J Ambient Intell Humaniz Comput 10(12):4837–4853

    Google Scholar 

  • Bacon M, Olgac N, Cepeda-Gomez R (2012) Robust region tracking for swarms via a novel utilization of sliding mode control. Intell Control Autom 3(1):98–109

    Google Scholar 

  • Basler MJ, Schaefer RC (2005) Understanding power system stability. In: 58th Annual Conference for Protective Relay Engineers, IEEE, College Station, TX, USA, pp 46–67. https://doi.org/10.1109/CPRE.2005.1430421

    Chapter  Google Scholar 

  • Coello CA (2009) Evolutionary multi-objective optimization: some current research trends and topics that remain to be explored. Front Comput Sci China 3(1):18–30

    Google Scholar 

  • Dash PK, Patnaik RK, Mishra SP (2018) Adaptive fractional integral terminal sliding mode power control of UPFC in DFIG wind farm penetrated multi-machine power system. Prot Control Mod Power Syst 3(8):1–14

    Google Scholar 

  • Falehi AD (2013) Design and scrutiny of maiden pss for alleviation of power system oscillations using RCGA and PSO techniques. J Electr Eng Technol 8(3):402–410

    Google Scholar 

  • Falehi AD (2018a) Optimal design of fractional order ANFIS-PSS based on NSGA-II aimed at mitigation of DG-connection transient impacts. Proc Roman Acad Ser A 19(3):473–481

    MathSciNet  Google Scholar 

  • Falehi AD (2018b) MOBA based design of FOPID–SSSC for load frequency control of interconnected multi-area power systems. Smart Struct Syst 22(1):81–94

    Google Scholar 

  • Falehi AD (2019a) An innovative OANF–IPFC based on MOGWO to enhance participation of DFIG-based wind turbine in interconnected reconstructed power system. Soft Comput 23(4):12911–12927

    Google Scholar 

  • Falehi AD (2019b) Optimal fractional order BELBIC to ameliorate small signal stability of interconnected hybrid power system. Environ Progress Sustain Energy 38(5):1–18

    Google Scholar 

  • Falehi AD, Mosallanejad A (2016) Neoteric HANFISC-SSSC based on MOPSO technique aimed at oscillation suppression of interconnected multi-source power systems. IET Gener Transm Distrib 10(7):1728–1740

    Google Scholar 

  • Falehi AD, Rafiee M (2019) Optimal control of novel fuel cell-based DVR using ANFISC-MOSSA to increase FRT capability of DFIG-wind turbine. Soft Comput 23(15):6633–6655

    Google Scholar 

  • Falehi AD, Rostami M (2011a) A robust approach based on RCGA-optimization technique to enhance power system stability by coordinated design of PSS and AVR. Int Rev Electr Eng 6(1):371–378

    Google Scholar 

  • Falehi AD, Rostami M (2011b) Design and analysis of a novel dual-input PSS for damping of power system oscillations employing RCGA-optimization technique. Int Rev Electr Eng 6(2):938–945

    Google Scholar 

  • Falehi AD, Dankoob A, Amirkhan S (2011) Coordinated design of STATCOM-based damping controller and dual-input PSS to improve transient stability of power system. Int Rev Electr Eng 6(3):1308–1318

    Google Scholar 

  • Fombu AM, Kenné G, Nguimfack-Ndongmo JD, Kuate-Fochie R (2016) Decentralized nonlinear coordinated excitation and steam valve adaptive control for multi-machine power systems. Electr Power Energy Syst 75(1):117–126

    Google Scholar 

  • Gibbard MJ (1988) Coordinated design of multi-machine power system stabilizers based on damping torque concepts. IEE Proc C Gener Transm Distrib 135(4):276–284

    Google Scholar 

  • Ginoya D, Shendge PD, Phadke SB (2014) Sliding mode control for mismatched uncertain systems using an extended disturbance observer. IEEE Trans Ind Electron 61(4):1983–1992

    Google Scholar 

  • Golshannavaz S, Khezri R, Esmaeeli M, Siano P (2018) A two-stage robust-intelligent controller design for efficient LFC based on Kharitonov theorem and fuzzy logic. J Ambient Intell Humaniz Comput 9(5):1445–1454

    Google Scholar 

  • Guan J, Lin G (2016) Hybridizing variable neighborhood search with ant colony optimization for solving the single row facility layout problem. Eur J Oper Res 248(3):899–909

    MathSciNet  MATH  Google Scholar 

  • Gupta R, Bandyopadhyay B, Kulkarni AM (2003) Design of power system stabilizer for single machine system using robust fast output sampling feedback technique. Electric Power Syst Res 65(3):247–257

    Google Scholar 

  • Hardiansyah SF, Irisawa J (2006) A robust H∞ power system stabilizer design using reduced-order models. Int J Electr Power Energy Syst 28(1):21–28

    Google Scholar 

  • Hemmati R, Boroujeni SMS, Abdollahi M (2010) Comparison of robust and intelligent based power system stabilizers. Int J Phys Sci 5(17):2564–2573

    Google Scholar 

  • Jiang L, Wu QH, Wen JY (2002) Nonlinear adaptive control via sliding-mode state and disturbance observer. IEE Proc Control Theory Appl 149(4):269–277

    Google Scholar 

  • Kenné G, Fombu AM, Nguimfack-Ndongmo JD (2016) Coordinated excitation and steam valve control for multimachine power system using high order sliding mode technique. Electr Power Syst Res 131(1):87–95

    Google Scholar 

  • Khodabakhshian A, Hemmati R (2012) Robust decentralized multi-machine power system stabilizer design using quantitative feedback theory. Int J Electr Power Energy Syst 41(1):112–119

    Google Scholar 

  • Kumar A (2016) Power system stabilizers design for multimachine power systems using local measurements’. IEEE Trans Power Syst 31(3):2163–2171

    MathSciNet  Google Scholar 

  • Kundur P, Klein M, Rogers GJ, Zywno MS (1989) Application of power system stabilizers for enhancement of overall system stability. IEEE Trans Power Syst 4(2):614–626

    Google Scholar 

  • Larsen EV, Swann DA (1981a) Applying power system stabilizers part III: practical considerations. IEEE Trans Power Appar Syst 100(6):3034–3046

    Google Scholar 

  • Larsen EV, Swann DA (1981b) Applying power system stabilizers part I: general concepts. IEEE Trans Power Appar Syst 100(6):3017–3024

    Google Scholar 

  • Li X, Zhou J (2017) A sliding mode control design for mismatched uncertain systems based on states transformation scheme and chattering alleviating scheme. Trans Inst Meas Control 40(8):2509–2516

    Google Scholar 

  • Liua X, Han Y (2016) Decentralized multi-machine power system excitation control using continuous higher-order sliding mode technique. Int J Electr Power Energy Syst 82(11):76–86

    Google Scholar 

  • Mafarjaa M, Aljarah I, Heidari AA, Hammouri AI, Faris H, Al-Zoubi AM, Mirjalili SA (2018) Evolutionary population dynamics and grasshopper optimization approaches for feature selection problems. Knowl Based Syst 145(7):25–45

    Google Scholar 

  • Marco FJ, Martins N, Ferraz JCR (2013) An automatic method for power system stabilizers phase compensation design. IEEE Trans Power Syst 28(2):997–1007

    Google Scholar 

  • Mohanty B (2019) Hybrid flower pollination and pattern search algorithm optimized sliding mode controller for deregulated AGC system. J Ambient Intell Humaniz Comput 11(2):763–776

    Google Scholar 

  • Nechadi E, Harmas MN, Hamzaoui A, Essounbouli N (2012) A new robust adaptive fuzzy sliding mode power system stabilizer. Int J Electr Power Energy Syst 42(1):1–7

    Google Scholar 

  • Rajesh KS, Dash SS (2019) Load frequency control of autonomous power system using adaptive fuzzy based PID controller optimized on improved sine cosine algorithm. J Ambient Intell Humaniz Comput 10(6):2361–2373

    Google Scholar 

  • Ray PK, Paital SR, Mohanty A, Eddy FYS, Gooi HB (2018) A robust power system stabilizer for enhancement of stability in power system using adaptive fuzzy sliding mode control. Appl Soft Comput 73(12):471–481

    Google Scholar 

  • Ricard OV, Rodrigo Ramos A, Newton GB (2010) An algorithm for computerized automatic tuning of power system stabilizers. Control Eng Pract 18(1):45–54

    Google Scholar 

  • Saremi S, Mirjalili SA, Lewis A (2017) Grasshopper optimization algorithm: theory and application. Adv Eng Softw 105(3):30–47

    Google Scholar 

  • Talbi E (2009) Metaheuristics from design to implementation, Wiley Online Library. https://doi.org/10.1002/9780470496916

  • vonLucken C, Baran B, Brizuela C (2014) A survey on multi-objective evolutionary algorithms for many-objective problems. Comput Optim Appl 58(3):707–756

    MathSciNet  MATH  Google Scholar 

  • Wang H, Yang S, Ip W, Wang D (2010) A particle swarm optimization based Memetic algorithm for dynamic optimization problems’. Nat Comput 9(3):703–725

    MathSciNet  MATH  Google Scholar 

  • Xie Y, Zhang H, Li C, Sun H (2017) Development approach of a programmable and open software package for power system frequency response calculation. Prot Control Mod Power Syst 2(18):1–10

    Google Scholar 

  • Yang T (1997) Applying H∞ optimisation method to power system stabiliser design part 1: single-machine infinite-bus systems. Int J Electr Power Energy Syst 19(1):29–35

    MathSciNet  Google Scholar 

Download references

Funding

There was no funding source for this study.

Author information

Authors and Affiliations

  1. Department of Electrical Engineering, Shadegan Branch, Islamic Azad University, Shadegan, Iran

    Ali Darvish Falehi

Authors
  1. Ali Darvish Falehi
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Ali Darvish Falehi.

Ethics declarations

Conflict of interest

Author declares that he has no conflict of interest.

Ethical approval

This article does not contain any studies with human participants or animals performed by any of the authors.

Informed consent

Informed consent was obtained from all individual participants included in the study.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

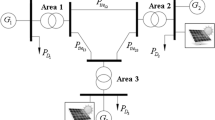
1.1 Single-machine infinite-bus power system

Generator: SB = 2100MVA, H = 3.7 s, VB = 13.8 kV, RS = 2.8544e−3, f = 60 Hz, \( X_{d} \) = 1.305 p.u.,\( X^{\prime}_{d} \) = 0.296 p.u \( X^{\prime\prime}_{d} \) = 0.252 p.u.,\( X_{q} \) = 0.474 p.u.,\( X^{\prime}_{q} \) = 0.243 p.u.,\( X^{\prime\prime}_{q} \) = 0.18 p.u., \( T_{d} \) = 1.01 s, \( T^{\prime}_{d} \) = 0.053 s, \( T^{\prime\prime}_{qo} \) = 0.1 s.

Load at Bus-2 250 MW.

Transformer 2100 MVA, 13.8/500 kV, 60 Hz, R1 = R2 = 0.002 p.u., L1 = 0, L2 = 0.12 p.u., D1/Yg connection, Rm = 500 p.u., Lm = 500 p.u.

Transmission line 3-Ph, 60 Hz, Length = 300 km each, R1 = 0.02546 Ω/km, R0 = 0.3864 Ω/km, L1 = 0.9337e−3 H/km, L0 = 4.1264e−3 H/km, C1 = 12.74e−9 F/km, C0 = 7.751e−9 F/km.

Hydraulic Turbine and Governor Ka = 3.33, Ta = 0.07, Gmin = 0.01, Gmax = 0.97518, Vgmin = − 0.1 p.u./s, Td = 0.01 s, β = 0 Tw = 2.67 s Vgmax = 0.1 p.u./s, Rp = 0.05, Kp = 1.163, Ki = 0.10

1.2 Four-machine eleven-bus power system

Generators SB = SB2 = SB3 = SB4 = 900MVA, VB = 20 kV, f = 60 Hz, \( {\text{X}}_{\text{d}} \) = 1.8, \( X^{\prime}_{d} \) = 0.3, \( X^{\prime\prime}_{d} \) = 0.25 pu, \( X_{q} \) = 1.7 pu, \( X^{\prime}_{q} \) = 0.55, \( X^{\prime\prime}_{q} \) = 0.25 pu, \( T_{d} \) = 8 s; \( T^{\prime}_{d} \) = 0.03 s, \( T^{\prime\prime}_{qo} \) = 0.05 s, RS = 2.5e−3, H = 6.5 s, p = 4.

Transformers SB1 = SB2 = SB3 = SB4 = 900 MVA, D1/Yg, V1 = 20 kV, V2 = 230 kV, R1 = R2 = 1e−6 pu, L1 = 0, L2 = 0.15 pu, Rm = 500 pu, Lm = 500 pu.

Transmission lines 3-Ph, R1 = 0.0529 Ω/km, R0 = 1.61 Ω/km, L1 = 1.403e−3H/km, L0 = 6.1e−3 H/km, C1 = 8.775e−9 F/km, C0 = 5.249e−9 F/km.

Loads Load 9 = 967 MW − 987 MVAR, Load 7 = 1767 MW − 437 MVAR.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Darvish Falehi, A. Optimal robust disturbance observer based sliding mode controller using multi-objective grasshopper optimization algorithm to enhance power system stability. J Ambient Intell Human Comput 11, 5045–5063 (2020). https://doi.org/10.1007/s12652-020-01811-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12652-020-01811-8

Keywords