Skip to main content

Advertisement

Springer Nature Link
Log in

Cubic intuitionistic WASPAS technique and its application in multi-criteria decision-making

  • Original Research
  • Published:
Journal of Ambient Intelligence and Humanized Computing Aims and scope Submit manuscript

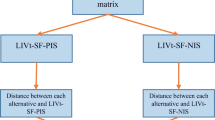
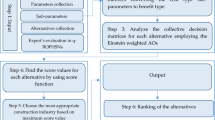
Abstract

Multi-criteria decision-making (MCDM) approaches have acquired various expansions under uncertain conditions in current years. The purpose of the current study is to broaden the implementation of the weighted aggregated sum product assessment (WASPAS) technique for decision-making (DM) in an uncertain environment. Thinking about the benefits of cubic intuitionistic sets (CIS) in dealing with the issue of uncertainty, the improvement of the cubic intuitionistic WASPAS (CI-WASPAS) technique for DM is taken into consideration within the paper. Experimental outcomes obtained for a real-world issue regarding bridge construction selection indicates that the suggested technique performs well compared to other established classification techniques.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  • Atanassov KT (1986) Intuitionistic fuzzy sets. Fuzzy Sets Syst 20:87–96

    Article  MATH  Google Scholar 

  • Atanassov KT, Gargov G (1989) Interval-valued intuitionistic fuzzy sets. Fuzzy Sets Syst 31:343–349

    Article  MathSciNet  MATH  Google Scholar 

  • Bausys R, Juodagalviene B (2017) Garage location selection for residential house by WASPAS-SVNS method. J Civ Eng Manag 23(3):421–429

    Article  Google Scholar 

  • Bridgman PW (1922) Dimensional analysis. Yale University Press, New Haven, p 1922

    Google Scholar 

  • Chakraborty S, Zavadskas EK (2014) Applications of WASPAS method in manufacturing decision making. Informatica 25(1):1–20

    Article  Google Scholar 

  • Chen TY (2012) Nonlinear assignment-based methods for interval-valued intuitionistic fuzzy multi-criteria decision analysis with incomplete preference information. Int J Inform Technol Dec Mak 11(4):821–855

    Article  Google Scholar 

  • Fahmi A, Abdullah S, Amin F, Ali A, Khan WA (2018) Some geometric operators with triangular cubic linguistic hesitant fuzzy number and their application in group decision-making. J Intell Fuzzy Syst 35(2):2485–2499

    Article  Google Scholar 

  • Fahmi A, Abdullah S, Amin F, Khan MSA (2019) Trapezoidal cubic fuzzy number Einstein hybrid weighted averaging operators and its application to decision making. Soft Comput 23:5753–5783

    Article  MATH  Google Scholar 

  • Garg H (2016) A new generalized improved score function of intervalvalued intuitionistic fuzzy sets and applications in expert systems. Appl Soft Comput 38:988–999

    Article  Google Scholar 

  • Garg H, Kaur G (2020a) Novel distance measures for cubic intuitionistic fuzzy sets and their applications to pattern recognitions and medical diagnosis. Granul Comput 5:169–184

    Article  Google Scholar 

  • Garg H, Kaur G (2020b) Extended TOPSIS method for multi-criteria group decision-making problems under cubic intuitionistic fuzzy environment. Sci Iran E 27(1):396–410

    Google Scholar 

  • Ghorshi Nezhad MR, Zolfani SH, Moztarzadeh F, Zavadskas EK, Bahrami M (2015) Planning the priority of high tech industries based on SWARA-WASPAS methodology: the case of the nanotechnology industry in Iran. Econ Res Ekonomska Istrazivanja 28:1111–1137

    Article  Google Scholar 

  • Hashemkhani Zolfani S, Aghdaie MH, Derakhti A, Zavadskas EK, Varzandeh MHM (2013) Decision making on business issues with foresight perspective; an application of new hybrid MCDM model in shopping mall locating. Exp Syst Appl 40(17):7111–7121

    Article  Google Scholar 

  • Jun YB (2017) A novel extension of cubic sets and its applications in BCK/BCI-algebras. Ann Fuzzy Math Inform 14(5):475–486

    Article  MathSciNet  MATH  Google Scholar 

  • Jun YB, Kim CS, Yang KO (2012) Cubic sets. Ann Fuzzy Math Inform 4:83–98

    MathSciNet  MATH  Google Scholar 

  • Kaur G, Garg H (2018a) Cubic intuitionistic fuzzy aggregation operators. Int J Uncertain Quantif 8(5):405–427

    Article  MathSciNet  Google Scholar 

  • Kaur G, Garg H (2018b) Generalized cubic intuitionistic fuzzy aggregation operators using t-norm operations and their applications to group decision-making process. Arab J Sci Eng 44(3):1–20

    Google Scholar 

  • Kaur G, Garg H (2018b) Multi-attribute decision-making based on bonferroni mean operators under cubic intuitionistic fuzzy set environment. Entropy 20(1): 65: https://doi.org/10.3390/e20010065.

  • Keshavarz Ghorabaee M, Zavadskas EK, Amiri M, Esmaeili A (2016) Multi-criteria evaluation of green suppliers using an extended WASPAS method with interval type-2 fuzzy sets. J Clean Prod 137:213–229

    Article  Google Scholar 

  • Keshavarz Ghorabaee M, Amiri M, Zavadskas EK, Antuchevičienċ J (2017) Assessment of third-party logistics providers using a CRITIC-WASPAS approach with interval type-2 fuzzy sets. Transport 32(1):66–78

    Article  Google Scholar 

  • Khan M, Abdullah S, Zeb A, Majid A (2016) Cubic aggregation operators. Int J Comput Sci Inf Secur 14(8):670–682

    Google Scholar 

  • Madic M, Gecevska V, Radovanovic M, Petkovic D (2014) multi-criteria economic analysis of machining processes using the WASPAS method. J Prod Eng 17:79–82

    Google Scholar 

  • Mardani A, Nilashi M, Zakuan N, Loganathan N, Soheilirad S, Saman MZM, Ibrahim O (2017) A systematic review and meta-Analysis of SWARA and WASPAS methods: theory and applications with recent fuzzy developments. Appl Soft Comput 57:265–292

    Article  Google Scholar 

  • Miller DW, Starr MK (1969) Executive decisions and operations research. Prentice-Hall Inc, Englewood Cliffs

    Google Scholar 

  • Nayagam VLG, Muralikrishnan S, Sivaraman G (2011) Multicriteria decision-making method based on interval-valued intuitionistic fuzzy sets. Expert Syst Appl 38(3):1464–1467

    Article  Google Scholar 

  • Peng X, Dai J (2017) Hesitant fuzzy soft decision making methods based on WASPAS, MABAC and COPRAS with combined weights. J Intell Fuzzy Systems 33(2):1313–1325

    Article  MATH  Google Scholar 

  • Senapati T, Shum KP (2017) Cubic implicative ideals of \(BCK\)-algebras. Missouri J Math Sci 29(2):125–138

    Article  MathSciNet  MATH  Google Scholar 

  • Senapati T, Shum KP (2018) Cubic commutative ideals of \(BCK\)-algebras. Missouri J Math Sci 30(1):5–19

    Article  MathSciNet  MATH  Google Scholar 

  • Senapati T, Kim CS, Bhowmik M, Pal M (2015) Cubic subalgebras and cubic closed ideals of \(B\)-algebras. Fuzzy Inf Eng 7(2):129–149

    Article  MathSciNet  Google Scholar 

  • Senapati T, Jun YB, Shum KP (2018) Cubic set structure applied in \(UP\)-algebras. Discrete Math Algorithms Appl 10(4):1850049. https://doi.org/10.1142/S1793830918500490

    Article  MathSciNet  MATH  Google Scholar 

  • Senapati T, Jun YB, Shum KP (2019) Cubic intuitionistic subalgebras and closed cubic intuitionistic ideals of \(B\)-algebras. J Intell Fuzzy Syst 36(2):1563–1571

    Article  Google Scholar 

  • Senapati T, Jun YB, Muhiuddin G, Shum KP (2019) Cubic intuitionistic structures applied to ideals of \(BCI\)-algebras. An St Univ Ovidius Constanta 27(2):213–232

    MathSciNet  MATH  Google Scholar 

  • Senapati T, Jun YB, Shum KP (2020a) Cubic intuitionistic implicative ideals of \(BCK\)-algebras. Proc Natl Acad Sci India, Sect A Phys Sci. https://doi.org/10.1007/s40010-020-00674-0

    Article  MATH  Google Scholar 

  • Senapati T, Jun YB, Shum KP (2020b) Cubic intuitionistic structure of KU-algebras. Afr Mat 31(2):237–248

    Article  MathSciNet  MATH  Google Scholar 

  • Senapati T, Jana C, Pal M, Jun YB (2018) Cubic intuitionistic \(q\)-ideals of \(BCI\)-algebras. Symmetry 10(12):752; https://doi.org/10.3390/sym10120752

  • Sivaraman G, Nayagam VLG, Ponalagusamy R (2013) Multi-criteria interval valued intuitionistic fuzzy decision making using a new score function. In: KIM 2013 Knowledge and Information Management Conference, pp. 122–131

  • Stević Z, Pamučar D, Subotić M, Antuchevičiene J, Zavadskas EK (2018) The location selection for roundabout construction using rough bwm-rough waspas approach based on a new rough hamy aggregator. Sustainability 10(8):2817

    Article  Google Scholar 

  • Stojić G, Stević Z, Antuchevičienė J, Pamučar D, Vasiljević M (2018) A novel rough WASPAS approach for supplier selection in a company manufacturing PVC carpentry products. Information 9(5):121

    Article  Google Scholar 

  • Triantaphyllou E (2000) Multi-Criteria Decision Making: A Comparative Study. Dordrecht, The Netherlands: Kluwer Academic Publishers (now Springer), 320 pages, 2000, ISBN 0-7923-6607-7

  • Triantaphyllou E, Mann SH (1989) An examination of the effectiveness of multi-dimensional decision-making methods: a decision-making paradox. Decis Support Syst 5(3):303–312

    Article  Google Scholar 

  • Turskis Z, Zavadskas EK, Antucheviciene J, Kosareva N (2015) A hybrid model based on fuzzy AHP and fuzzy WASPAS for construction site selection. Int J Comput Commun Control 10(6):113–128

    Article  Google Scholar 

  • Urosevic S, Karabasevic D, Stanujkic D, Maksimovic M (2017) An approach to personnel selection in the tourism industry based on the SWARA and the WASPAS methods. Econ Comput Econ Cybern Stud Res 51(1):75–88

    Google Scholar 

  • Wang W, Liu X (2013a) Interval-valued intuitionistic fuzzy hybrid weighted averaging operator based on Einstein operation and its application to decision making. J Intell Fuzzy Syst 25(2):279–290

    Article  MathSciNet  MATH  Google Scholar 

  • Wang W, Liu X (2013b) The multi-attribute decision making method based on interval-valued intuitionistic fuzzy Einstein hybrid weighted geometric operator. Comput Math Appl 66(10):1845–1856

    Article  MathSciNet  MATH  Google Scholar 

  • Xu Z (2007) Methods for aggregating interval-valued intuitionistic fuzzy information and their application to decision making. Control Decis 22(2):215–219

    Google Scholar 

  • Xu Z, Chen J (2007) On geometric aggregation over interval-valued intuitionistic fuzzy information. In: The 3rd international conference on natural computation (ICNC‘07) and the fourth international conference on fuzzy systems and knowledge discovery (FSKD‘07), Haikou, China, 2:466–471

  • Ye J (2009) Multicriteria fuzzy decision-making method based on a novel accuracy function under interval-valued intuitionistic fuzzy environment. Expert Syst Appl 36:6899–6902

    Article  Google Scholar 

  • Zadeh LA (1965) Fuzzy sets. Inform Control 8:338–353

    Article  MATH  Google Scholar 

  • Zavadskas EK, Turskis Z, Antucheviciene J, Zakarevicius A (2012)Optimization of weighted aggregated sum product assessment. Electron Elect Eng 122(6):3–6

  • Zavadskas EK, Turskis Z, Antucheviciene J, Zakarevicius A (2012) Optimization of weighted aggregated sum product assessment. Elektronika ir elektrotechnika 122(6):3–6

    Article  Google Scholar 

  • Zavadskas EK, Antucheviciene J, Razavi Hajiagha SH, Hashemi SS (2014) Extension of weighted aggregated sum product assessment with interval-valued intuitionistic fuzzy numbers (WASPAS-IVIF). Appl soft comput 24:1013–1021

    Article  Google Scholar 

  • Zavadskas EK, Bausys R, Lazauskas M (2015) Sustainable assessment of alternative sites for the construction of a waste incineration plant by applying WASPAS method with single-valued neutrosophic set. Sustainability 7(12):1–14

    Google Scholar 

  • Zavadskas EK, Turskis Z, Antucheviciene J (2015) Selecting a contractor by using a novel method for multiple attribute analysis: weighted aggregated sum product assessment with grey values (WASPAS-G). Stud Inform Control 24(2):141–150

    Article  Google Scholar 

  • Zavadskas EK, Bausys R, Stanujkic D (2016) Selection of lead-zinc flotation circuitdesign by applying WASPAS method with single-valued neutrosophic set. Acta Montanist Slovaca 21:85–92

    Google Scholar 

Download references

Acknowledgements

The author is very grateful to the anonymous referees for their insightful and constructive comments and suggestions, which have been very helpful in improving the paper. This work is supported by the National Natural Science Foundation of China (Grant No-11671324).

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Southwest University, Beibei, Chongqing, 400715, China

    Tapan Senapati

  2. Machine Intelligence Institute, Iona College, New Rochelle, NY, 10801, USA

    Ronald R. Yager

  3. School of Mathematics and Statistics, Southwest University, Beibei, Chongqing, 400715, China

    Guiyun Chen

Authors
  1. Tapan Senapati
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Ronald R. Yager
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Guiyun Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Tapan Senapati.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Senapati, T., Yager, R.R. & Chen, G. Cubic intuitionistic WASPAS technique and its application in multi-criteria decision-making. J Ambient Intell Human Comput 12, 8823–8833 (2021). https://doi.org/10.1007/s12652-020-02667-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12652-020-02667-8

Keywords