Computing stationary distributions of the D-MAP/D-MSP$^{(a,b) }/1$ queueing system

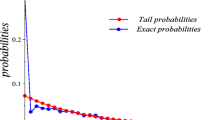
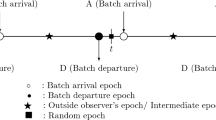
Abstract

The present study investigates a single-server batch service queueing model with arrival process as discrete-time Markovian arrival process and service process as discrete-time Markovian service process. The server serves the customers in batches followed by the general bulk-service rule. We first determine the random epoch probabilities using the matrix-geometric method, where the rate matrix ${\mathbf{R}}$ is determined by an efficient approach based on the eigenvalues and the corresponding eigenvectors of the associated characteristic equation. Next we obtain the explicit closed-form expressions for the pre-arrival, intermediate, outside observer’s and post-departure epoch probabilities by developing the relations among them in equilibrium state. Further, we provide an analytically simple approach to carry out the waiting-time distribution in the queue measured in slots as well as the distribution of the size of a service batch of an arriving customer. We also demonstrate a cost function to evaluate the optimum value of the minimum service batch size and the corresponding expected cost of the system. Finally, an adequate variety of numerical experiments are performed for validation purpose of our analytical results and they are conferred in the form of tables and graphs.

Appendix: Commuting matrices

Horváth et al. (2014) proved that ${\mathbf{R}}$ and $({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+{\mathbf{R}}({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_1)$ commute for continuous-time MAP/MSP/1 queueing model. Now, we extend the proof of the commutativity for our proposed bulk-service discrete-time queueing model, i.e., ${\mathbf{R}}$ and $({\mathbf{I}}_{m_1}\otimes \mathbf{L}_0)+{\mathbf{R}}^b({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_1)$ commute. According to the spectral decomposition approach conferred in Horváth et al. (2014), we establish the commutativity for our queueing model.

Assumption 1

We assume that the matrices ${\mathbf{D}}_1+\alpha _i{\mathbf{D}}_0$ and $\mathbf{L}_0+\alpha _i^b{\mathbf{L}}_1$ can be diagonalized for $i=1,2,\ldots , m$.

Lemma 1

If $\mathbf{U}$ and $\mathbf{V}$ be two square matrices of order $m_1$ and $m_2$, respectively, such that they are diagonalizable then $\mathbf{U}\otimes \mathbf{V}$ is also diagonalizable. Moreover, if $\mathbf{u}_i$ is the left eigenvector of $\mathbf{U}$ corresponding to the eigenvalue $\theta _i$ for $i=1,2,\ldots ,m_1$ and $\mathbf{v}_j$ is the left eigenvector of $\mathbf{V}$ corresponding to the eigenvalue $\delta _j$ for $j=1,2,\ldots ,m_2$, then $\mathbf{U}\otimes \mathbf{V}$ has left eigenvector $\mathbf{u}_i\otimes \mathbf{v}_j$ corresponding to the eigenvalue $\theta _i\delta _j$.

Lemma 2

If ${{\varvec{\eta }}}_i$ be the left eigenvector of the matrix ${\mathbf{R}}$ corresponding to the eigenvalue $\alpha _i$ for $i=1,2,\ldots , m$, then ${{\varvec{\eta }}}_i$ is also left eigenvector of $({\mathbf{I}}_{m_1}\otimes ({\mathbf{L}}_0+\alpha _i^b\mathbf{L}_1))$.

Proof

Pre-multiplying (6) by ${\varvec{\eta }}_i$ and using ${{\varvec{\eta }}}_i\mathbf{R}=\alpha _i{{\varvec{\eta }}}_i$, for $i=1,2,\ldots , m$, we have

$$\begin{aligned} \alpha _i{{\varvec{\eta }}}_i= & {} {\varvec{\eta }}_i\left[ ({\mathbf{D}}_1 \otimes \mathbf{L}_0)+\alpha _i^{b}({\mathbf{D}}_1 \otimes \mathbf{L}_1)\right] +\alpha _i{\varvec{\eta }}_i\left[ ({\mathbf{D}}_0 \otimes {\mathbf{L}}_0)\right. \nonumber \\&\left. +{\alpha _i}^{b}({\mathbf{D}}_0 \otimes {\mathbf{L}}_1)\right] \nonumber \\= & {} {\varvec{\eta }}_i\left[ ({\mathbf{D}}_1+\alpha _i{\mathbf{D}}_0) \otimes (\mathbf{L}_0+\alpha _i^b{\mathbf{L}}_1)\right] . \end{aligned}$$

(17)

This implies that ${\varvec{\eta }}_i$ is the left eigenvector of $\left[ (\mathbf{D}_1+\alpha _i{\mathbf{D}}_0) \otimes ({\mathbf{L}}_0+\alpha _i^b\mathbf{L}_1)\right]$ corresponding to the eigenvalue $\alpha _i$. According to our above Assumption 1 and Lemma 1, if ${{\varvec{\upsilon }}}_{i,j}$ is the left eigenvector of $({\mathbf{D}}_1+\alpha _i\mathbf{D}_0)$ corresponding to the eigenvalue $\xi _{i,j}$, for $j=1,2,\ldots , m_1$, and ${{\varvec{\phi }}}_{i,k}$ is the left eigenvector of $({\mathbf{L}}_0+\alpha _i^b{\mathbf{L}}_1)$ corresponding to the eigenvalue $\gamma _{i,k}$, for $k=1,2,\ldots , m_2$ then we have ${\varvec{\eta }}_i={\varvec{\upsilon }}_{i,p_i}\otimes {\varvec{\phi }}_{i,r_i}$ and $\alpha _i=\xi _{i,p_i}\gamma _{i,r_i}$, for some $p_i\in \{1,2,\ldots , m_1\}$ and $r_i\in \{1,2,\ldots ,m_2\}$. Therefore, we have

$$\begin{aligned}&{\varvec{\eta }}_i ({\mathbf{I}}_{m_1}\otimes ({\mathbf{L}}_0+\alpha _i^b\mathbf{L}_1))\\&\quad =({\varvec{\upsilon }}_{i,p_i}\otimes {\varvec{\phi }}_{i,r_i}) ({\mathbf{I}}_{m_1}\otimes ({\mathbf{L}}_0+\alpha _i^b{\mathbf{L}}_1))\\&\quad ={\varvec{\upsilon }}_{i,p_i}\otimes {\varvec{\phi }}_{i,r_i}({\mathbf{L}}_0+\alpha _i^b{\mathbf{L}}_1)\\&\quad ={\varvec{\upsilon }}_{i,p_i}\otimes \gamma _{i,r_i}{\varvec{\phi }}_{i,r_i}\\&\quad =\gamma _{i,r_i}{\varvec{\eta }}_i. \end{aligned}$$

Hence, it is proved that ${{\varvec{\eta }}}_i$ is the left eigenvector of $({\mathbf{I}}_{m_1}\otimes ({\mathbf{L}}_0+\alpha _i^b{\mathbf{L}}_1))$. $\square$

Lemma 3

If ${{\varvec{\eta }}}_i$ be the left eigenvector of the matrix ${\mathbf{R}}$ corresponding to the eigenvalue $\alpha _i$ for $i=1,2,\ldots , m$, then ${{\varvec{\eta }}}_i$ is also the left eigenvector of $({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+{\mathbf{R}}^b(\mathbf{I}_{m_1}\otimes {\mathbf{L}}_1)$ corresponding to the eigenvalue $\gamma _{i,r_i}.$

Proof

Using Lemma 2, we can write

$$\begin{aligned}&{\varvec{\eta }}_i\left[ ({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+{\mathbf{R}}^b(\mathbf{I}_{m_1}\otimes {\mathbf{L}}_1)\right] \\&\quad ={\varvec{\eta }}_i\left[ (\mathbf{I}_{m_1}\otimes {\mathbf{L}}_0)+\alpha _i^b({\mathbf{I}}_{m_1}\otimes \mathbf{L}_1)\right] \\&\quad ={\varvec{\eta }}_i ({\mathbf{I}}_{m_1}\otimes (\mathbf{L}_0+\alpha _i^b{\mathbf{L}}_1))\\&\quad =\gamma _{i,r_i}{\varvec{\eta }}_i. \end{aligned}$$

$\square$

Theorem

Under Assumption 1, the matrices ${\mathbf{R}}$ and $({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+{\mathbf{R}}^b(\mathbf{I}_{m_1}\otimes {\mathbf{L}}_1)$ commute.

Proof

From Lemma 3, we can say that ${\mathbf{R}}$ and $({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+{\mathbf{R}}^b({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_1)$ are simultaneously diagonalizable. Therefore, there exists a matrix ${\mathbf{Y}}$ such that

$$\begin{aligned} {\mathbf{R}}= & {} \Big [{\mathbf{Y}}{\varvec{\Delta }}{\mathbf{Y}}^{-1}\Big ]^T ~\text {and}~({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+{\mathbf{R}}^b(\mathbf{I}_{m_1}\otimes {\mathbf{L}}_1)\nonumber \\= & {} \Big [{\mathbf{Y}}{\widehat{\varvec{\Delta }}}\mathbf{Y}^{-1}\Big ]^T, \end{aligned}$$

(18)

where

$$\begin{aligned} {\widehat{\varvec{\Delta }}}=\left[ \begin{array}{ccccc} \gamma _{1,r_1}&{} &{} &{} &{} \\ &{} \gamma _{2,r_2}&{} &{} &{} \\ &{} &{} \ddots &{} &{} \\ &{} &{} &{} &{} \gamma _{m,r_m} \end{array} \right] . \end{aligned}$$

Now, using (18), we have

$$\begin{aligned}&{\mathbf{R}}\left[ ({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+{\mathbf{R}}^b(\mathbf{I}_{m_1}\otimes {\mathbf{L}}_1)\right] \\&\quad =({\mathbf{Y}}^T)^{-1}{\varvec{\Delta }}({\mathbf{Y}}^T)({\mathbf{Y}}^T)^{-1}{\widehat{\varvec{\Delta }}}(\mathbf{Y}^T)\\&\quad =({\mathbf{Y}}^T)^{-1}{\varvec{\Delta }}{{\widehat{\varvec{\Delta }}}}(\mathbf{Y}^T)\\&\quad =({\mathbf{Y}}^T)^{-1}{\widehat{\varvec{\Delta }}}{\varvec{\Delta }}(\mathbf{Y}^T)\\&\quad =({\mathbf{Y}}^T)^{-1}{{\widehat{\varvec{\Delta }}}}({\mathbf{Y}}^T)(\mathbf{Y}^T)^{-1}{\varvec{\Delta }}({\mathbf{Y}}^T)\\&\quad =\left[ ({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_0)+ {\mathbf{R}}^b({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_1)\right] \mathbf{R}. \end{aligned}$$

Hence, it is proved that ${\mathbf{R}}$ and $({\mathbf{I}}_{m_1}\otimes \mathbf{L}_0)+ {\mathbf{R}}^b({\mathbf{I}}_{m_1}\otimes {\mathbf{L}}_1)$ commute each other. $\square$