Skip to main content
Springer Nature Link
Log in

Emergency decision support modeling under generalized spherical fuzzy Einstein aggregation information

  • Original Research
  • Published:
Journal of Ambient Intelligence and Humanized Computing Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

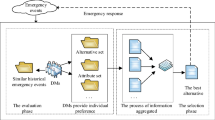
Dominant emergency action should be adopted in the case of an emergency situation. Emergency is interpreted as limited time and information, harmfulness and uncertainty, and decision-makers are often critically bound by uncertainty and risk. This framework implements an emergency decision-making approach to address the emergency situation of COVID-19 in a spherical fuzzy environment. As the spherical fuzzy set (SFS) is a generalized framework of fuzzy structure to handle more uncertainty and ambiguity in decision-making problems (DMPs). Keeping in view the features of the SFSs, the purpose of this paper is to present some robust generalized operating laws in accordance with the Einstein norms. In addition, list of propose aggregation operators using Einstein operational laws under spherical fuzzy environment are developed. Furthermore, we design the algorithm based on the proposed aggregation operators to tackle the uncertainty in emergency decision making problems. Finally, numerical case study of COVID-19 as an emergency decision making is presented to demonstrate the applicability and validity of the proposed technique. Besides, the comparison of the existing and the proposed technique is established to show the effectiveness and validity of the established technique.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

No data were used to support this study.

References

  • Ashraf S, Abdullah S, Mahmood T (2018) GRA method based on spherical linguistic fuzzy Choquet integral environment and its application in multi-attribute decision-making problems. Math Sci 12(4):263–275

    Article  Google Scholar 

  • Ashraf S, Abdullah S (2019) Spherical aggregation operators and their application in multiattribute group decision-making. Int J Intell Syst 34(3):493–523

    Article  Google Scholar 

  • Ashraf S, Abdullah S, Mahmood T, Ghani F, Mahmood T (2019) Spherical fuzzy sets and their applications in multi-attribute decision making problems. J Intell Fuzzy Syst 36(3):2829–2844

    Article  Google Scholar 

  • Ashraf S, Abdullah S, Abdullah L (2019) Child development influence environmental factors determined using spherical fuzzy distance measures. Mathematics 7(8):661

    Article  Google Scholar 

  • Ashraf S, Abdullah S, Aslam M, Qiyas M, Kutbi MA (2019) Spherical fuzzy sets and its representation of spherical fuzzy t-norms and t-conorms. J Intell Fuzzy Syst 36(6):6089–6102

    Article  Google Scholar 

  • Ashraf S, Abdullah S, Mahmood T (2020) Spherical fuzzy Dombi aggregation operators and their application in group decision making problems. J Amb Intel Hum Comp 11:2731–2749

    Article  Google Scholar 

  • Ashraf S, Abdullah S, Almagrabi AO (2020) A new emergency response of spherical intelligent fuzzy decision process to diagnose of COVID19. Soft Comput. https://doi.org/10.1007/s00500-020-05287-8

    Article  Google Scholar 

  • Ashraf S, Abdullah S, Aslam M (2020) Symmetric sum based aggregation operators for spherical fuzzy information: Application in multi-attribute group decision making problem. J Intell Fuzzy Syst 38(4):5241–5255

    Article  Google Scholar 

  • Ashraf S, Abdullah S (2020) Emergency decision support modeling for COVID-19 based on spherical fuzzy information. Int J Intell Syst 35(11):1601–1645

    Article  Google Scholar 

  • Atanassov KT (1986) Intuitionistic fuzzy sets. Fuzzy Set Syst 20:87–96

    Article  Google Scholar 

  • Baležentis T, Štreimikienė D, Melnikienė R, Zeng S (2019) Prospects of green growth in the electricity sector in Baltic States: Pinch analysis based on ecological footprint. Resour Conserv Recy 142:37–48

    Article  Google Scholar 

  • Barukab O, Abdullah S, Ashraf S, Arif M, Khan SA (2019) A new approach to fuzzy topsis method based on entropy measure under spherical fuzzy information. Entropy 21(12):1231

    Article  MathSciNet  Google Scholar 

  • Centers for Disease Control and Prevention, https://www.cdc.gov/coronavirus/2019-nCoV/index.html

  • Coronavirus COVID-19 Global Cases by the Center for Systems Science and Engineering (CSSE) at Johns Hopkins University (JHU). ArcGIS. Johns Hopkins CSSE. Retrieved 15 NOV 2020

  • Coronavirus COVID-19 situation of Pakistan and Worldwide, http://covid.gov.pk/stats/global

  • Cuong BC, Kreinovich V. Picture Fuzzy Sets-a new concept for computational intelligence problems. In2013 Third World Congress on Information and Communication Technologies (WICT 2013) 2013 (pp. 1-6). IEEE

  • Garg H, Munir M, Ullah K, Mahmood T, Jan N (2018) Algorithm for T-spherical fuzzy multi-attribute decision making based on improved interactive aggregation operators. Symmetry 10(12):670

    Article  Google Scholar 

  • Garg H (2016) Generalized intuitionistic fuzzy interactive geometric interaction operators using Einstein t-norm and t-conorm and their application to decision making. Comput Ind Eng 101:53–69

    Article  Google Scholar 

  • Garg H (2016) A new generalized Pythagorean fuzzy information aggregation using Einstein operations and its application to decision making. Int J Intell Syst 31(9):886–920

    Article  Google Scholar 

  • Garg H (2017) Generalized Pythagorean fuzzy geometric aggregation operators using Einstein t-norm and t-conorm for multicriteria decision-making process. Int J Intell Syst 32(6):597–630

    Article  Google Scholar 

  • Goala S, Dutta P (2018) Detection of area under potential threat via an advanced aggregation operator on generalized triangular fuzzy number. J Taibah Univ Sci 12(5):536–544

    Article  Google Scholar 

  • Hussain A, Ali MI, Mahmood T (2020) Pythagorean fuzzy soft rough sets and their applications in decision-making. J Taibah Univ Sci 14(1):101–113

    Article  Google Scholar 

  • Hwang CL, Yoon K (1981) Methods for multiple attribute decision making. Multiple attribute decision making. Springer, Berlin, pp 58–191

    Chapter  Google Scholar 

  • Jan N, Mahmood T, Zedam L, Abdullah L, Ullah K (2021) Analysis of double domination by using the concept of spherical fuzzy information with application. J Ambient Intell Human Comput. https://doi.org/10.1007/s12652-021-03370-y

    Article  Google Scholar 

  • Jin Y, Ashraf S, Abdullah S (2019) Spherical fuzzy logarithmic aggregation operators based on entropy and their application in decision support systems. Entropy 21(7):628

    Article  MathSciNet  Google Scholar 

  • Jin Y, Wu H, Sun D, Zeng S, Luo D, Peng B (2019) A multi-attribute Pearson’s picture fuzzy correlation-based decision-making method. Mathematics 7(10):999

    Article  Google Scholar 

  • Jin H, Ashraf S, Abdullah S, Qiyas M, Bano M, Zeng S (2019) Linguistic spherical fuzzy aggregation operators and their applications in multi-attribute decision making problems. Mathematics 7(5):413

    Article  Google Scholar 

  • Khan S, Abdullah S, Ashraf S (2019) Picture fuzzy aggregation information based on Einstein operations and their application in decision making. Math Sci. 13(3):213–229

    Article  MathSciNet  Google Scholar 

  • Kutlu Gundogdu F, Kahraman C (2019) Extension of WASPAS with spherical fuzzy sets. Informatica 30(2):269–292

    Article  Google Scholar 

  • Kutlu Gündoğdu F, Kahraman C (2019) A novel VIKOR method using spherical fuzzy sets and its application to warehouse site selection. J Intell Fuzzy Syst 37(1):1197–1211

    Article  Google Scholar 

  • Qiyas M, Abdullah S, Liu Y, Naeem M (2021) Multi-criteria decision support systems based on linguistic intuitionistic cubic fuzzy aggregation operators. J Ambient Intell Human Comput 12:8285–8303

    Article  Google Scholar 

  • Rahman K, Abdullah S, Ali A, Amin F (2019) Interval-valued Pythagorean fuzzy Einstein hybrid weighted averaging aggregation operator and their application to group decision making. Comp Intell Syst 5(1):41–52

    Article  Google Scholar 

  • Rahman K, Abdullah S, Ghani F (2019) Some new generalized interval-valued Pythagorean fuzzy aggregation operators using einstein t-norm and t-conorm. J Intell Fuzzy Syst 37(3):3721–3742

    Article  Google Scholar 

  • Rahman K, Abdullah S, Khan MSA (2020) Some interval-valued Pythagorean fuzzy Einstein weighted averaging aggregation operators and their application to group decision making. J Intell Syst 29(1):393–408

    Article  Google Scholar 

  • Rafiq M, Ashraf S, Abdullah S, Mahmood T, Muhammad S (2019) The cosine similarity measures of spherical fuzzy sets and their applications in decision making. J Intell Fuzzy Syst 36:6059–6073

    Article  Google Scholar 

  • Saaty TL (2008) Decision making with the analytic hierarchy process. Int J Serv Sci 1(1):83–98

    Google Scholar 

  • Saha A, Majumder P, Dutta D, Debnath BK (2021) Multi-attribute decision making using q-rung orthopair fuzzy weighted fairly aggregation operators. J Amb Intel Hum Comp 12:8149–8171

    Article  Google Scholar 

  • WHO Coronavirus Disease (COVID-19) Dashboard 2021. https://covid19.who.int/

  • Yager RR. Pythagorean fuzzy subsets. In2013 joint IFSA world congress and NAFIPS annual meeting (IFSA/NAFIPS) 2013 Jun 24 (pp. 57–61). IEEE

  • Yager RR, Abbasov AM (2013) Pythagorean membership grades, complex numbers, and decision making. Int J Intell Syst 28(5):436–452

    Article  Google Scholar 

  • Zadeh LA (1965) Fuzzy sets. Inf control 8:338–353

    Article  Google Scholar 

  • Zeng S, Hussain A, Mahmood T, Ali MI, Ashraf S, Munir M (2019) Covering-based spherical fuzzy rough set model hybrid with TOPSIS for multi-attribute decision-making. Symmetry 11(4):547

    Article  Google Scholar 

  • Zeng S, Chen SM, Kuo LW (2019) Multiattribute decision making based on novel score function of intuitionistic fuzzy values and modified VIKOR method. Inf Sci 488:76–92

    Article  Google Scholar 

  • Zhang X, Xu Z (2014) Extension of TOPSIS to multiple criteria decision making with Pythagorean fuzzy sets. Int J Intell Syst 29(12):1061–1078

    Article  MathSciNet  Google Scholar 

  • Zimmerman TG (1996) Personal area networks: near-field intrabody communication. IBM syst J 35(3.4):609–617

    Article  Google Scholar 

Download references

Acknowledgement

This study was supported by Higher Education Commission (HEC), Pakistan under National Research Program for University (NRPU), Project title: Fuzzy Mathematical Modeling for Decision Support Systems and Smart Grid Systems (No. 10701/KPK/NRPU/R&D/HEC/2017).

Author information

Authors and Affiliations

  1. Department of Mathematics and Statistics, Bacha Khan University, Charsadda, 24420, Khyber Pakhtunkhwa, Pakistan

    Shahzaib Ashraf

  2. Department of Mathematics, Abdul Wali Khan University, Mardan, 23200, Khyber Pakhtunkhwa, Pakistan

    Saleem Abdullah

  3. Department of Mathematics and Statistics, Faculty of Science, Prince of Songkla University, Hat Yai, Songkhla, 90110, Thailand

    Ronnason Chinram

Authors
  1. Shahzaib Ashraf
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Saleem Abdullah
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Ronnason Chinram
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Shahzaib Ashraf.

Ethics declarations

Conflict of Interest

The authors declare that they have no conflict of interest regarding the publication of the research article.

Ethical approval

This article does not contain any studies with human participants or animals performed by any of the authors.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ashraf, S., Abdullah, S. & Chinram, R. Emergency decision support modeling under generalized spherical fuzzy Einstein aggregation information. J Ambient Intell Human Comput 13, 2091–2117 (2022). https://doi.org/10.1007/s12652-021-03493-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12652-021-03493-2

Keywords