Secrecy performance of a novel energy efficient hybrid CIoT network with beacon-aided RF energy harvesting under dissimilar fading environments

Abstract

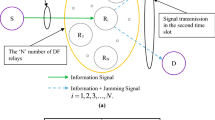
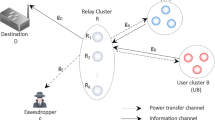
This paper presents a novel energy-efficient Radio Frequency (RF) Energy Harvesting (EH) scheme for secrecy performance in Internet of Things (IoT) network using cooperative communication approach under dissimilar faded channel environments. We have combined the concept of Destination Based Jamming (DBJ) with power beacon aided EH in our model. This paper provides three scenarios when the links undergo rayleigh fading, weibull fading, and nakagami-q (hoyt) fading where the effect of fading on the secrecy performance has been examined. Closed-form expressions for Secrecy Rate (SR) and System Energy Efficiency (SEE) are derived for the given scenarios. Analytical expressions have been well substantiated through extensive simulation results. The comparative analysis of system performance in terms of SR with respect to some important system parameters has been shown for various conventional protocols i.e., Decode-and-Forward (DF), Amplify-and-Forward (AF) and Hybrid-Decode-Amplify-Forward (HDAF). Furthermore, our scheme has been compared with benchmark techniques such as Cooperative Relaying (CR) and Cooperative Jamming (CJ) to quantify the performance improvement. Theoretical analysis and simulated results reveal the improvement of our proposed Signal-to-Noise (SNR) based hybrid decode-amplify forward Cooperative IoT (CIoT) scheme over existing relaying schemes which are further validated through statistical analysis. Results also depict the impact of eavesdropper position on secrecy performance at different channel mean power values. Finally, the SEE has been addressed and the impact of power splitting factor and transmit beacon power on the secrecy performance on the proposed model has also been analyzed with respect to change in fading parameters.

Appendices

Appendix

Proof of proposition 1

The Cumulative Distribution Function (CDF) of the S-R link is given as:

$$\begin{gathered} F_{{\gamma_{SR} }} (\gamma_{th} ) = P(\gamma_{SR} < \gamma_{th} ) = P\left( {\frac{{(1 - \beta )(\rho p_{S} )g_{SR} }}{{(1 - \beta )p_{D} g_{RD} + 1}} < \gamma_{th} } \right) \hfill \\ = P\left( {\frac{{(1 - \beta )\rho p_{S} X_{1} }}{{(1 - \beta )p_{D} X_{2} + 1}} < \gamma_{th} } \right) \hfill \\ = \int\limits_{0}^{\infty } {F_{{X_{1} }} \left( {\frac{{\gamma_{th} (p_{D} X_{2} + 1)}}{{p_{S} }}} \right)_{1} } f_{{X_{2} }} (x_{2} )dx_{2} \hfill \\ = \left[ {1 - \frac{1}{{\psi_{X} (\gamma_{th} + \psi_{X}^{ - 1} )}}\exp \left( { - \frac{{\gamma_{th} \lambda_{{X_{1} }} }}{{p_{S} }}} \right)} \right] \hfill \\ \end{gathered}$$

(42)

$$P(\gamma_{SR} \ge \gamma_{th} ) = \left[ {\frac{1}{{\psi_{X} (\gamma_{th} + \psi_{X}^{ - 1} )}}\exp \left( { - \frac{{\gamma_{th} \lambda_{{X_{1} }} }}{{p_{S} }}} \right)} \right]$$

(43)

where.

$\psi_{X} = \frac{{\rho_{D} }}{{\rho_{S} }}\frac{{m_{x} }}{{m_{y} }}$; $\rho_{S} = \frac{{P_{S} }}{{N_{O} }}$;$\rho_{D} = \frac{{P_{D} }}{{N_{O} }}$

$$\begin{gathered} C_{{\gamma_{RD} }}^{AF} = {\rm E}\{ \ln (1 + \gamma_{RD} )\} \hfill \\ = {\rm E}\left\{ {\ln \left( {1 + \frac{{\mu^{2} (1 - \beta )(\rho P_{S} )g_{sr} g_{rd} }}{{(\mu^{2} g_{rd} + 1)N_{o} }}} \right)} \right\} \hfill \\ = {\rm E}\left\{ {\ln (1 + \frac{{X_{AF} Y_{AF} }}{{Y_{AF} + 1}})} \right\} \hfill \\ \mathop \ge \limits^{(a)} \ln \left( {1 + \exp \left( {{\rm E}\left\{ {\ln (\frac{{X_{AF} Y_{AF} }}{{Y_{AF} + 1}})} \right\}} \right)} \right) \hfill \\ = \ln \left( {1 + \exp \left( {\overbrace {{{\rm E}\{ \ln [X_{AF} Y_{AF} ]\} }}^{{\varphi_{1} }} - \overbrace {{{\rm E}\{ \ln [Y_{AF} + 1]\} }}^{{\varphi_{2} }}} \right)} \right) \hfill \\ \end{gathered}$$

(44)

where $X_{AF} = \frac{{(1 - \beta )(\rho P_{S} )g_{SR} }}{{N_{O} }}$ and $Y_{AF} = \mu^{2} g_{RD}$ are exponential random variables with mean $\lambda_{{X_{AF} }} = \frac{{(1 - \beta )(\rho P_{S} )\Omega_{SR} }}{{N_{O} }}$ and $\lambda_{{Y_{AF} }} = \mu^{2} \Omega_{RD}$; Using Eq. (4.352.1) and Eq. (4.331.2) in Gradshteyn and Ryzhik (2007), $\varphi_{1}$ and $\varphi_{2}$ can be calculated, respectively as.

$$\varphi_{1} = - 2\Phi - \ln \left( {\frac{1}{{\lambda_{{X_{AF} }} \lambda_{{Y_{AF} }} }}} \right)\,\varphi_{2} = - \exp (\lambda_{{Y_{AF} }}^{ - 1} )Ei\left( { - \frac{1}{{\lambda_{{Y_{AF} }} }}} \right)$$

(45)

where $\Phi$ is the Euler’s constant [9.73].

Putting the value of $\varphi_{1}$ and $\varphi_{2}$ from (48) to (47) we have,

$$C_{{\gamma_{{\text{RD}}} }}^{AF} = \ln \left( {1 + \exp \left( { - 2\phi - \ln \left( {\frac{1}{{\lambda_{{X_{AF} }} \lambda_{{Y_{AF} }} }}} \right)} \right) + \exp (\lambda_{{Y_{AF} }}^{ - 1} )Ei\left( { - \frac{1}{{\lambda_{{Y_{AF} }} }}} \right)} \right)$$

(46)

where $Ei(.)$ is the exponential integral.

Capacity of EAV channel is given as:

$$C_{{\gamma_{{\text{RE}}} }}^{AF} = P\left( {\frac{{\mu^{2} (1 - \beta )(\rho P_{S} )g_{sr} g_{re} }}{{P_{D} g_{rd} }} < R_{th} } \right) = P\left( {\frac{{X_{2} g_{sr} g_{re} }}{{g_{rd} }} < R_{th} } \right)$$

$$= P\left( {g_{sr} < \frac{{R_{th} }}{{X_{2} }}\underbrace {{\frac{{g_{rd} }}{{g_{re} }}}}_{{Y_{2} }}} \right) = P\left( {g_{sr} < \frac{{R_{th} }}{{X_{2} }}Y_{2} } \right) = \int\limits_{0}^{\infty } {F_{{g_{sr} }} \left( {\frac{{R_{th} }}{{X_{2} }}y_{2} } \right)} .f_{{Y_{2} }} (y_{2} )dy_{2} = \int\limits_{0}^{\infty } {(1 - e^{{\frac{{R_{th} Y_{2} }}{{X_{2} g_{sr} }}}} )} .\frac{{\lambda_{re} \lambda_{rd} }}{{(\lambda_{rd} + y_{2} \lambda_{re} )^{2} }}dy_{2}$$

After some simplification and manipulation:

$$C_{{\gamma_{RE} }}^{AF} = 1 - \frac{{\lambda_{{RD_{AF} }} R_{th} }}{{X_{2} \lambda_{{RE_{AF} }} \lambda_{SR} }}\exp \left( {\frac{{\lambda_{{RD_{AF} }} R_{th} }}{{a_{1} \lambda_{{RE_{AF} }} \lambda_{SR} }}} \right)Ei\left( { - \frac{{\lambda_{{RD_{AF} }} R_{th} }}{{a_{1} \lambda_{{RE_{AF} }} \lambda_{SR} }}} \right) - \frac{{\lambda_{{RE_{AF} }} }}{{\lambda_{{RD_{AF} }} }}$$

(47)

The secrecy capacity of HDAF relay when operating in AF mode is given as:

$$\begin{gathered} C_{S}^{AF} = C_{RD}^{AF} - C_{RE}^{AF} = \ln \left( {1 + \exp \left( { - 2\phi - \ln \left( {\frac{1}{{\lambda_{{X_{AF} }} \lambda_{{Y_{AF} }} }}} \right)} \right) + \exp \left( {\lambda_{{Y_{AF} }}^{ - 1} } \right)Ei\left( {\frac{1}{{\lambda_{{Y_{AF} }} }}} \right)} \right) \hfill \\ - \left( {1 - \frac{{\lambda_{{RD_{AF} }} R_{th} }}{{X_{2} \lambda_{{RE_{AF} }} \lambda_{SR} }}\exp \left( {\frac{{\lambda_{{RD_{AF} }} R_{th} }}{{a_{1} \lambda_{{RE_{AF} }} \lambda_{SR} }}} \right)Ei\left( { - \frac{{\lambda_{RDAF} R_{th} }}{{a_{1} \lambda_{{RE_{AF} }} \lambda_{SR} }}} \right) - \frac{{\lambda_{{RE_{AF} }} }}{{\lambda_{{RD_{AF} }} }}} \right) \hfill \\ \end{gathered}$$

(48)

The secrecy capacity of HDAF relay when operating in DF mode is given as:

$$\begin{gathered} C_{{\gamma_{RD} }}^{DF} = {\rm E}\left\{ {\ln \left( {1 + \gamma_{RD} } \right)} \right\} \hfill \\ = {\rm E}\left\{ {\ln \left( {1 + \frac{{\eta \beta (1 - \beta )\rho P_{S} g_{sr} g_{rd} }}{{(\eta \beta g_{sr} + (1 - \beta ))N_{o} }}} \right)} \right\} \hfill \\ = {\rm E}\left\{ {\ln (1 + \frac{{X_{DF} Y_{DF} }}{{Y_{DF} + 1}})} \right\} \hfill \\ \mathop \ge \limits^{(a)} \ln \left( {1 + \exp \left( {{\rm E}\left\{ {\ln (\frac{{X_{DF} Y_{DF} }}{{Y_{DF} + 1}})} \right\}} \right)} \right) \hfill \\ = \ln \left( {1 + \exp \left( {\overbrace {{{\rm E}\{ \ln [X_{DF} Y_{DF} ]\} }}^{{\varphi_{1} }} - \overbrace {{{\rm E}\{ \ln [Y_{DF} + 1]\} }}^{{\varphi_{2} }}} \right)} \right) \hfill \\ \end{gathered}$$

(49)

where $X_{DF} = \frac{{(1 - \beta )(\rho P_{S} )g_{SR} }}{{N_{O} }}$ and $Y_{DF} = \frac{{\eta \beta g_{rd} }}{(1 - \beta )}$ are exponential random variables with means $\lambda_{{X_{DF} }} = \frac{{(1 - \beta )(\rho P_{S} )\Omega_{SR} }}{{N_{O} }}$ and $\lambda_{{Y_{DF} }} = \frac{{\eta \beta \Omega_{rd} }}{(1 - \beta )}$.

Capacity of EAV channel is given as:

$$C_{{\gamma_{{\text{RE}}} }}^{DF} = P\left( {\frac{{(1 - \beta )(\rho P_{S} )g_{sr} g_{re} }}{{P_{D} g_{rd} }} < R_{th} } \right) = P\left( {\frac{{X_{3} g_{sr} g_{re} }}{{g_{rd} }} < R_{th} } \right)$$

$$= P\left( {g_{sr} < \frac{{R_{th} }}{{X_{3} }}\underbrace {{\frac{{g_{rd} }}{{g_{re} }}}}_{{Y_{3} }}} \right) = P\left( {g_{sr} < \frac{{R_{th} }}{{X_{3} }}Y_{3} } \right) = \int\limits_{0}^{\infty } {F_{{g_{sr} }} \left( {\frac{{R_{th} }}{{X_{3} }}y_{3} } \right)} .f_{{Y_{3} }} (y_{3} )dy_{3} = \int\limits_{0}^{\infty } {(1 - e^{{\frac{{R_{th} Y_{3} }}{{X_{3} g_{sr} }}}} )} .\frac{{\lambda_{re} \lambda_{rd} }}{{(\lambda_{rd} + y_{3} \lambda_{re} )^{2} }}dy_{3}$$

After some simplification and manipulation:

$$C_{{\gamma_{{\text{RE}}} }}^{DF} = \left( {1 - \frac{{\lambda_{{RD_{DF} }} R_{th} }}{{X_{3} \lambda_{{RE_{DF} }} \lambda_{SR} }}\exp \left( {\frac{{\lambda_{{RD_{DF} }} R_{th} }}{{a_{1} \lambda_{{RE_{DF} }} \lambda_{SR} }}} \right)Ei\left( { - \frac{{\lambda_{{RD_{DF} }} R_{th} }}{{a_{1} \lambda_{{RE_{DF} }} \lambda_{SR} }}} \right) - \frac{{\lambda_{{RE_{DF} }} }}{{\lambda_{{RD_{DF} }} }}} \right)$$

(50)

Similarly, the secrecy capacity of HDAF relay when operating in DF mode is given as:

$$\begin{gathered} C_{S}^{DF} = C_{{\gamma_{RD} }}^{DF} - C_{{\gamma_{RE} }}^{DF} = \ln \left( {1 + \exp \left( { - 2\phi - \ln \left( {\frac{1}{{\lambda_{{X_{DF} }} \lambda_{{Y_{DF} }} }}} \right)} \right) + \exp (\lambda_{{Y_{DF} }}^{ - 1} )Ei\left( { - \frac{1}{{\lambda_{{Y_{DF} }} }}} \right)} \right) \hfill \\ - \left( {1 - \frac{{\lambda_{{RD_{DF} }} R_{th} }}{{X_{3} \lambda_{{RE_{DF} }} \lambda_{SR} }}\exp \left( {\frac{{\lambda_{{RD_{DF} }} R_{th} }}{{a_{1} \lambda_{{RE_{DF} }} \lambda_{SR} }}} \right)Ei\left( { - \frac{{\lambda_{{RD_{DF} }} R_{th} }}{{a_{1} \lambda_{{RE_{DF} }} \lambda_{SR} }}} \right) - \frac{{\lambda_{{RE_{DF} }} }}{{\lambda_{{RD_{DF} }} }}} \right) \hfill \\ \end{gathered}$$

(51)

Thus substituting Eq. (48) and Eq. (51) in Eq. (31) we obtain Eq. (36). Putting Eq. (36) in Eq. (41) gives us the closed form equation for SEE.

Proof of proposition 2.

The pdf of main and wiretap links for weibull fading takes the following form:

$$f_{{\gamma_{RD} }} (\gamma_{RD} ) = \frac{{v_{m} }}{2}\left( {\frac{1}{{u_{o} }}\Gamma (1 + \frac{2}{{v_{m} }})} \right)^{{\frac{{v_{m} }}{2}}} u^{{v_{m} /2 - 1}} \times \exp \left\{ { - \left[ {\frac{u}{{u_{o} }}\Gamma (1 + \frac{2}{{v_{m} }})} \right]} \right\}^{{\frac{{v_{m} }}{2}}}$$

(52)

$$f_{{\gamma_{{\text{RE}}} }} (\gamma_{RE} ) = \frac{{v_{e} }}{2}\left( {\frac{1}{{w_{o} }}\Gamma (1 + \frac{2}{{v_{e} }})} \right)^{{\frac{{v_{e} }}{2}}} w^{{v_{e} /2 - 1}} \times \exp \left\{ { - \left[ {\frac{w}{{w_{o} }}\Gamma (1 + \frac{2}{{v_{e} }})} \right]} \right\}^{{\frac{{v_{e} }}{2}}}$$

(53)

where $v_{m}$ is the fading factor of the main channel and $\Gamma (.)$ is the gamma function, defined as:

$$\Gamma (x) = \int\limits_{0}^{\infty } {t^{x - 1} \exp ( - t)dt}$$

$v_{e}$ is the fading factor of the EAV channel.

Corresponding to (55) and (56), the CDF takes the following form:

$$F_{{\gamma_{RD} }} (\gamma_{RD} ) = 1 - \exp \left( { - \left[ {\frac{{\gamma_{RD} }}{{\overline{\gamma }_{RD} }}\Gamma \left( {1 + \frac{2}{{v_{m} }}} \right)} \right]^{{v_{m} /2}} } \right)$$

(54)

$$F_{{\gamma_{RE} }} (\gamma_{RE} ) = 1 - \exp \left( { - \left[ {\frac{{\gamma_{RE} }}{{\overline{\gamma }_{RE} }}\Gamma \left( {1 + \frac{2}{{v_{e} }}} \right)} \right]^{{v_{e} /2}} } \right)$$

(55)

The SR of the channel is defined as follows:

$$C_{{S_{WEIBULL} }}^{AF} = \int\limits_{0}^{\infty } {\int\limits_{{\gamma_{{RE_{AF} }} }}^{\infty } {\log_{2} \left( {\frac{{1 + \gamma_{{RD_{AF} }} }}{{1 + \gamma_{{RE_{AF} }} }}} \right)} } f_{{\gamma_{{RD_{AF} }} }} (\gamma_{{RD_{AF} }} )f_{{\gamma_{{RE_{AF} }} }} (\gamma_{{RE_{AF} }} )d\gamma_{{RD_{AF} }} d\gamma_{{RE_{AF} }}$$

(56)

Substituting (20), (22), (55) and (56) into (59), we have:

$$\begin{gathered} C_{{S_{WEIBULL} }}^{AF} = \frac{{v_{m} v_{e} }}{4}\left[ {\frac{1}{{\overline{\gamma }_{{RD_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{m} }}} \right)} \right]^{{v_{m} /2}} \left[ {\frac{1}{{\overline{\gamma }_{{RE_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{m} }}} \right)} \right]^{{v_{e} /2}} \times \int\limits_{0}^{\infty } {\int\limits_{{\gamma_{{RE_{AF} }} }}^{\infty } {\log_{2} \left( {\frac{{1 + \gamma_{{RD_{AF} }} }}{{1 + \gamma_{{RE_{AF} }} }}} \right)} } \gamma_{{RD_{AF} }}^{{(v_{m} /2) - 1}} \exp \left( { - \left[ {\frac{{\gamma_{{RD_{AF} }} }}{{\overline{\gamma }_{{RD_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{m} }}} \right)} \right]^{{\frac{{v_{m} }}{2}}} } \right) \times \hfill \\ \gamma_{{RE_{AF} }}^{{(v_{e} /2) - 1}} \exp \left( { - \left[ {\frac{{\gamma_{{RE_{AF} }} }}{{\overline{\gamma }_{{RE_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{e} }}} \right)} \right]^{{\frac{{v_{e} }}{2}}} } \right)d\gamma_{{RD_{AF} }} d\gamma_{{RE_{AF} }} \hfill \\ \end{gathered}$$

(57)

After converting to single integral form, we have,

$$C_{{S_{WEIBULL} }}^{AF} = \frac{2}{{v_{m} }}\log_{2} \left( {1 + \frac{{\gamma_{{RD_{AF} }}^{{v_{m} /2}} }}{{\gamma_{{RE_{AF} }}^{{v_{e} /2}} }}} \right) - \int\limits_{0}^{1} {\log_{2} \left( {1 + \frac{{2\Gamma (2/v_{m} )\{ [1 + (\gamma_{{RD_{AF} }}^{{}} /\gamma_{{RE_{AF} }}^{{}} )^{{v_{m} /2}} ]^{{2/v_{m} }} - 1\} }}{{2\Gamma (2/v_{m} ) + a\gamma_{{RD_{AF} }}^{{}} ( - \ln t)^{{2/v_{m} }} }}} \right)} dt$$

(58)

$$C_{{S_{WEIBULL} }}^{AF} = \int\limits_{1}^{\infty } {\log_{2} (z)f_{Z} (z)dz}$$

(59)

According to probability theory, we have:

$$f_{Z} (z) = \int\limits_{0}^{\infty } {\gamma_{RE} f_{{\gamma_{RD} }} (\gamma_{RE} z)f_{{\gamma_{RE} }} (\gamma_{RE} )} d\gamma_{RE}$$

Accordingly, the CDF is formulated as:

$$F_{Z} (z) = \int\limits_{0}^{z} {f_{Z} (t)dt = \int\limits_{0}^{z} {\int\limits_{0}^{\infty } {\gamma_{RE} f_{{\gamma_{RD} }} (\gamma_{RE} t)f_{{\gamma_{RE} }} (\gamma_{RE} )d\gamma_{RE} } } }$$

$$= \int\limits_{0}^{\infty } {f_{{\gamma_{RE} }} (\gamma_{RE} )\left[ {\int\limits_{0}^{{\gamma_{RE} z}} {f_{{\gamma_{RD} }} (y)dy} } \right]} d\gamma_{RE} = \int\limits_{0}^{\infty } {f_{{\gamma_{RE} }} (\gamma_{RE} )F_{{\gamma_{RD} }} (\gamma_{RE} z)d\gamma_{RE} }$$

(60)

With several transformations, we obtain:

$$F_{Z} (z) = \frac{{v_{e} }}{2}(H_{1} - H_{2} )$$

(61)

$$H_{1} = \int\limits_{0}^{\infty } {\gamma_{{RE_{AF} }}^{{(v_{e} /2) - 1}} \left[ {\frac{1}{{\overline{\gamma }_{{RE_{AF} }}^{{}} }}\Gamma \left( {1 + \frac{2}{{v_{e} }}} \right)} \right]}^{{\frac{{v_{e} }}{2}}} \times \exp \left( { - \left[ {\frac{{\gamma_{{RE_{AF} }} }}{{\overline{\gamma }_{{RE_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{e} }}} \right)} \right]^{{^{{\frac{{v_{e} }}{2}}} }} } \right)d\gamma_{{RE_{AF} }} \approx \frac{2}{{v_{e} }}$$

$$H_{2} = \int\limits_{0}^{\infty } {\gamma_{{RE_{AF} }}^{{(v_{e} /2) - 1}} } \exp \left( { - \left[ {\frac{{\gamma_{{RE_{AF} }} z}}{{\overline{\gamma }_{{RE_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{e} }}} \right)} \right]^{{^{{\frac{{v_{m} }}{2}}} }} } \right) \times \left[ {\frac{1}{{\overline{\gamma }_{{RE_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{e} }}} \right)} \right]^{{\frac{{v_{e} }}{2}}} \exp \left( { - \left[ {\frac{{\gamma_{{RE_{AF} }} }}{{\overline{\gamma }_{{RE_{AF} }} }}\Gamma \left( {1 + \frac{2}{{v_{e} }}} \right)} \right]^{{^{{\frac{{v_{m} }}{2}}} }} } \right)d\gamma_{{RE_{AF} }}$$

$$= \frac{2}{{v_{e} }}\int\limits_{0}^{\infty } {\exp \left( { - t - \left[ {\frac{{v_{e} \Gamma (2/v_{m} )z}}{{v_{m} \Gamma (2/v_{e} )r}}} \right]^{{\frac{{v_{m} }}{2}}} t^{{\frac{{v_{m} }}{{v_{e} }}}} } \right)} dt$$

Thus

$$F_{Z} (z) = 1 - \int\limits_{0}^{\infty } {\exp \left[ { - t - \left( \frac{z}{r} \right)^{{\frac{{v_{m} }}{2}}} t} \right]dt = 1 - \frac{1}{{1 + (z/r)^{{v_{m} /2}} }}}$$

(62)

Thus, secrecy capacity of HDAF relay when operating in AF mode is given as:

$$C_{{S_{WEIBULL} }}^{AF} = \int\limits_{1}^{\infty } {\log_{2} (z)f_{Z} (z)dz} = \frac{1}{\ln 2}\int\limits_{1}^{\infty } {\frac{dz}{{z[1 + (z/r)^{{v_{m} /2}} ]}}} \approx \frac{2}{{v_{m} }}\log_{2} (1 + r^{{v_{m} /2}} )$$

(63)

Similarly, the secrecy capacity of HDAF relay when operating in DF mode is given as:

$$C_{{S_{WEIBULL} }}^{DF} = \frac{2}{{v_{m} }}\log_{2} (1 + r^{{v_{m} /2}} )$$

(64)

Thus substituting Eq. (63) and Eq. (64) in Eq. (31) we obtain Eq. (37) and thereafter the closed form equation for SEE.

Proof of proposition 3.

The pdf of S-R link for hoyt fading takes the following form:

$$f_{{\gamma_{SR} }} (\gamma_{th} ) = \frac{{(1 + q_{m}^{2} )}}{{2q_{m} \overline{\gamma }_{RD} }}\exp \left( { - \frac{{(1 + q_{m}^{2} )^{2} \gamma_{th} }}{{4q_{m}^{2} \overline{\gamma }_{RD} }}} \right)I_{O} \left( {\frac{{1 - q_{m}^{4} }}{{4q_{m}^{2} \overline{\gamma }_{RD} }}\gamma_{th} } \right)$$

(65)

where $I_{O} (.)$ is the modified bessel function of the first kind and zero order, $\overline{\gamma }_{RD} = {\rm E}\{ \gamma_{RD} \}$ and $q \in [0,1]$

$$F_{{\gamma_{SR} }} (\gamma_{th} ) = Q\left( {\alpha (q_{1} )\sqrt {\frac{{\gamma_{th} }}{{\overline{\gamma }_{1} }}} ,\beta (q_{1} )\sqrt {\frac{{\gamma_{th} }}{{\overline{\gamma }_{1} }}} } \right) - Q\left( {\beta (q_{1} )\sqrt {\frac{{\gamma_{th} }}{{\overline{\gamma }_{1} }}} ,\alpha (q_{1} )\sqrt {\frac{{\gamma_{th} }}{{\overline{\gamma }_{1} }}} } \right)$$

(66)

$$\left\{ \begin{gathered} \alpha (q) = \frac{{\sqrt {1 - q^{4} } }}{2q}\sqrt {\frac{1 + q}{{1 - q}}} \hfill \\ \beta (q) = \frac{{\sqrt {1 - q^{4} } }}{2q}\sqrt {\frac{1 - q}{{1 + q}}} \hfill \\ \end{gathered} \right.$$

For rayleigh faded channel, the capacity per unit bandwidth using optimum rate adaptation (ORA) policy is calculated as:

$$P(C_{S} > R_{S} ) = \frac{{\overline{\gamma }_{RD} }}{{\overline{\gamma }_{RD} + 2^{{R_{S} }} \overline{\gamma }_{RE} }}\exp \left( { - \frac{{2^{{R_{S} }} - 1}}{{\overline{\gamma }_{RD} }}} \right)$$

Now, for a hoyt faded channel, the capacity per unit bandwidth is calculated as:

$$C_{S}^{AF} = \frac{{\log_{2} (e)}}{\pi }\int\limits_{0}^{\pi } {e^{1/\gamma (\theta ,q)} E_{1} \left( {\frac{1}{\gamma (\theta ,q)}} \right)d\theta }$$

(67)

where $\gamma (\theta ,q) \triangleq \overline{\gamma }(1 - \frac{{1 - q^{2} }}{{1 + q^{2} }}\cos \theta )$

$$C_{{S_{HOYT} }}^{AF} = \frac{1}{{\pi^{2} }}\int\limits_{0}^{\pi } {\int\limits_{0}^{\pi } {\exp \left( { - \frac{{2^{{R_{th} }} - 1}}{{\overline{\gamma }_{{RD_{AF} }} (1 - \in_{{RD_{AF} }} \cos \theta_{{RD_{AF} }} )}}} \right)} \times \frac{{\overline{\gamma }_{{RD_{AF} }} (1 - \in_{{RD_{AF} }} \cos \theta_{{RD_{AF} }} )}}{{\overline{\gamma }_{RD} (1 - \in_{{RD_{AF} }} \cos \theta_{{RD_{AF} }} ) + 2^{{R_{S} }} \overline{\gamma }_{RE} (1 - \in_{RE} \cos \theta_{{RE_{AF} }} )}}d\theta_{{RE_{AF} }} } d\theta_{{RD_{AF} }}$$

(68)

where $q_{m}$ and $q_{e}$ represent the hoyt shape parameters for the desired and EAV links. Eccentricities associated with both hoyt distributions as $\in_{RD} = \frac{{1 - q_{m}^{2} }}{{1 + q_{m}^{2} }}$ and $\in_{RE} = \frac{{1 - q_{e}^{2} }}{{1 + q_{e}^{2} }}$

$$C_{{S_{HOYT} }}^{AF} = \frac{1}{\pi }\int\limits_{0}^{\pi } {\exp \left( { - \frac{{2^{{R_{th} }} - 1}}{{\overline{\gamma }_{{RD_{AF} }} (\theta )}}} \right)} \times \frac{{\overline{\gamma }_{{RD_{AF} }} (\theta )}}{{\overline{\gamma }_{{RD_{AF} }} (\theta ) + 2^{{R_{th} }} \overline{\gamma }_{{RE_{AF} }} }}\frac{1}{{\sqrt {1 - \left( {\frac{{q_{e} 2^{{R_{S} }} \overline{\gamma }_{{{\text{RE}}_{AF} }} }}{{\overline{\gamma }_{{{\text{RD}}_{AF} }} (\theta ) + 2^{{R_{S} }} \overline{\gamma }_{{{\text{RE}}_{AF} }} }}} \right)^{2} } }}d\theta$$

(69)

Thus, secrecy capacity of HDAF relay when operating in AF mode is given as:

$$C_{{S_{HOYT} }}^{AF} = \frac{{\overline{\gamma }_{{RD_{AF} }} }}{{\overline{\gamma }_{{RD_{AF} }} + \overline{\gamma }_{{RE_{AF} }} }}\frac{1}{{\sqrt {1 - \left( {\frac{{q_{e} \overline{\gamma }_{{{\text{RE}}_{AF} }} }}{{\overline{\gamma }_{{{\text{RD}}_{AF} }} + \overline{\gamma }_{{{\text{RE}}_{AF} }} }}} \right)^{2} } }}$$

(70)

Similarly, the secrecy capacity of HDAF relay when operating in DF mode is given as:

$$C_{{S_{HOYT} }}^{DF} = \frac{{\overline{\gamma }_{{RD_{DF} }} }}{{\overline{\gamma }_{{RD_{DF} }} + \overline{\gamma }_{{RE_{DF} }} }}\frac{1}{{\sqrt {1 - \left( {\frac{{q_{e} \overline{\gamma }_{{{\text{RE}}_{DF} }} }}{{\overline{\gamma }_{{{\text{RD}}_{DF} }} + \overline{\gamma }_{{{\text{RE}}_{DF} }} }}} \right)^{2} } }}$$

(71)

Thus substituting Eq. (70) and Eq. (71) in Eq. (31) we obtain Eq. (38) followed by the closed form equation for SEE.