Abstract
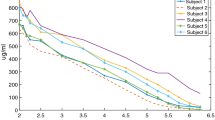
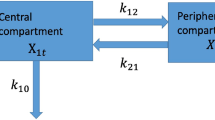
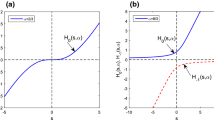
Pharmacokinetics studies the time course of drug concentration in body compartments, and one of the commonly used methods of administration is extravascular administration. Undoubtedly, biological systems are subject to various internal and external noises that change over time. To rationally deal with these dynamic noises, this paper deduces a pharmacokinetic model for extravascular administration using uncertain differential equations for the first time. Some essential pharmacokinetic indexes such as drug concentration, area under the curve, and maximum drug concentration are investigated. Based on the three basic principles of reliability science, i.e., margin-based reliable principle, eternal degradation principle and uncertainty principle, the belief reliability for drug is investigated. The minimum cover estimation for the set of unknown parameters in this uncertain pharmacokinetic model is provided. Numerical example and a real data analysis illustrate our method in detail. Finally, the paradox of a stochastic pharmacokinetic model is presented.









Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Chen X, Liu B (2010) Existence and uniqueness theorem for uncertain differential equations. Fuzzy Optim Decis Mak 9:69–81
Ditlevsen S, Gaetano A (2005) Stochastic vs. deterministic uptake of dodecanedioic acid by isolated rat livers. Bull Math Biol 67:547–561
Ferrante L, Bompader S, Leone L (2003) A stochastic compartmental model with long lasting infusion. Biometr J 45:182–194
Gibaldi M, Perrier D (2007) Pharmacokinetics, 2nd edn. Informa Healthcare Inc, London
Kang R (2021) Belief reliability theory and methodology. Springer, Singapore
Kiureghian A, Ditlevsen O (2009) Aleatory or epistemic? Does it matter? Struct Saf 31:105–112
Li Z, Sheng Y, Teng Z, Miao H (2017) An uncertain differential equation for SIS epidemic model. J Intell Fuzzy Syst 33:2317–2327
Lio W, Liu B (2020) Initial value estimation of uncertain differential equations and zero-day of COVID-19 spread in China. Fuzzy Optim Decis Mak 20:177–188
Liu B (2013) Toward uncertain finance theory. J Uncertain Anal Appl 1:Article 1
Liu B (2007) Uncertainty theory, 2nd edn. Springer, Berlin
Liu B (2008) Fuzzy process, hybrid process and uncertain process. J Uncertain Syst 2:3–16
Liu B (2009) Some research problems in uncertainty theory. J Uncertain Syst 3:3–10
Liu Y (2012) Analytic method for solving uncertain differential equations. J Uncertain Syst 6:243–248
Liu B (2015) Uncertainty theory, 4th edn. Springer, Berlin
Liu Z (2021) Generalized moment estimation for uncertain differential equations. Appl Math Comput 392:125724
Liu Z, Yang Y (2021) Pharmacokinetic model based on multifactor uncertain differential equation. Appl Math Comput 392:125722
Liu Z, Yang Y (2021) Uncertain pharmacokinetics models based on uncertain differential equations. Appl Math Comput 404:126118
Liu Z, Yang X (2021) A linear uncertain pharmacokinetic model driven by Liu process. Appl Math Model 89:1881–1899
Liu Z, Yang Y (2021) Selection of uncertain differential equations using cross validation. Chaos Soliton Fract 148:111049
Ramanathan M (1999) An application of Ito’s lemma in population pharmacokinetics and pharmacodynamics. Pharm Res Dordr 16:584–586
Stephanou A, Fanchon E, Innominato P et al (2018) Systems biology, systems medicine, systems pharmacology: the what and the why. Acta Biotheor 66:345–365
Teorell T (1937) Kinetics of distribution of substances administered to the body I. The extravascular modes of administration. Arch Int Pharmacodyn Ther 57:205–225
Teorell T (1937) Kinetics of distribution of substances administered to the body II. The intravascular modes of administration. Arch Int Pharmacodyn Ther 57:226–240
Wagner J (1973) A modern view of pharmaeokinetics. J Pharmacokinet Biopharm 1:363–401
Yakatan G, Smith R, Frome E et al (1977) Pharmacokinetics of orally administered hydroflumethiazide in man. J Clin Pharmacol 17:37–47
Yang X, Ralescu D (2015) Adams method for solving uncertain differential equations. Appl Math Comput 270:993–1003
Yang X, Yao K (2017) Uncertain partial differential equation with application to heat conduction. Fuzzy Optim Decis Mak 16:379–403
Yang X, Liu Y, Park G (2020) Parameter estimation of uncertain differential equation with application to financial market. Chaos Soliton Fract 139:110026
Yang X, Shen Y (2015) Runge–Kutta method for solving uncertain differential equations. J Uncertain Anal Appl 3:Article 17
Yao K (2013) A type of nonlinear uncertain differential equations with analytic solution. J Uncertain Anal Appl 1:Article 8
Yao K (2013) Extreme values and integral of solution of uncertain differential equation. J Uncertain Anal Appl 1:2
Yao K, Chen X (2013) A numerical method for solving uncertain differential equations. J Intell Fuzzy Syst 25:825–832
Yao K, Liu B (2020) Parameter estimation in uncertain differential equations. Fuzzy Optim Decis Mak 19:1–12
Yao K, Gao J, Gao Y (2013) Some stability theorems of uncertain differential equation. Fuzzy Optim Decis Mak 12:3–13
Yao K, Ke H, Sheng Y (2015) Stability in mean for uncertain differential equation. Fuzzy Optim Decis Mak 14:365–379
Zhang Z, Yang X (2020) Uncertain population model. Soft Comput 24:2417–2423
Acknowledgements
This work was supported by National Natural Science Foundation of China (No. 62073009) and the Funding of Science and Technology on Reliability and Environmental Engineering Laboratory (No. 6142004210401)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Liu, Z., Kang, R. Pharmacokinetic model for extravascular administration based on uncertain differential equation. J Ambient Intell Human Comput 14, 13887–13899 (2023). https://doi.org/10.1007/s12652-022-04100-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12652-022-04100-8