Skip to main content
Springer Nature Link
Log in

An uncertain multi-objective programming model for machine scheduling problem

  • Original Article
  • Published:
International Journal of Machine Learning and Cybernetics Aims and scope Submit manuscript

Abstract

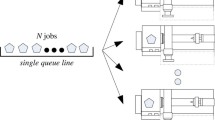
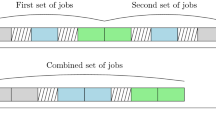
This paper discusses a parallel machine scheduling problem in which the processing times of jobs and the release dates are independent uncertain variables with known uncertainty distributions. An uncertain programming model with multiple objectives is obtained, whose first objective is to minimize the maximum completion time or makespan, and second objective is to minimize the maximum tardiness time. A genetic algorithm is employed to solve the proposed uncertain machine scheduling model, and its efficiency is illustrated by some numerical experiments.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Ahmadizar F, Ghazanfari M, Ghomi SM (2010) Group shops scheduling with makespan criterion subject to random release dates and processing times. Comput Oper Res 37:152–162

    Article  MathSciNet  MATH  Google Scholar 

  2. Baker KR, Scudder GD (1990) Sequencing with earliness and tardiness penalties: a review. Oper Res 38:22–36

    Article  MathSciNet  MATH  Google Scholar 

  3. Banerjee BP (1965) Single facility sequencing with random execution times. Oper Res 13(3):358–364

    Article  MathSciNet  Google Scholar 

  4. Chen XW (2011) American option pricing formula for uncertain financial market. Int J Oper Res 8(2):32–37

    MathSciNet  Google Scholar 

  5. Gharehgozli AH, Moghaddam TR, Zaerpour N (2009) A fuzzy-mixed-integer goal programming model for a parallel-machine scheduling problem with sequence-dependent setup times and release dates. Robot Comput Integr Manuf 25:853–859

    Article  Google Scholar 

  6. Graham RL, Lawler EL, Lenstra JK, Rinnooy Kan AHG (1979) Optimization and approximation in deterministic sequencing and scheduling: a survey. Ann Discrete Math 5:287–326

    Article  MathSciNet  MATH  Google Scholar 

  7. Hodegson TJ (1977) A note on single machine sequencing with random processing times. Manag Sci 23(10):1144–1146

    Article  MathSciNet  Google Scholar 

  8. Ishibuchi H, Yamamoto N, Murata T, Tanaka H (1994) Genetic algorithms and neighborhood search algorithms for fuzzy flowshop scheduling problems. Fuzzy Sets Syst 67:81–100

    Article  MathSciNet  Google Scholar 

  9. Johnson SM (1954) Optimal two and three-stage production schedules with setup times included. Nav Res Logist Q 1:61–68

    Article  MATH  Google Scholar 

  10. Kahneman D, Tversky A (1979) Prospect theory: an analysis of decision under risk. Econometrica 47(2):263–292

    Article  MATH  Google Scholar 

  11. Lam K, Xing WX (1997) New trends in parallel machine scheduling. Int J Oper Prod Manag 17(3):326–338

    Article  Google Scholar 

  12. Lenstra JK, Rinnooy Kan AHG, Brucker P (1977) Complexity of machine scheduling problems. Ann Discrete Math 1:343–362

    Article  MathSciNet  MATH  Google Scholar 

  13. Liu B (2007) Uncertainty theory, 2nd edn. Springer-Verlag, Berlin

    MATH  Google Scholar 

  14. Liu B (2009) Theory and practice of uncertain programming, 2nd edn. Springer-Verlag, Berlin

    Book  MATH  Google Scholar 

  15. Liu B (2009) Some research problems in uncertainty theory. J Uncertain Syst 3(1):3–10

    MathSciNet  Google Scholar 

  16. Liu B (2010) Uncertainty theory: a branch of mathematics for modeling human uncertainty. Springer-Verlag, Berlin

    Book  Google Scholar 

  17. Liu B (2015) Uncertainty theory, 5th edn. Springer-Verlag, Berlin

    MATH  Google Scholar 

  18. Liu B, Chen X (2015) Uncertain multiobjective programming and uncertain goal programming. J Uncertain Anal Appl 3:10. doi:10.1186/s40467-015-0036-6

    Article  Google Scholar 

  19. Liu B, Yao K (2015) Uncertain multilevel programming: algorithm and applications. Comput Ind Eng 89:235–240

    Article  Google Scholar 

  20. Li RY, Liu G An uncertain goal programming model for machine scheduling problem. J Intell Manuf. doi:10.1007/s10845-014-0982-8

  21. Liu YH, Ha MH (2010) Expected value of function of uncertain variables. J Uncertain Syst 4(3):181–186

    Google Scholar 

  22. Mokotoff E (2001) Parallel machine scheduling problems: a survey. Asia Pac J Oper Res 18:193–242

    MathSciNet  MATH  Google Scholar 

  23. Naughton MC (1959) Scheduling with deadlines and loss functions. Manag Sci 6(1):1–12

    Article  MathSciNet  Google Scholar 

  24. Peng J, Liu B (2004) Parallel machine scheduling models with fuzzy processing times. Inf Sci 166:49–66

    Article  MathSciNet  MATH  Google Scholar 

  25. Petrovic S, Fayad C (2004) A fuzzy shifting bottleneck hybridised with genetic algorithm for real-world job shop scheduling. In: Proceedings of Mini-Euro Conference, managing uncertainty in decision support models, Coimbra, Portugal, pp 1–6

  26. Pinedo M (1983) Stochastic scheduling with release dates and due dates. Oper Res 31:559–572

    Article  MATH  Google Scholar 

  27. Pinedo M (2008) Scheduling: theory, algorithms and systems. Springer, New York

    MATH  Google Scholar 

  28. Prade H (1979) Using fuzzy set theory in a scheduling problem: a case study. Fuzzy Sets Syst 2:153–165

    Article  MATH  Google Scholar 

  29. Seo DK, Klein CM, Jang W (2005) Single machine stochastic scheduling to minimize the expected number of tardy jobs using mathematical programming models. Comput Ind Eng 48:153–161

    Article  Google Scholar 

  30. Wang X, Ning YF, Moughal TA, Chen XM (2015) Adams–Simpson method for solving uncertain differential equation. Appl Math Comput 271:209–219

    MathSciNet  Google Scholar 

  31. Wu XL, Lan YF, Liu HT (2014) Optimal revenue-sharing contract based on forecasting effort for uncertain agency problem. Int J Mach Learn Cybern 5(6):971–979

    Article  Google Scholar 

  32. Xing LN, Chen YW, Yang KW (2009) An efficient search method for multi-objective flexible job shop scheduling problems. J Intell Manuf 20:283–293

    Article  Google Scholar 

  33. Yang K, Zhao RQ, Lan YF (2014) The impact of risk attitude in new product development under dual information asymmetry. Comput Ind Eng 76(10):122–137

    Article  Google Scholar 

  34. Yao K (2015) Uncertain differential equation with jumps. Soft Comput 19(7):2063–2069

    Article  MATH  Google Scholar 

  35. Zhang XF, Meng GW (2013) Expected-variance-entropy model for uncertain parallel machine scheduling. Inf Int Interdiscip J 16(2(A)):903–908

    Google Scholar 

Download references

Acknowledgments

This work is supported by Natural Science Foundation of Shandong Province (ZR2014GL002).

Author information

Authors and Affiliations

  1. School of Information Engineering, Shandong Youth University of Political Science, Jinan, 250103, China

    Yufu Ning, Xiumei Chen, Zhiyong Wang & Xiangying Li

  2. Key Laboratory of Information Security and Intelligent Control in Universities of Shandong, Jinan, 250103, China

    Yufu Ning, Xiumei Chen, Zhiyong Wang & Xiangying Li

Authors
  1. Yufu Ning
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Xiumei Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Zhiyong Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Xiangying Li
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yufu Ning.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ning, Y., Chen, X., Wang, Z. et al. An uncertain multi-objective programming model for machine scheduling problem. Int. J. Mach. Learn. & Cyber. 8, 1493–1500 (2017). https://doi.org/10.1007/s13042-016-0522-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13042-016-0522-2

Keywords